No. E.g., (identifying linear operators with matrices in a standard manner) let $$a=\left( \begin{array}{cc} 1 & 0 \\ 0 & 0 \\ \end{array} \right),\quad b=\frac12 \left( \begin{array}{cc} 1 & 1 \\ 1 & 1 \\ \end{array} \right). $$ Then (by straightforward but somewhat tedious calculations) for all $p\in(0,1)$ $$d(p):=\det(a^p+b^p-(a+b)^p) \\ =2^{-p-3/2} \\ \times\left(\sqrt{2} \left(2^p+2\right)-2 \left(\sqrt{2}-1\right) \left(2+\sqrt{2}\right)^p-2 \left(1+\sqrt{2}\right) \left(2-\sqrt{2}\right)^p\right)<0 \tag{1}\label{1},$$ and hence $(a+b)^p\not\le a^p+b^p$.
To prove the inequality in \eqref{1}, let
$$d_0(p):=d(p)2^{p+3/2},\quad
d_1(p):=\frac{d_0'(p)}{(2+\sqrt2)^p},\\
d_2(p):=d_1'(p)(1+1/\sqrt2)^p.
$$
Then
$$d_2'(p)=\left(1+\sqrt{2}\right) 2^{1-p} \left(2-\sqrt{2}\right)^p \ln\left(3-2
\sqrt{2}\right) \ln\left(2-\sqrt{2}\right) \ln\left(2+\sqrt{2}\right)>0,$$
$d_2$ is increasing (on $(0,1)$), $d_2(1)=\sqrt{2} \ln\left(2-\sqrt{2}\right) \ln\left(6+4 \sqrt{2}\right)<0$, $d_2<0$, $d_1$ is decreasing, $d_1(1)=0$, $d_1>0$, $d_0$ is increasing, $d_0(1)=0$, $d_0<0$, $d<0$. $\quad\Box$
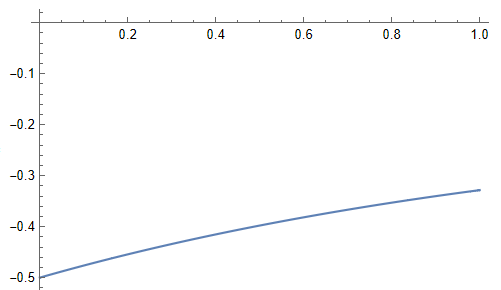
Here is the graph $\{(p,d(p)(1-p)^{-2})\colon0<p<1\}$: