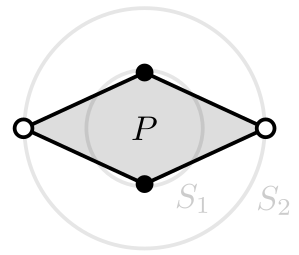
After some simplifications, I arrived to the following general fact (it can be generalized even further).
Paint the vertices in black and white according to the bipartite structure. We prove that, if all black vertices lie on a sphere, then one of the white vertices is (non-strictly) outside the corresponding ball, provided that there are at least as many black vertices as white ones.
Suppose the contrary. Let $W$ and $B$ be the convex hulls of white and black vertices, respectively. By bipartiteness, each edge of $B$ is crossed by $W$ (and vice versa). Moreover, there exist edges of $W$ lying on the boundary of $P$.
Take any point $a$ strictly inside $P$ and project all white vertices from $a$ to the sphere to obtain green points; let $G$ be the convex hull of those. Consider the convex hull $S$ of $B\cup G$. Then $S$ contains no black-black edge but contains some green-green edges (those obtained from white-white edges of $W$ on the boundary of $P$). Moreover, all green and black points are vertices of $S$ (as they are conspheric).
All this is now impossible by the following version of Steinitz’s non-inscribability criterion.
Claim. Suppose that $S$ is a (full-dimensional) convex polytope in $\mathbb R^n$ whose vertices are painted in black and green, such that no edge has two black endpoints. Suppose further that there are at least as many black vertices as green ones, and, if their numbers are equal, then there is at least one edge with two green endpoints. Then $S$ is not inscribed in a sphere.
For completeness, here is a sketch of the proof. Assume otherwise. Performing a projective transform of the sphere, we may assume that $S$ contains the center of the sphere in its interior. Now the tangents to the sphere at the vertices of $S$ (a.k.a. their polars w.r.t. the sphere) form the convex polytope $T$ dual to $S$, and $T$ is circumscribed. The facets of $T$ inherit the colors of the corresponding vertices of $S$. No two black facets have a common face of codimension 2, but some green facets do share such face if the numbers of black and green facets are equal.
Now, each face $f$ of codimension 2 is adjacent to two facets; in each of those, consider the solid angle based on $f$ with apex at the tangency point of the facet with the sphere. The two constructed solid angles are congruent. Using the properties mentioned above, we get that the sum of solid angles in green facets is strictly larger than that in black ones. However, the sum of solid angles in any facet is the same (it equals the area of the $(n -2)$-dimensional sphere). This contradicts the condition on the number of black and green facets.