The answer is No, there are no other such polytopes. The proof is quite laborious in parts, and I wrote it down in this article.
Theorem. In dimension $d\ge 4$, an edge-transitive polytope is vertex-transitive.
The idea is as follows: first, show that every edge-transitive polytope $P$ that is not vertex-transitive has the following three properties:
- all edges of $P$ are of the same length,
- $P$ has an edge in-sphere, and
- the edge-graph of $P$ is bipartite.
Call a polytope with these three properties bipartite.
One then tries to classify these polytopes instead.
This is easier, because every face of a bipartite polytope is again bipartite (not true for edge- or vertex-transitive polytopes).
The second step is to deal with all inscribed bipartite polytopes.
It is not hard to see that these are zonotopes. By a result from this article (see also this question), inscribed zonotopes with all edges of the same length are vertex-transitive. We can therefore exclude all the inscribed bipartite polytopes.
In the third step one classifies all the 3-dimensional non-inscribed bipartite polyhedra.
This is quite tedious.
Here is one example of a polyhedron which satisfies 2. and 3., but fails to have all edges of the same length.
The deviation is so miniscule, that it cannot be spotted visually.
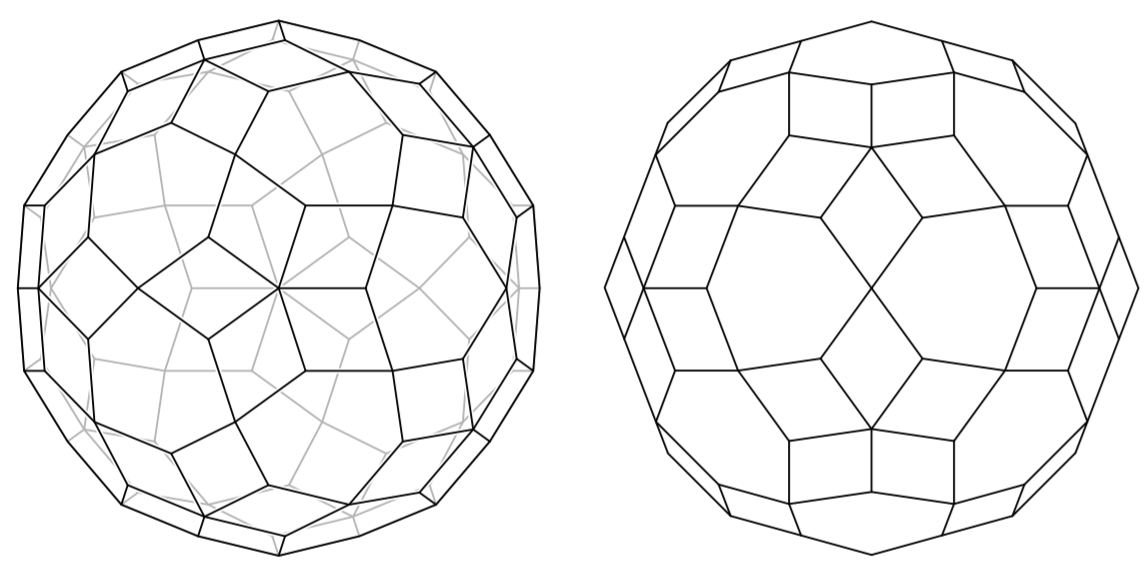
The result is then that there are only two such polyhedra: exactly those that I already mentioned in the question.
The final step is then to show that no 4-dimensional non-inscribed bipartite polytope can be built if we can use only these two polyhedra as facets. This uses a straight-forward argument on dihedral angles (see also Nick's answer).
 $\quad$$\quad$
$\quad$$\quad$

