2
$\begingroup$

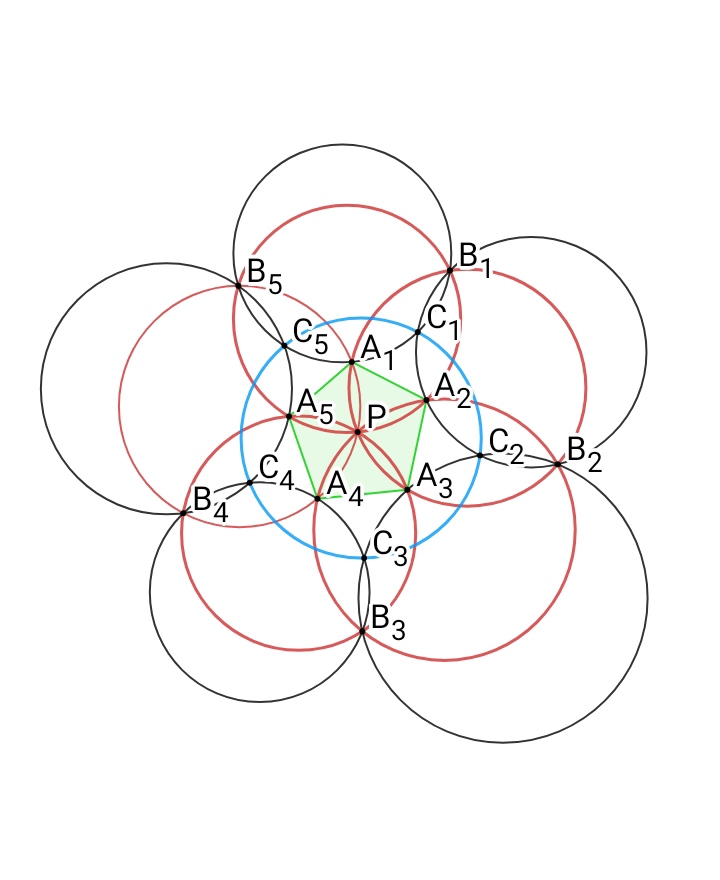
Let $A_1A_2A_3A_4A_5$ be irregular convex Pentagon and Let $P$ be arbitrary point anywhere in Plane geometry.

Let $X_1,X_2,X_3,X_4,X_5$ be Circumcircle of $\triangle PA1A3$; $\triangle PA2A4$; $\triangle PA3A5$; $\triangle PA4A1$; $\triangle PA5A2$.

Let $X_5 ∩ X_1= (P,B_1)$; $X_1 ∩ X_2= (P,B_2)$; $X_2 ∩ X_3= (P,B_3)$; $X_3 ∩ X_4= (P,B_4)$; $X_4 ∩ X_5= (P,B_5)$

Let $Z_1,Z_2,Z_3,Z_4,Z_5$ be Circumcircle of $\triangle B1B2A2$; $\triangle B2B3A3$; $\triangle B3B4A4$; $\triangle B4B5A5$; $\triangle B5B1A1$.

Let $Z_5 ∩ Z_1=(B_1,C_1)$; $Z_1 ∩ Z_2=(B_2,C_2)$; $Z_2 ∩ Z_3=(B_3,C_3)$; $Z_3 ∩ Z_4=(B_4,C_4)$; $Z_4 ∩ Z_5=(B_5,C_5)$; then Five point ${C_1,C_2,C_3,C_4,C_5}$ lies on Same Circle as Shown in this figure: Circle

Question : Is this above result is already mentioned anywhere?

Reference:

  1. Miquel Five point Circle

  2. SOME NEW THEOREM ON PENTAGON AND PENTAGRAM

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

This is just a variant of Miquel’s pentagram theorem. Just apply a circle inversion in a circle centered at $P$, and you will obtain the same configuration as the pentagram theorem. I’m not sure if this exact theorem is mentioned anywhere, but at least your result is readily deduced from Miquel’s theorem.

Improve this answer
$\endgroup$
3
  • $\begingroup$ I reflect the red circle of figure into blue circle then I take intersection of those 5 five Circumcircle which gives the Same Blue Circle. But my question is that when we reflect the circle in Circle , we get another Circle which is not straight lines but in Case of "Miquel five points Circle" we can see that he extend the line which is not a circle......, can you please give clarification on that?may be I am understanding it wrongly And thanks for answering it $\endgroup$
    – user423633
    Commented May 11, 2022 at 6:58
  • 1
    $\begingroup$ @JayendraandSankalp $P$ is not the center of the blue circle. It’s just a common point of the five red circles. Draw any circle $K$ with center $P$. Then invert the entire configuration in $K$. You will get Miquel’s configuration. In general, a circle that passes through the center of inversion is mapped to a straight line that doesn’t pass through it, and vice versa. In this case, the five red circles are transformed into five straight lines, forming a pentagon. $\endgroup$
    – K. Miyamoto
    Commented May 11, 2022 at 9:06
  • $\begingroup$ We have understood our mistake, thank you very much for your clarification. $\endgroup$
    – user423633
    Commented May 11, 2022 at 9:42

You must log in to answer this question.