mertens-function in the light of divergent summation - what summation method were best adapted

Question

Just reading about the Mertens-function in the other thread Mertens function I remember an earlier attempt to apply divergent summation to the series which is constructed of the Moebius-function at consecutive arguments, or in other words of which the Mertens-function-values represent the partial sums.

Eulersummation, although relatively poorly adapted, suggested that the (divergent) sum should be meaningfully evaluated to -2. But that sequence of partial sums (although seldom exceeding only the squareroot of its current index) seems to be a specific difficult case for such common summation methods - the approximation is relatively poor even for 128 terms. I tried Nörlund-means/Cesaro-sum, Euler-sums of different orders and also a selfmade matrix summation-method using the eulerian numbers (with which I could -on the other hand- well handle the even strongly diverging $ 0!-1!+2!-3!+...$ -series), but I tried not yet for instance Abel and Borel.

Q1: What method would be most appropriate to sum the series $ S = \sum _{k=1}^{\inf} moebius(k) $

Evaluation of 128 terms (Euler,Cesaro) suggested the result $S = -2$

Q2: And how could it be determined whether the Cesaro- and/or Euler-summation are at all capable to evaluate that series to a final value?

Here is 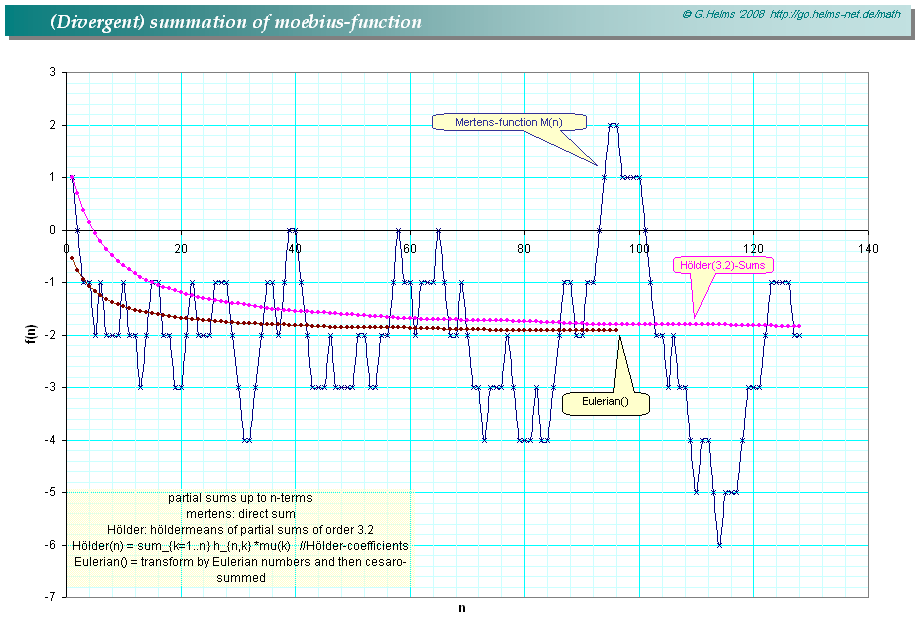of the summation.

Answer 1

Well, $$\sum_{n=1}^\infty\frac{\mu(n)}{n^s}=\frac1{\zeta(s)}$$ for $s>1$, so setting $s=0$ should give $$\sum_{n=1}^\infty\mu(n)=\frac1{\zeta(0)}=-2$$ as $\zeta(0)=-1/2$. :-)

I should add that this is a trick often used in analytic number theory (for instance in Eisenstein series). More generally given a divergent sum $$S=\sum_{i\in I}a_i$$ then consider, for an appropriate choice of weights $b_i>0$ the series $$f(s)=\sum_{i\in I}\frac{a_i}{b_i^s}.$$ We hope this converges in a suitable half-plane and can be analytically continued to $0$. Then we "define" $S=f(0)$.