Three circles meet at a point [closed]

Question

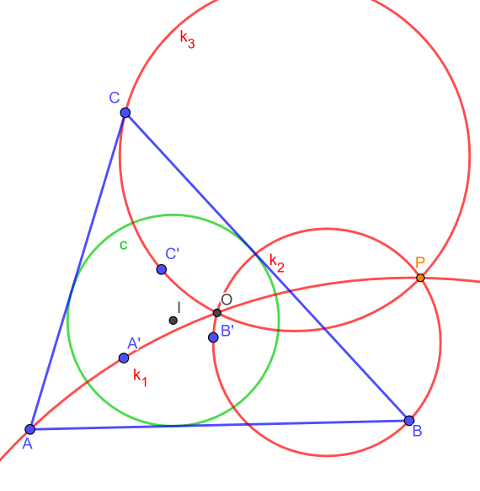
I am looking for the proof of the following proposition:

Proposition. Let $\triangle ABC$ be an arbitrary triangle with circumcenter $O$. Let $A',B',C'$ be a reflection points of the points $A,B,C$ with respect to the incircle of triangle. Consider the three circles $k_1,k_2,k_3$ defined by the points $AOA'$ , $BOB'$ and $COC'$ , respectively. I claim that $k_1$,$k_2$ and $k_3$ meet at a common point $P$.

The GeoGebra applet that demonstrates this proposition can be found here.

Answer 1

Let $P$ be the image of $O$ by the inversion with respect to the incircle. Since $IA\cdot IA'=IO\cdot IP=r^2$, we have that $A, A', O, P$ are concyclic, so $P$ is on $k_1$. Similarly $P$ is on the two other circles, which gives the result. Actually we used nothing special about $O$ here, the result still holds if you replace it by any other point.