5
$\begingroup$

The question is in the title:

Question: Is there any 4-dimensional polytope without 3-gonal and 4-gonal faces (of dimension two), other than the 120-cell?

I consider only convex polytopes (convex hull of finitely many points) that are full-dimensional (not contained in a proper subspace). And I consider a polytope to be distinct from the 120-cell if it has a non-isomorphic face-lattice.

It is known that any 4-polytope must have a 3-gonal, 4-gonal or 5-gonal face of dimension two. The 120-cell has only 5-gonal faces of dimension two.

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ "other than" means: not isomorphic as polyhedral complex? (this is a reasonable isomorphism notion; an a priori stronger one would be being isotopic, i.e., have a continuous deformation from one to another) $\endgroup$
    – YCor
    Commented May 23, 2020 at 16:38
  • $\begingroup$ @YCor Yes, thanks. I edited that into the question. $\endgroup$
    – M. Winter
    Commented May 23, 2020 at 16:41
  • $\begingroup$ Did you find who proved that the only possible faces are 3-, 4-, or 5-gonal? (An earlier version requested a citation for that result.) $\endgroup$
    – Brian Hopkins
    Commented May 23, 2020 at 16:54
  • 2
    $\begingroup$ @BrianHopkins I still don't have a source, but I realized the following: one can show (via a standard double counting arguments) that a planar graph has a vertex of degree 5 or smaller, or equivalently (considering its dual), a 3-gonal, 4-gonal or 5-gonal face. Since the edge-graph of a (3-dimensional) polyhedron is a planar graph, this proves it in dimension three. This then carries over to higher dimensions by considering the 3-faces of the polytopes. $\endgroup$
    – M. Winter
    Commented May 23, 2020 at 16:57
  • 1
    $\begingroup$ I found this highly relevant question with an equivalently relevant answer. $\endgroup$
    – M. Winter
    Commented May 23, 2020 at 18:17

2 Answers 2

Reset to default
8
$\begingroup$

There are other polytopes. To construct one let's do the following. Remember first that in the hyperbolic $4$-space there exists a regular compact right-angled 120-cell. Here, right-angled means that any two adjacent faces intersect under angle $\frac{\pi}{2}$. Regular means, that all the faces are isomeric, and the polytope has the same group of self-isometries as the Euclidean 120-cell. This polytope is discussed, for example, in

https://pdfs.semanticscholar.org/a0eb/ccbed0687d966a9aaaac2f370bc930a556be.pdf

at the bottom of page 65. The references to more classical articles are given there.

Now, if we double it in one face then we get a new convex polytope, and it is not hard to see, that it doesn't have 2-faces that are triangles and quadrilaterals. But any convex hyperbolic polytope is also combinatorially equivalent to a Euclidean one.

More generally, you can take any compact right-angled hyperbolic polytope in $\mathbb H^4$. Since it is hyperbolic and right-angled, it can not have $2$-faces that are triangles of quadrilaterals. And there is a infinite number of such polytopes in dimension 4. Each of them gives a Euclidean one as well.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thank you for your answer. I am not familiar with some terminology: 1. what is this "regular compact right-angled 120-cell" in hyperbolic 4-space. Is it like a tiling of 4-space? I wasn't able to find out what a "convex hyperbolic polytope" is via google. 2. What does it mean to "double it on one face"? $\endgroup$
    – M. Winter
    Commented May 23, 2020 at 17:06
  • $\begingroup$ I added a reference. Yes, starting with such a polytope you get a tiling of $\mathbb H^4$, in the same way as cubes tile $\mathbb R^n$. And what I say is that you need to take the union of two tiles that share a common hyperface to get a new polytope as you want. This can be continued as far as you want. $\endgroup$
    – Dmitri Panov
    Commented May 23, 2020 at 17:13
0
$\begingroup$

Dmitri's answer is definitely correct. I just want to add my geometric intuition, and a generalization, which, in hindsight, is quite obvious.

All in all, we can have the following:

If $P\subset\Bbb R^d$ is a polytope with $n$ facets, each of which is combinatorially (or projectively) equivalent to $Q\subset\smash{\Bbb R^{d-1}}\!$, then for each $k\ge 1$ there also exists a polytope $P_k\subset\Bbb R^d$ with $k(n-2)+2$ facets, all of which are combinatorially (or projectively) equivalent to $Q$.

With this, it should be clear that there are many 4-polytopes with only 5-gonal 2-faces.

The main idea is visualized below.

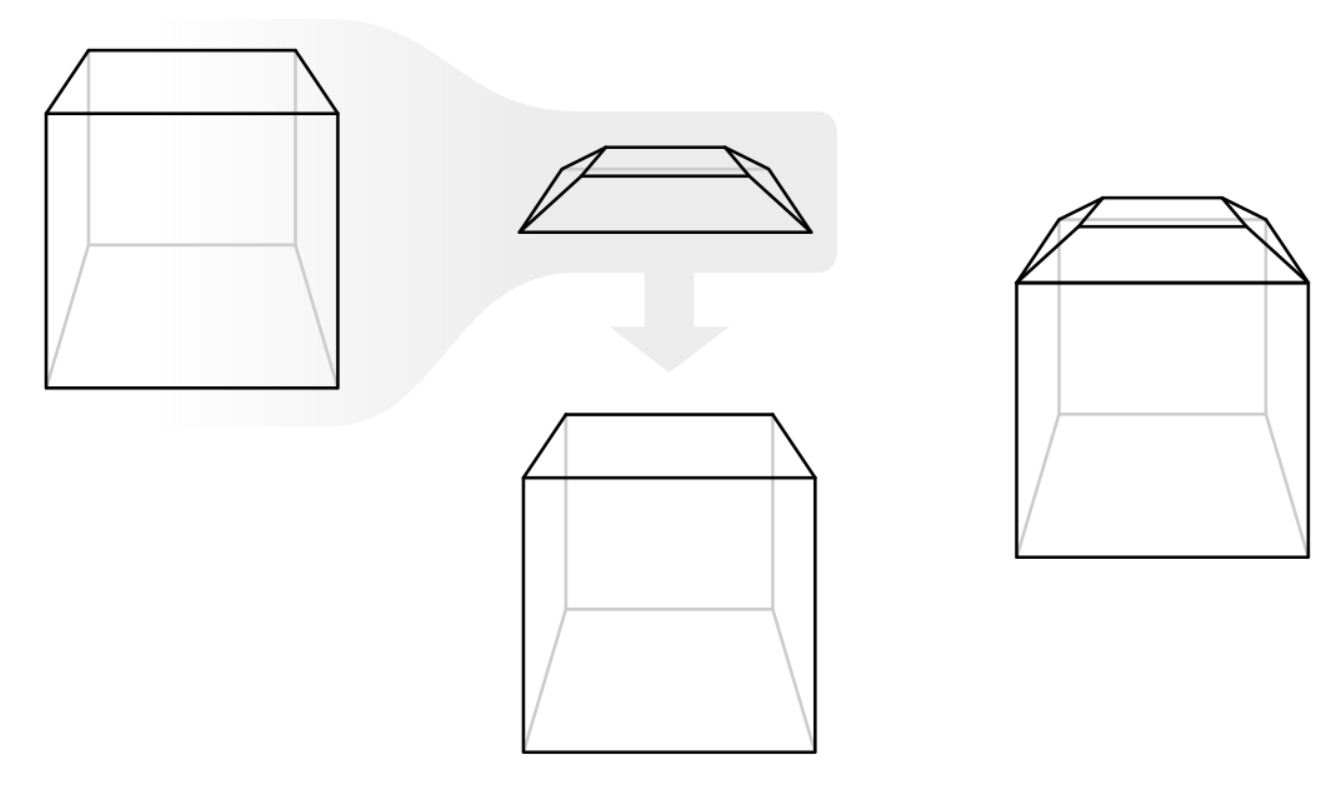
Construction:

  1. Fix a face $\sigma\subset P$.
  2. Let $P'$ be the polytope obtained from $P$ by applying a certain projective transformation that a) fixes $\sigma$, and b) moves all vertices of $P$ "beyond" $\sigma$ (see the image). This construction is related to the idea behind the Schlegel diagram, in particular, this transformations always exists.
  3. Glue $P'$ and $P$ on their common face isomorphic to $\sigma$ (if we have chosen the correct transformation in 2., then this is a convex polytope).

Repeat this to obtain as many $Q$-facets as you like.

Still, it might be interesting to determine the atomic $Q$-facetted polytopes, i.e. those, which are not "stacked" in the sense above.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .