The following is about getting help for a proof on existence and indexability of periodic points of the exponential-function, here with base $e:=\exp(1)$.
Update The question is a complete rewriting of the previous formulation of my question which I hope is much better focused and straightforward.
Let us define $f(z):=\exp(z)$ for $z \in \mathbb C$. Iteration may be denoted by $f°^1(z)=f(z)$ and $f°^{h+1}(z)=f°^{h}(f°^1(z))$.
Fixpoints: it is known (for instance W. Bergweiler1, pg 16),
- that $f$ has infinitely many fixpoints (=$1$-periodic points) $p_1$,
- that all of them are non-real, and
- that all of them are repelling.
They may be indexed by the branchindex $k \in \mathbb Z$ used in the Lambert-$W(k;z)$-function like $p_{1:k}$.
Periodic points: it is further known that for all $n$ the sets of $n$-periodic points are as well infinite1(pg.16). Let us denote such a set from now $\mathbb P_n$. So, in generalization of the indexing of $1$-periodic points, one might say, that the set $\mathbb P$ of all $\mathbb P_n , \text{with } n=1 \ldots \infty $ can be indexed by $\mathbb Z^\infty$.
But I think this is not precise enough; I assume, and would like to prove,
- (Conj. 1) that actually $\mathbb P_n$ can exactly be indexed by $\mathbb Z^n$ $\qquad \Leftarrow \mathbb {\text{problem to be proved}}$
For my approach arguing for (Conj. 1) I refer to the property, that a fixed- or periodic points, which is repelling for iteration over function $f()$, is attracting for iteration over its inverse, which means is attracting for the iteration over the $\log()$-function. From Bergweiler, pg. 17, I take, that all periodic points are repelling on iteration on $f()$ and thus are all attracting for its inverse.
For convenience of further notation let us define $\log()$ as $g(x):=\log(x)$ and as well the iteration $g°^1(z)=g(z)$ and $g°^{h+1}(z)=g°^{h}(g°^1(z))$.
To make $g()$ a true inverse of $f()$, we'll need the branch index(es) explicite, so let us simply extend the notation $$g(x,k):=\log(x) + k \cdot C \qquad \text{where } C=2 \pi î$$ This allows to make precisely for some fixed $z$ $$ g(f(z),0)=z $$ but for the reversion of some $z'=z + k\cdot C$ we need $$ g(f(z'),k)=g(f(z+k\cdot C),k) =g(f(z),k)=z+k \cdot C=z' $$
For adressing periodic points of period-length $n$ we expand the notation further $$ \begin{array}{} g(z,[k_1])&:= g(z,k_1) \\ g(z,[k_1,k_2])&:= g(g(z,k_1),k_2) \\ g(z,[k_1,k_2,...,k_n])&:= g(...g(g(z,k_1),k_2)...,k_n) \\ &\small \text{where all $k_j \in \mathbb Z$}\\ \end{array}$$
Finally I use $K_n:=[k_1,k_2,...,k_n]$ for the vector of branch-indexes. With this I conjecture now the following:
- iterations of each expression $z_{i+1}=g(z_i,K_n)$ are attracting.
- we can approximate any periodic point $p_{n,K}$ by simple fixed-point iteration of the previous with some suitable initial value $z_0 \ne 0$ according to $$p_{n,K} = \lim_{i \to \infty} z_{i+1}=g(z_i,K_n)$$ (of course we can increase speed of approximation when Newton-iteration on $g()$ follows).
- the iteration for a given $K_n$ is attracting over the whole complex plane except for the initial values $z_0 \in \{0,1,e,e^e,...\}$.
Non uniqueness occurs only for $K=[0]$ (and its non-primitive repetitions $K=[0,0]$,... $K=[0,0,...,0]$) in that the initial value $z_0$ is relevant for to converge towards the $1$-periodic point either in the upper or in the lower half plane. - Main conjecture to be proved: All $n$-periodic points with the exception of the conjugated primary fixed points $p_{1:[0]}$ and $\overline {p_{1:[0]}}$ (which have the same branch index-vector $K=[0]$) are in bijection to the indexes $K_n$ and can be approximated by simple fixed-point iteration over $g(z,K_n)$ (if desired followed by Newton-iteration on $g(z,K_n)$ to speed up convergence).
Remark: I have seen, that with exponential bases different from $e:=exp(1)$ spuriously non-uniquenesses and non-existences of $n$-periodic points occur, which I cannot yet nail down except by giving a couple of heuristic examples. However, large surveys on the exponential with base $e$ -as discussed here- seem to have only that one exception as mentioned in (3.).
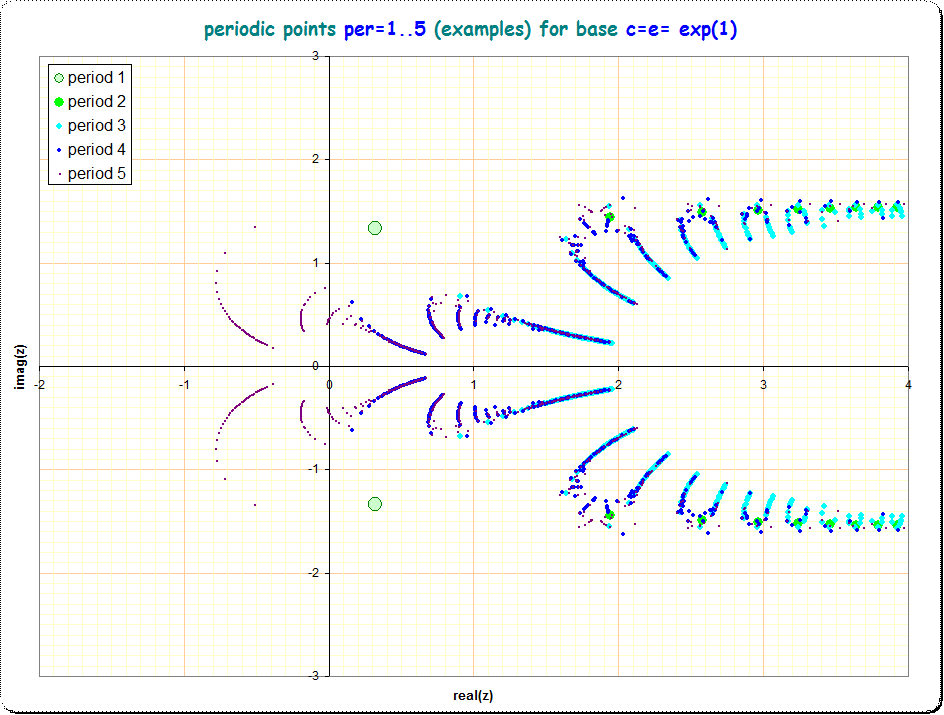
An illustration of periodic points of periods $n=1..5$ . Those were found by screening the square $-4-4î...4+4î$ on the complex plane in steps by $1/40$ with the newton-iteration applied. The list has then been checked whether they all agree with the $K_n$-indexing scheme; all found periodic points have a valid $K$-index.
A long & wide discussion (using other bases than $e$, and using another ansatz for partial solutions) can be found at MSE
A handful of used literature: I've found some resources on fixed points and their properties for the exponential function base $e$, but less about $n$-periodic points. The most fruitful so far was the habilitation of Walter Bergweiler, 1991. If there are more comprehensive texts (optimally online available), please leave a comment.
1Bergweiler, Walter, Periodische Punkte bei der Iteration ganzer Funktionen, Aachen: Rheinisch-Westfälische Techn. Hochsch., Math.-Naturwiss. Fak., Habil.-Schr. 51 S. (1991). ZBL0728.30021.
Pg.16:
- "Dieses Ergebnis wurde im Jahre 1948 duch Rosenbloom verallgemeinert, der zeigte, daß für jedes $n \gt 2$ unendlich viele periodische Punkte der Periode $n$ existieren"
- "Baker im Jahre 1960 (...) bewies (...), daß höchstens eine (von $f$ abhängige) natürliche Zahl $n$ existiert mit der Eigenschaft, daß $f$ nur endlich viele periodische Punkte der primitive Periode $n$ hat."
Pg. 17:
- "Satz 2: Es sei $f$ eine ganze transendente Funktion und es sei $n \ge 2$. Dann hat $f$ unendlich viele abstoßende periodische Punkte der primitiven Periode $n$."
- "Wir bemerken noch, daß ganze Funktionen keine anziehenden periodischen Punkte zu haben brauchen. Ein Beispiel, (...) ist durch $f(z)=e^z$ gegeben."
Additional readings:
Hellmuth Kneser, [Real analytic solutions of the equation $φ(φ(x))=e^x$ and related functional equations. (Reelle analytische Lösungen der Gleichung $φ(φ(x))=e^x$ und verwandter Funktionalgleichungen.)], J. Reine Angew. Math. 187, 56-67 (1949)(German).Zbl0035.04801.4
Shen, Zhaiming; Rempe-Gillen, Lasse, The exponential map is chaotic: an invitation to transcendental dynamics, Am. Math. Mon. 122, No. 10, 919-940 (2015). ZBL1361.37002.5
Here general aspects of the set of $n$-periodic points are presented in existence-theorems. Even the concept of infinite non-periodic, but not diverging-to-infinity, orbits -as part of the general chaotic behaviour- is covered by the list of theorems.(G.H.)
An introductory article which deals with the question of $\mathbb P_1$ (fixed-) points on the branches of the $\log()$-function by Stanislav Sykora (2016) at his web-space.
