Polynomial inequality of sixth degree

Question

There is the following problem.

Let $a$, $b$ and $c$ be real numbers such that $\prod\limits_{cyc}(a+b)\neq0$ and $k\geq2$ such that $\sum\limits_{cyc}(a^2+kab)\geq0.$ Prove that: $$\sum_{cyc}\frac{2a^2+bc}{(b+c)^2}\geq\frac{9}{4}.$$

I have a proof of this inequality for any $k\geq2.6$.

I think, for $k<2.6$ it's wrong, but my software does not give me a counterexample

and I don't know, how to prove it for some $k<2.6$.

It's interesting that without condition $\sum\limits_{cyc}(a^2+kab)\geq0$ the equality occurs also for $(a,b,c)=(1,1,-1)$.

My question is: What is a minimal value of $k$, for which this inequality is true?

Thank you!

Answer 1

We want to show that your inequality does not hold for $k\in[2,13/5)$. In view of the identity in your answer, it is enough to show that for each $k\in[2,13/5)$ there is a triple $(a,b,c)\in\mathbb R^3$ with the following properties: $a=-1>b$, \begin{align}s_4&:=\sum_{cyc}(2a^3-a^2b-a^2c) \\ &=a^2 (2 a-b-c)+b^2 (-a+2 b-c)+c^2 (-a-b+2 c)=0, \end{align} $$s_3:=a b + b c + c a<0,$$ and $$s_2+k s_3=0,$$ where $$s_2:=a^2 + b^2 + c^2.$$ Indeed, then the right-hand side of your identity will be $$\frac{20}{3}\sum_{cyc}(a^4-a^2b^2)(13/5-k)s_3<0,$$ so that your identity will yield $$\sum_{cyc}\frac{2a^2+bc}{(b+c)^2}<9/4.$$

For each $k\in(2,13/5)$, the triple $(a,b,c)$ will have all the mentioned properties if $a=-1$, $b$ is the smallest (say) of the 6 real roots $x$ of the polynomial $$P_k(x):=-18 - 15 k + 4 k^2 + 4 k^3 + (36 k + 6 k^2 - 12 k^3) x + (-27 - 9 k - 21 k^2 + 6 k^3) x^2 + (18 + 60 k - 10 k^2 + 8 k^3) x^3 + (-27 - 9 k - 21 k^2 + 6 k^3) x^4 + (36 k + 6 k^2 - 12 k^3) x^5 + (-18 - 15 k + 4 k^2 + 4 k^3) x^6, $$ and $$c=\tfrac12\, (k - b k) - \tfrac12\, \sqrt{-4 - 4 b^2 + 4 b k + k^2 - 2 b k^2 + b^2 k^2}.$$ For $k=2$, $(a,b,c)=(-1,0,1)$ will be such a triple.

So, we are done.

---

This result was obtained with Mathematica, as follows (which took Mathematica about 0.05 sec):

Answer 2

Your inequality fails to hold when e.g. $k=25999/10000=2.6-10^{-4}$ and $(a,b,c)=(97661/65536,-5/3,-1)$.

---

Indeed, the smallest value for which your inequality holds is $13/5=2.6$. Here is a proof by Mathematica:

So, the value $13/5$ of $k$ is witnessed by $$a=-1,\ b=x_*,\ c=\frac{1}{10} \left(13-13 x_*-\sqrt{69 x_*^2-78 x_*+69}\right),\tag{1}$$ where $x_*=-1.68\ldots$ is the smallest root of the $6$ real roots of the polynomial $$p(x)=1681 - 3198 x - 3621 x^2 + 10292 x^3 - 3621 x^4 - 3198 x^5 + 1681 x^6.$$

For this proof, Mathematica took about 32 sec, which is a huge time for a computer.

---

The values of the sums $$(s_1,s_2,s_3):=\left(\frac{2 a^2+b c}{(b+c)^2}+\frac{2b^2+a c}{(a+c)^2}+\frac{2 c^2+a b}{(a+b)^2},a^2+b^2+c^2,a b+a c+b c\right)$$ for the extremal $(a,b,c)$ given by (1) are
$$\Big(\frac94,-\frac{13}5\,s_{3*},s_{3*}\Big),$$ where $s_{3*}=-2.34\ldots$ is the smallest root of the $3$ real roots of the polynomial $$p_3(x)=3375 + 8775 x + 7065 x^2 + 1681 x^3,$$ with the other two roots $-1.04\ldots$ and $-0.826\ldots$.

Answer 3

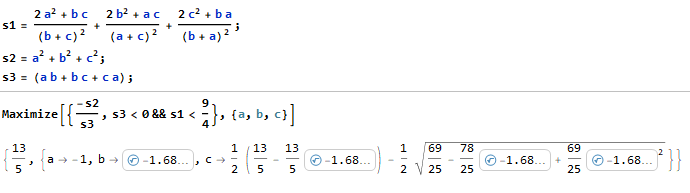
I have found the following identity, which solves my problem for $k=\frac{13}{5}.$ $$4\prod_{cyc}(a+b)^2\left(\sum_{cyc}\frac{2a^2+bc}{(b+c)^2}-\frac{9}{4}\right)=\frac{1}{3}\left(\sum_{cyc}(2a^3-a^2b-a^2c)\right)^2+$$ $$+\frac{20}{3}\sum_{cyc}(a^4-a^2b^2)\sum_{cyc}\left(a^2+\frac{13}{5}ab\right).$$ I got this identity by the following reasoning.

Let $a+b+c=3u$, $ab+ac+bc=3v^2$, $abc=w^3$ and $\mathbb{w}(p)$ be a coefficient before $w^6$ in writing of a symmetric polynomial $p$ of three variables $a$, $b$ and $c$ as a polynomial of $u$, $v^2$ and $w^3$.

Thus, $$\mathbb{w}\left(4\prod_{cyc}(a+b)^2\left(\sum_{cyc}\frac{2a^2+bc}{(b+c)^2}-\frac{9}{4}\right)\right)=$$ $$=\mathbb{w}\left(4\sum_{cyc}(2a^2+bc)(a^2+3v^2)^2-9(9uv^2-w^3)^2\right)=$$ $$=\mathbb{w}(8(a^6+b^6+c^6)+4abc(a^3+b^3+c^3)-9w^6)=24+12-9=27.$$ Now, we'll choose $m$, $n$ and $k$ such that the inequality $$4\prod_{cyc}(a+b)^2\left(\sum_{cyc}\frac{2a^2+bc}{(b+c)^2}-\frac{9}{4}\right)\geq$$ $$\geq\frac{1}{3m^2}\left(\sum\limits_{cyc}(a^3+m(a^2b+a^2c)-(2m+1)abc)\right)^2+$$ $$+n\sum_{cyc}(a^4-a^2b^2)\sum_{cyc}(a^2+kab)$$ would be true for any reals $a$, $b$ and $c$ such that $\sum\limits_{cyc}(a^2+kab)\geq0.$

Indeed, since $$\mathbb{w}\left(\frac{1}{3m^2}\left(\sum\limits_{cyc}(a^3+m(a^2b+a^2c)-(2m+1)abc)\right)^2\right)=$$ $$=\mathbb{w}\left(\frac{1}{3m^2}(3w^3-3mw^3-3(2m+1)w^3)^2\right)=27,$$ we see that the last inequality is a linear inequality of $w^3$,

which by $uvw$ (see here: https://artofproblemsolving.com/community/c6h278791 ) says that it's enough to assume $b=c=1$ (the case $b=c=0$ we can check later).

Also, since for $b=c=1$ we have $$4\prod_{cyc}(a+b)^2\left(\sum_{cyc}\frac{2a^2+bc}{(b+c)^2}-\frac{9}{4}\right)=8(a-1)^2(a+1)^2(a+2)^2$$ and $$\sum_{cyc}(a^4-a^2b^2)=(a^2-1)^2,$$ we see that $$\sum\limits_{cyc}(a^3+m(a^2b+a^2c)-(2m+1)abc)$$ has for $b=c=1$ a factor $a+1$, which gives $m=-\frac{1}{2}$ and we obtain: $$8(a+1)^2(a-1)^2(a+2)^2\geq$$ $$\geq\frac{4}{3}(a-1)^4(a+1)^2+n(a-1)^2(a+1)^2(a^2+2+k(2a+1))$$ or $$(20-3n)a^2+(104-6kn)a+92-3n(k+2)\geq0,$$ for which we need $$20-3n\geq0$$ and $$(52-3kn)^2-(20-3n)(92-3n(k+2))=0,$$ where the last it's $$(4+2n-kn)(kn+n-24)=0.$$ Here both cases give a same result.

For example, $$n=\frac{24}{k+1}$$ gives $$20-\frac{72}{k+1}\geq0$$ or $$k\geq\frac{13}{5}.$$ For $k=\frac{13}{5}$ we obtain $n=\frac{20}{3}$ and for these values of $n$ and $k$ our inequality turned out an identity!