Your inequality fails to hold when e.g. $k=25999/10000=2.6-10^{-4}$ and $(a,b,c)=(97661/65536,-5/3,-1)$.
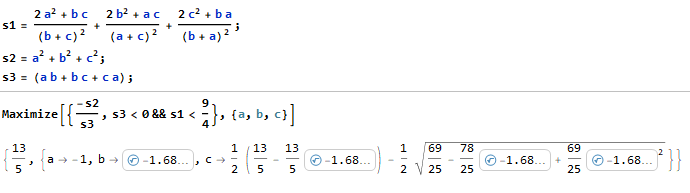
Indeed, the smallest value for which your inequality holds is $13/5=2.6$. Here is a proof by Mathematica:
So, the value $13/5$ of $k$ is witnessed by $$a=-1,\ b=x_*,\ c=\frac{1}{10} \left(13-13 x_*-\sqrt{69 x_*^2-78 x_*+69}\right),$$$$a=-1,\ b=x_*,\ c=\frac{1}{10} \left(13-13 x_*-\sqrt{69 x_*^2-78 x_*+69}\right),\tag{1}$$ where $x_*=-1.68\ldots$ is the smallest root of the $6$ real roots of the polynomial $$p(x)=1681 - 3198 x - 3621 x^2 + 10292 x^3 - 3621 x^4 - 3198 x^5 + 1681 x^6.$$
For this proof, Mathematica took about 32 sec, which is a huge time for a computer.
The values of the sums
$$(s_1,s_2,s_3):=\left(\frac{2 a^2+b c}{(b+c)^2}+\frac{2b^2+a c}{(a+c)^2}+\frac{2 c^2+a b}{(a+b)^2},a^2+b^2+c^2,a b+a c+b
c\right)$$
for the extremal $(a,b,c)$ given by (1) are
$$\Big(\frac94,-\frac{13}5\,s_{3*},s_{3*}\Big),$$
where $s_{3*}=-2.34\ldots$ is the smallest root of the $3$ real roots of the polynomial
$$p_3(x)=3375 + 8775 x + 7065 x^2 + 1681 x^3,$$
with the other two roots $-1.04\ldots$ and $-0.826\ldots$.