Let $(M,g)$ be a complete $d$-dimensional Riemannian manifold, $p \in M$ be fixed and let $C_p$ be the cut-locus of $p$.
Other than when $M$ is non-positively curved (in which $C_p=
\emptyset$ by Cartan-Hadamard) when is the cut-locus contained in a collared neighbourhood ie: an open subset $U\subseteq M$ containing $C_p$ satisfying and a homeomorphism $f:U\rightarrow C_p \times [0,1)$ such that
$$
f[C_p]=C_p \times \{0\}.
$$
$\begingroup$
$\endgroup$
12
-
4$\begingroup$ Collared by normal coordinates, or just topologically collared? $\endgroup$– Ben McKayCommented Mar 31, 2020 at 16:41
-
4$\begingroup$ Could you define "topologically collared"? The cut locus $C_p$ is not a submanifold. For example on a surface it should be a topologically embedded tree, and so it cannot have a neighborhood homeomorphic to $[0,1]\times C_p$ because the latter is not a manifold. $\endgroup$– Igor BelegradekCommented Mar 31, 2020 at 18:56
-
3$\begingroup$ And the tree need not be locally finite, as was famously shown by Gluck and Singer in "Scattering of Geodesic Fields, I". Actually, in general on a surface, it is a graph not a tree, see arxiv.org/abs/1103.1759. $\endgroup$– Igor BelegradekCommented Mar 31, 2020 at 19:05
-
2$\begingroup$ As I explained in my comment above your definition of collared cannot work. Imagine that the cut locus $C$ in a surface is a tripod (the graph with 3 edges joined in a common vertex). Such examples are in the paper "Every graph is a cut locus" linked above. No neighborhood of a tripod in a $2$-manifold is homeomorphic to $C\times [0,1)$ because the latter is not a manifold. $\endgroup$– Igor BelegradekCommented Mar 31, 2020 at 22:47
-
3$\begingroup$ @AIM_BLB that's certainly a correct theorem but you didn't say anything about boundary in your question so I assumed you meant a manifold without boundary. For manifolds without boundary what you ask for is impossible unless the cut locus is empty. It is of course possible for manifolds with boundary (e.g. a closed disk in $R^n$) provided you define cut locus appropriately. $\endgroup$– Vitali KapovitchCommented Apr 2, 2020 at 16:49
|
Show 7 more comments
1 Answer
$\begingroup$
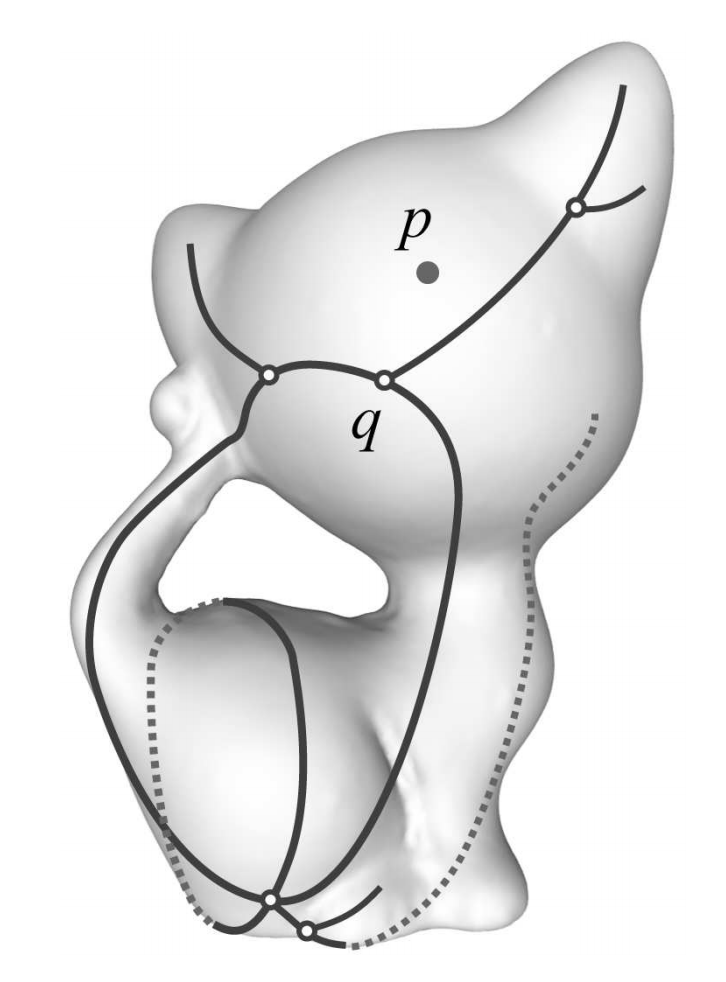
The source point $p$ is on the cat's forehead, the other side in this rear-view.
$\endgroup$
Permit me to include this nice image from Day & Li to illustrate @Igor's point that "in general on a surface, it [the cut locus] is a graph not a tree."

The source point $p$ is on the cat's forehead, the other side in this rear-view.
Dey, Tamal K., and Kuiyu Li. "Cut locus and topology from surface point data." In Proceedings of the 25th Symposium on Computational Geometry, pp. 125-134. 2009. ACM link.
In the paper they compute an approximation to the cut locus on this model that pretty much follows the smooth curves drawn above.
