Permit me to include this nice image from Day & Li to illustrate @Igor's point that "in general on a surface, it [the cut locus] is a graph not a tree."
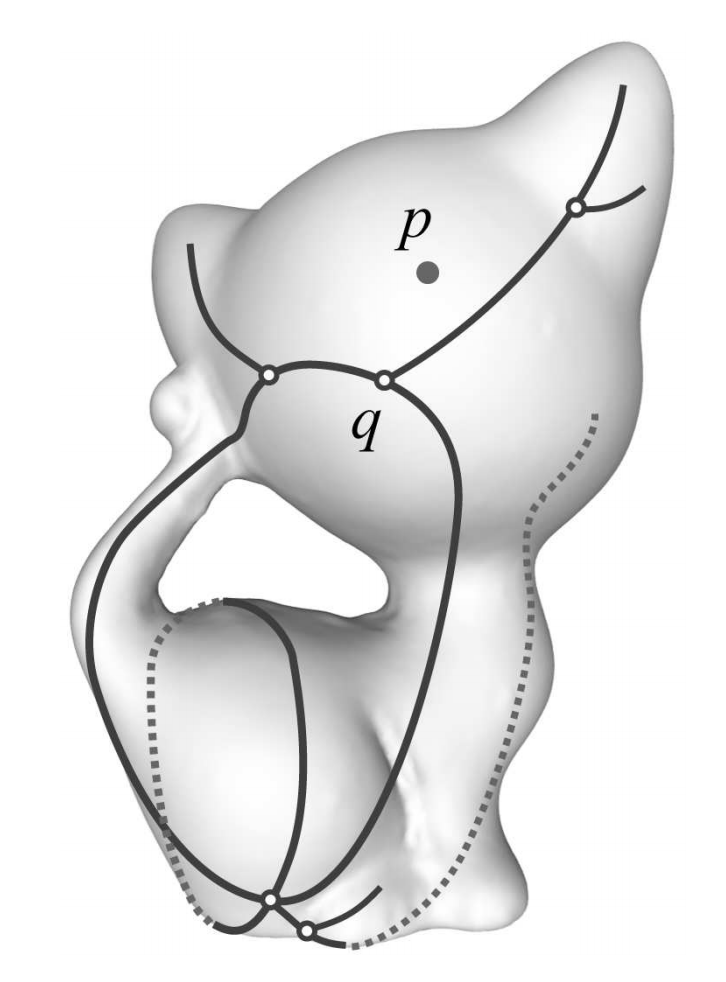
The source point $p$ is on the cat's forehead, the other side in this backrear-view.
>Dey, Tamal K., and Kuiyu Li. "Cut locus and topology from surface point data." *In Proceedings of the 25th Symposium on Computational Geometry*, pp. 125-134. 2009. [ACM link](https://dl.acm.org/doi/abs/10.1145/1542362.1542390).
In the paper they compute an approximation to the cut locus on this model that pretty much follows the smooth curves drawn above.
