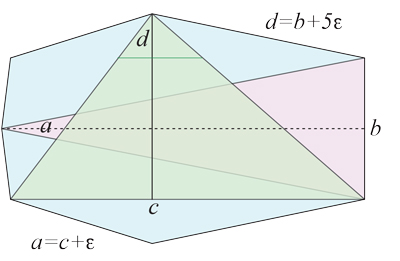
Given any 2D convex region $C$ with a mirror symmetry. Two pairs of questions:
We need to find the smallest area (likewise, smallest perimeter) triangle that contains $C$. Is it sufficient to only search among isosceles triangles aligned along the direction of mirror symmetry of $C$ for answers to both questions?
Similarly, if we seek the largest area (largest perimeter) triangle contained within $C$, is it enough to look only among isosceles triangles aligned along the direction of symmetry of $C$?