Let $u=(u_1,...,u_n), v=(v_1,...,v_n)$ be two random vectors independently and uniformly distributed on the unit sphere in $\mathbb{R}^n$. Define two other random variables $X=\sum_{i=1}^nu_i^2v_i^2$, $Y=u_1^2v_1^2$. Consider the following ratio of expectation: $$r_n(\alpha)=\frac{\mathbb{E}\{\exp[-\alpha^2\frac{1-X}{2}]\}}{\mathbb{E}\{\exp[-\alpha^2(1-Y)]\}}$$ Does there exist a finite upper bound for $r_n(\alpha)$, independent of $\alpha$? I'm interested in the behavior with fixed $n$ and large $\alpha$.
Update:
I did some simulation, the answer seems to be negative. Below is a plot for $n=4$ using Monte Carlo simulation by averaging 20000 samples on the numerator and denominator respectively.
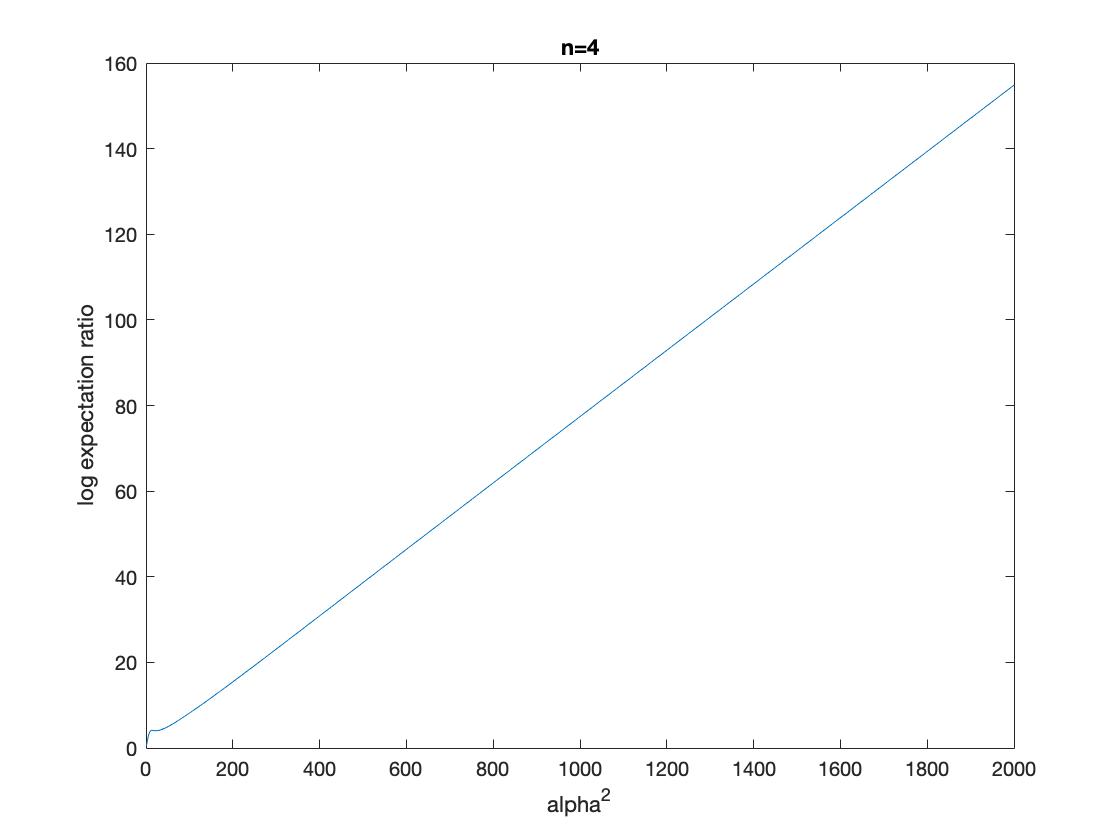
The result is similar for $n=3$. However, for $n=2$, the result is pretty striking:
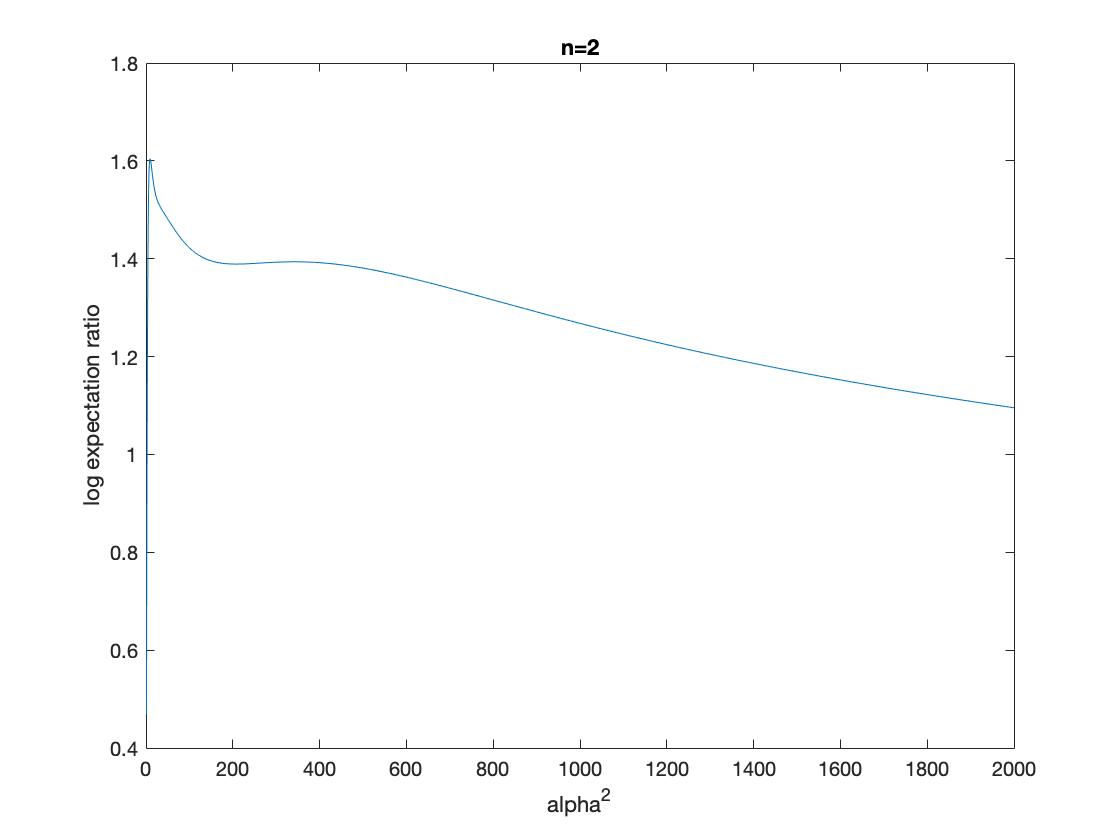
It should also be pointed out that there seems to a critical point for $\alpha$ when $\alpha^2$ is around 10, for all $n=2,3,4$.
