$\newcommand{\al}{\alpha}
\newcommand{\be}{\beta}
\newcommand{\de}{\delta}
\newcommand{\De}{\Delta}
\newcommand{\ep}{\varepsilon}
\newcommand{\ga}{\gamma}
\newcommand{\Ga}{\Gamma}
\newcommand{\la}{\lambda}
\newcommand{\si}{\sigma}
\newcommand{\Si}{\Sigma}
\newcommand{\thh}{\theta}
\newcommand{\om}{\omega}
\newcommand{\R}{\mathbb{R}}
\newcommand{\Z}{\mathbb{Z}}
\newcommand{\F}{\mathcal{F}}
\newcommand{\E}{\operatorname{\mathsf E}}
\newcommand{\Var}{\operatorname{\mathsf Var}}
\renewcommand{\P}{\operatorname{\mathsf P}}
\newcommand{\ii}[1]{\operatorname{\mathsf I}\{#1\}}
\newcommand{\eD}{\overset{\text{D}}\to}
\newcommand{\D}{\overset{\text{D}}=}
\newcommand{\tsi}{\tilde\si}$
We may write $z_i=U_i+V$ for $i\in[n]:=\{1,\dots,n\}$, where $U_1,\dots,U_n,V$ are iid standard normal random variables. Let $\Phi$ and $\phi$ denote the standard normal cdf and pdf, respectively.
Then for
\begin{equation}
m:=\min_{1\le i\le n}|z_i|^2
\end{equation}
and any real $s>0$ we have
\begin{align*}
\P(m>s^2)&=\P(|U_i+V|>s\ \forall i\in [n]) \\
&=\int_\R dv\,\phi(v)\,\P(|U_i+v|>s\ \forall i\in [n]) \\
&=\int_\R dv\,\phi(v)g(s,v)^n,
\end{align*}
where
\begin{equation}
g(s,v):=\bar\Phi(s-v)+\bar\Phi(s+v)[\in(0,1)]
\end{equation}
and $\bar\Phi:=1-\Phi$.
Hence,
\begin{align*}
\E m&=\int_0^\infty2s\,ds\,\P(m>s^2) \\
&=\int_0^\infty2s\,ds\,\int_\R dv\,\phi(v)g(s,v)^n \\
&=4\int_0^\infty s\,ds\,\int_0^\infty dv\,\phi(v)g(s,v)^n.
\end{align*}
I think this expression for $\E m$ is amenable to bounding and asymptotic analysis, for which, unfortunately, I do not have time at this point. Hopefully, someone else (or maybe I) can do this later.
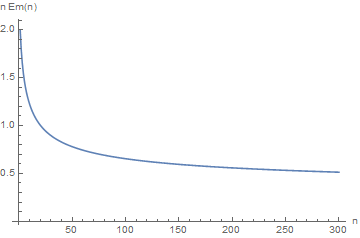
The following plot of the values $n\E m(n)$ (where $m(n):=m$) suggests that $m$ is indeed on the order of $1/n$:
Added: The next step could be as follows. For $t\in(0,1)$, let
\begin{equation}
G(t):=\int_0^\infty s\,ds\,\int_0^\infty dv\,\phi(v)\ii{g(s,v)>1-t}.
\end{equation}
Clearly, the function $G$ is increasing.
It should be not hard to see that $(1-t)^nG(t)\to0$ as $t\uparrow1$. Then, integrating by parts, we will have
\begin{equation}
\E m/4=\int_0^\infty s\,ds\,\int_0^\infty dv\,\phi(v)g(s,v)^n
=\int_0^1(1-t)^n\,dG(t)=n\int_0^1(1-t)^{n-1}\,G(t)\,dt.
\end{equation}
So, it appears that, to obtain the asymptotics of $\E m$ as $n\to\infty$, it suffices to find that of $G(t)$ as $t\downarrow0$. This will take some extra effort. I think MO users (me included) may be more inclined to take on this task if you could let us know the motivation behind your question, the context in which it arises.

