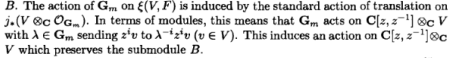3
$\begingroup$

I am reading Simpson's paper on The Hodge filtration on nonabelian cohomology. In particular Chapter 5 (p.24) and I am confused about the notion of a group acting on an equivariant sheaf.

The set up is as follows:

$\mathbb{A}^1=Spec(\mathbb{C}[z])$ admits an obvious action by the multiplicative group $\mathbb{G}_m=Spec(\mathbb{C}[z,z^{-1}])$ \begin{equation} a:\mathbb{G}_m\times \mathbb{A}^1\to \mathbb{A}^1. \end{equation} which is equivalent to giving the canonical grading on $\mathbb{C}[z]$ (cf. here).

Now what is a $\mathbb{G}_m$-equivariant vector bundle over $\mathbb{A}^1$?

For me this is a $\mathbb{G}_m$-equivariant locally free sheaf $W$. In the affine case this reduces to a locally free module $B=W(\mathbb{A}^1)$ with a grading that respects the grading of $\mathbb{C}[z]$ or equivalently a comodule map $B\to B\otimes_\mathbb{C} \mathbb{C}[z,z^{-1}]$.

So I was quite surprised when I found the following comment in a more written out version of the paper*:

enter image description here

It seems like there is an identification $\mathbb{G}_m$ with $\mathbb{C}^*$, which is confusing to me.

  • How does this action of $\mathbb{G}_m$ (or rather $\mathbb{C}^*$ ?) on $\mathbb{C}[z,z^{-1}]\otimes_\mathbb{C} V$ induce an equivariant structure on the sheaf or equivalently a comodule structure?
  • Is my approach for the definition correct to begin with?

This question is cross-posted from stackexchange

Improve this question
$\endgroup$
5
  • $\begingroup$ I think it is a fairly standard abuse of notation to equate a scheme over $\mathbb C$ (e.g. $\mathbb G_m$) with its $\mathbb C$-points ($\mathbb G_m(\mathbb C) = \mathbb C^\times$). In my opinion it is quite natural to express the data of a $\mathbb G_m$ representation in the way the author does above. Such an expression can easily be translated in to your preferred form: the $i$th-graded piece of the module $\mathbb C[z,z^{-1}] \otimes V$ above consists of expressions of the form $z^{-i} \otimes v$. Perhaps this was not the cause of confusion here though? $\endgroup$
    – Sam Gunningham
    Commented Jun 8, 2019 at 12:00
  • $\begingroup$ I think the author is giving me an automorphism $\lambda^*:\mathbb{C}[z,z^{-1}]\otimes V\to \mathbb{C}[z,z^-1]\otimes V$ for each $\lambda\in \mathbb{C}^*$ (which he denotes by $\mathbb{G}_m$, alright). And intuition wise it is somehow natural that this corresponds to the grading you gave. Nevertheless I can't formalize it... $\endgroup$
    – Louis Jaburi
    Commented Jun 8, 2019 at 12:16
  • 1
    $\begingroup$ More generally, suppose you have an algebraic representation $X$ of an affine algebraic group $G$. As you say, $X$ can be formalized of as a comodule for $\mathcal O(G)$. But sometimes it is convenient to express this data in terms of an action map $G\times X \to X$, $(g,x) \mapsto g\cdot x$ (I guess technically I have in mind that $g$ denotes a $\mathbb C$-point of $G$)... $\endgroup$
    – Sam Gunningham
    Commented Jun 8, 2019 at 12:38
  • 1
    $\begingroup$ ...The fact that this action is algebraic means that for each $v\in X$ there is a formula of the form $g \cdot v = \sum f_i(g) v_i$ for some $f_i \in \mathcal O(G)$. But this is just the expression for the coaction $X \to \mathcal O(G) \otimes X$. Namely, $v \mapsto \sum f_i \otimes v_i$. $\endgroup$
    – Sam Gunningham
    Commented Jun 8, 2019 at 12:40
  • $\begingroup$ I think I am starting to understand! So I assume instead of $\mathbb{C}$ we could take any algebraically closed field $k$. Then I get an equivalence {Comodule map $X\to \mathcal{O}(G) \otimes X$} <-> {an action $\mathcal{O}(G) (k) \times X\to X$ such that the above condition is fulfilled}. Is that right? $\endgroup$
    – Louis Jaburi
    Commented Jun 8, 2019 at 13:19

0

Reset to default

You must log in to answer this question.