What are the possible linking matrices of a quasi-positive link?

Question

I was surprised recently to come across a 3-component link where the linking number of two of the components was negative. For a while I thought I had made a mistake, then I thought a little more and realized that there was no particular reason that the linking numbers had to be all positive.

The linking matrix I found was $$\begin{pmatrix} * & 3 & 3 \\ 3 & * & -1 \\ 3 & -1 & * \end{pmatrix}. $$ Here is the link. (I haven't attempted to find a quasi-positive representative yet, the proof it's quasi-positive was pretty indirect.)

Answer 1

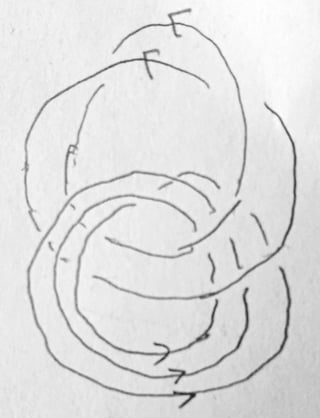
There seem to be hardly any restrictions on the linking matrices you can get from a quasipositive link. For example, this construction shows that everything except the first row and column of the linking matrix can be completely arbitrary:

Start with any link $L$, represent it as the closure of a braid, and then draw the braid with horizontal and vertical segments so that horizontal segments always pass over vertical segments. Then a typical small piece of the braid will look like this:

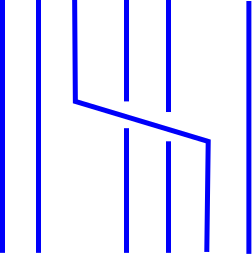
To this picture, add one new strand that generally runs along the left edge of the braid, but near a horizontal segment does something like this:

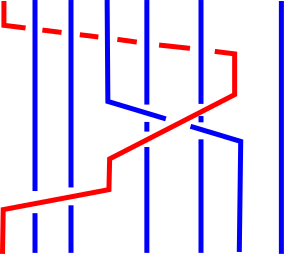
Then the resulting braid is strongly quasipositive, and its closure is a link $L' = L \cup U$ consisting of $L$ together with an unknot winding around it. Applying this construction to the negative Hopf link gives something close to your link, except the extra strand only needs to wind around twice.

The linking matrices coming from this construction always have nonnegative row sums, even if the entries can be very negative, and I think it should be possible to show that the same holds for any strongly quasipositive link, using the fact that a strand of a quasipositive braid can only participate in negative crossings while it's moving to the right. However, this doesn't work for non-strongly quasipositive links. This two-component link is the closure of a quasipositive braid, but has linking number $-1$:

Answer 2

Any linking matrix appears as linking matrix of a strongly quasipositive link.

This follows from a result of Rudolph ("Constructions of Quasipositive Knots and Links, I") that any Seifert matrix appears as Seifert matrix of a strongly quasipositive link.