I am reading the famous paper "Unitary Representations of the Virasoro and Super-Virasoro Algebras" by Goddard, Kent, Olive.
From a compact simple Lie algebra $\mathfrak{g}$ and a Lie subalgebra $\mathfrak{h}$, they obtain a representation $Vir(\mathfrak{g},\mathfrak{h})$ of the Virasoro algebra. The unitary highest weight irreducible representations of a Virasoro algebra are labelled by $(c,h)$, with $c$ the central charge and $h$ the highest weight. In the paper, they show that $c$ can take any value in the series
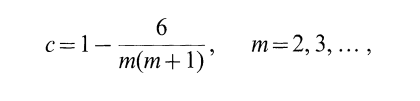
In a second moment, they show that $h$ can take any value in the series

They prove this last result using character theory. But what I do not understand is the idea behind this last proof. They start it with the following paragraph:

In particular, I do not understand how to make sense of the highlighted sentence: what do they mean with "decompose with respect to" in this context; how such decomposition helps us at all; and how exactly does (2.20) come to be.
