Let $G$ be a split semisimple real Lie group in characteristic zero, and let $B=TU$ be a Borel subgroup with unipotent radical $U$ and Levi $T$. Fix an ordering on the roots $\Phi^+$ of $T$ in $U$, and for each root subgroup $U_{\alpha}$ of $U$, let $u_{\alpha}: \mathbb R \rightarrow U_{\alpha}$ be an isomorphism.
For all $\alpha, \beta \in \Phi^+$, there exist unique real numbers $C_{\alpha,\beta,i,j}$ (depending on the $u_{\alpha}$ and the ordering) such that for all $x, y \in \mathbb R$,
$$u_{\alpha}(x) u_{\beta}(y) u_{\alpha}(x)^{-1} = u_{\beta}(y) \prod\limits_{\substack{i,j>0\\ i\alpha + j \beta \in \Phi^+}} u_{i\alpha+j\beta}(C_{\alpha,\beta,i,j}x^iy^j)$$
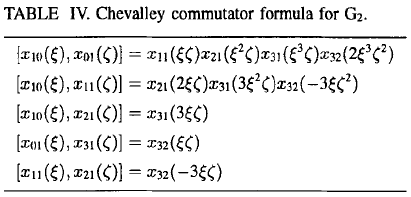
I want to work out some examples with unipotent groups of exceptional semisimple groups, and am looking for table of structure constants for the root system G2. Does anyone know a reference where an ordering on the roots is chosen and these constants are written down?