6
$\begingroup$

The question concerns the group homology or group cohomology of symmetric groups.

  1. The entries in groupprops.subwiki.org and in this MO post show the results for the symmetric group S$_4$.

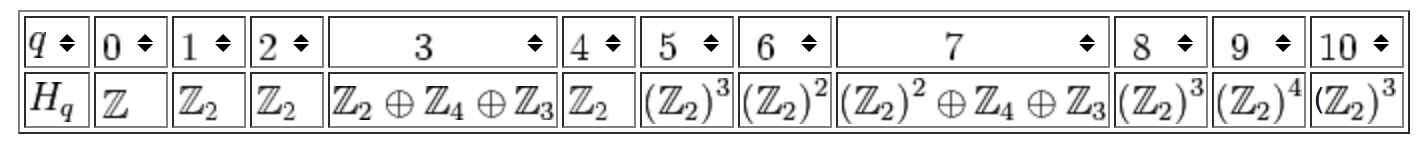
groupprops.subwiki.org, $H_q(\text{S}_4,\mathbb{Z})$: enter image description here

this MO post, $H_k(\text{S}_{n=4},\mathbb{Z})$: enter image description here

  1. If the groupprops.subwiki.org has the correct result, is it correct to say that the torsion parts of Group (Co)Homology of Symmetric Group are related by:

$$H^q(\text{S}_4,\mathbb{R}/\mathbb{Z})=H_q(\text{S}_4,\mathbb{Z})?$$

Thus, $$H^1(\text{S}_4,\mathbb{R}/\mathbb{Z})=H_1(\text{S}_4,\mathbb{Z})=\mathbb{Z}_2?$$ $$H^2(\text{S}_4,\mathbb{R}/\mathbb{Z})=H_2(\text{S}_4,\mathbb{Z})=\mathbb{Z}_2?$$ $$H^3(\text{S}_4,\mathbb{R}/\mathbb{Z})=H_3(\text{S}_4,\mathbb{Z})=\mathbb{Z}_2\oplus \mathbb{Z}_4\oplus \mathbb{Z}_3?$$ $$H^4(\text{S}_4,\mathbb{R}/\mathbb{Z})=H_4(\text{S}_4,\mathbb{Z})=\mathbb{Z}_2?$$

  1. More generally, do we have a precise relation between the group cohomology and group homology of different coefficients:

$$H^q(G,\mathbb{R}/\mathbb{Z}) \text { and } H_p(G,\mathbb{Z}),$$ say for any finite group $G$, and here in particular $\text{S}_4$? (Here $\mathbb{R}/\mathbb{Z}=S^1=$U(1).)

Improve this question
$\endgroup$
7
  • $\begingroup$ + 1, Jeremy Rickard, thanks for reminding me. $\endgroup$
    – wonderich
    Commented Apr 8, 2018 at 17:48
  • 2
    $\begingroup$ Since symmetric groups are finite groups, its (co)homology is entirely torison (except in $H_0$. $\endgroup$
    – user43326
    Commented Apr 8, 2018 at 17:50
  • 2
    $\begingroup$ Just in case my previous comment was not clear enough: $R/Z$ is injective, so in the universal coefficient theorem's statement, there is no $Ext ^1$ term, so you only get $Hom$ term. However, for any torsion abelian group $A$ you have $Hom(A,R/Z)\cong A$ in a non-canonical way. $\endgroup$
    – user43326
    Commented Apr 8, 2018 at 18:33
  • $\begingroup$ @ user43326, Thanks so much +1. then, am I correct about my statements in part 2, after all? How about the part 3? [Feel free to write an answer - it will not have to be demanding one.] $\endgroup$
    – wonderich
    Commented Apr 8, 2018 at 19:14
  • $\begingroup$ @user43326 it's false (that $Hom(A,R/Z)$ is isomorphic to $A$ for $A$ torsion abelian). Two counterexamples (among others) (a) if $A=\bigoplus A_n$ with $A_n\neq 0$ finite, then $A$ is countable, while $Hom(A,R/Z)\simeq\prod_n A_n$, which is uncountable. (b) if $A=\mathbf{Z}[1/n]/\mathbf{Z}$, then $Hom(A,R/Z)\simeq\mathbf{Z}_n$, which is torsion-free (and also uncountable). Actually when $A$ is infinite countable, it's always uncountable. $\endgroup$
    – YCor
    Commented Apr 8, 2018 at 22:04

1 Answer 1

Reset to default
5
$\begingroup$

First of all, let $X$ be a space (with usual nice properties). Then as ${\mathbb Q}/{\mathbb Z}$ is injective, the universal coefficient theorem for cohomology gives an isomorphism $$Hom (H_*(X,{\mathbb Z}) ,{\mathbb Q}/{\mathbb Z}) \cong H^*(X,{\mathbb Q}/{\mathbb Z}). $$ Now, when $X$ is the classifying space of a finite group $BG$, in positive dimension, $A=H_*(X,{\mathbb Z})$ is finite abelian group, thus there is non-canonical isomorphism between $A$ and $Hom(A,{\mathbb Q}/{\mathbb Z})$.

Thus the answer to the third question (which includes the second one) is "yes".

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .