4
$\begingroup$

Let $I$ be a closed interval in $\mathbb{R}$, and let $f:I \to \mathbb{R}$ be a bounded function, smooth except at finitely many points (piece-wise smooth). There are also only finitely many critical points, except that some of the pieces may be horizontal lines. [Edit: at any rate, I'm assuming $f$ has finitely many critical values in the codomain.] Let $$G = \operatorname{closure}\big(\{(x,y) \in I \times \mathbb{R} ~\big|~ y \leq f(x)\}\big)$$ denote the closed region under the graph of $f$. For each $s \in \mathbb{R}$, let $n(s)$ denote the number of connected components of the intersection of $G$ with the line $y=s$. In other words, the number of connected components of $\{(x,s) ~\big|~ s \leq f(x)\}.$

I would like a reference the following, which my intuition says is true and not difficult from the right perspective:

The function $n:\mathbb{R} \to \{0,1,2,3,\dots\}$ is continuous from below. That is, for any $s \in \mathbb{R}$, there exists $\varepsilon >0$ such that $n$ is constant on $(s-\varepsilon,s]$.

Here's a poor picture: 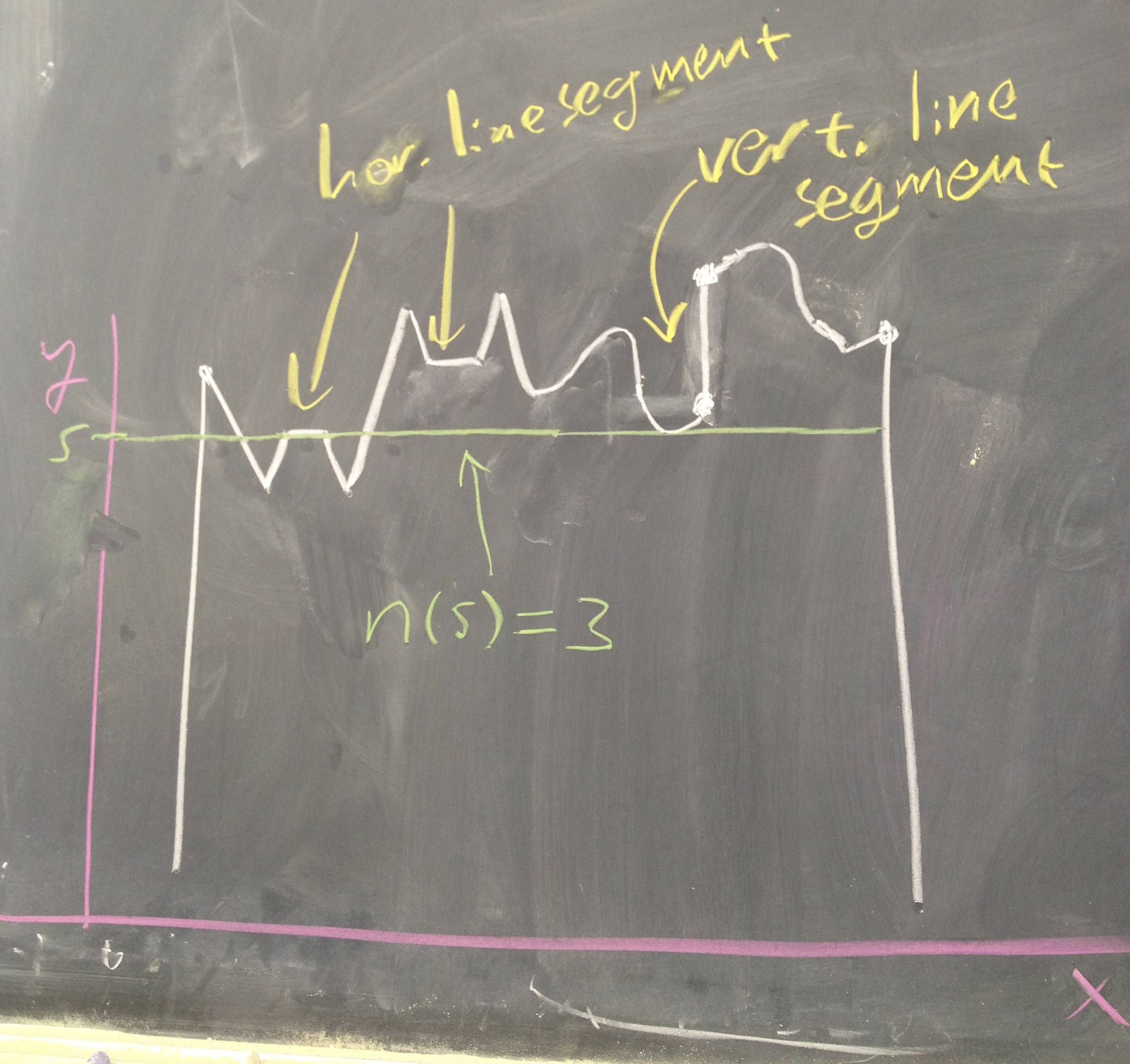enter image description here (The vertical line segment in the boundary is at a place where $f$ had a discontinuity.)

A little context. This seems like a really elementary result (unless I'm dense and it's false as stated), but from an area that I'm not as comfortable with. I want to say it's like Morse theory for a 2-manifold with piece-wise smooth boundary in $\mathbb{R}^2$? But I'm not looking for a sledgehammer. I'm a number theorist, and this will be used in a number theory paper, and I guess that people reading (refereeing?) the paper might like a reference for this fact, even if it might be stated without proof or reference in papers in other fields. If you have a super short proof that would make sense to someone outside the area, that would be fine, too, of course.

Please help me improve this question if I've stated it poorly or tagged it inappropriately!

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

Suppose there were some $s\in\mathbb R$ such that $n$ is not continuous from below. Then, for all $\epsilon>0$, there is some $x\in(s-\epsilon,s]$ that is a critical value of $f$, i.e., $x$ is either a local maximum, local minimum, or the height of a horizontal segment. This implies that $f$ has infinitely many such critical values, which implies that $f$ has infinitely many critical points: a contradiction of the hypothesis.

More succinctly, the claim is clearly true at an isolated critical value, which is enough, since there are finitely many critical values.

Improve this answer
$\endgroup$
3
  • $\begingroup$ So what it seems like is the case is that you get continuity from below at any critical value which is not a local minimum (or horizontal line segment which is basically a fat local minimum) of $G$ (I'm not talking about a local minimum of the graph of $f$), and since $G$ is the region below the graph of a function, there is no local minimum -- but I'm not sure the best way to make this statement precise. $\endgroup$
    – Bobby Grizzard
    Commented Apr 14, 2016 at 21:12
  • $\begingroup$ I'm defining a local minimum of a subset $\Omega$ of $\mathbb{R}^2$ as a point $P$ such that there is an open neighborhood of $P$ containing no points of $\Omega$ having lower $y$-coordinate. $\endgroup$
    – Bobby Grizzard
    Commented Apr 14, 2016 at 21:39
  • $\begingroup$ If you flip the argument upside down, or let $G$ be the area above the graph, then we see that $n$ will be continuous from above at all of the critical points. Of course, $n$ cannot be both continuous from above and below, or it would be constant. So, if $\Omega$ is a general compact region of the plane, then $n$ will be continuous from below when exiting $\Omega$ and continuous from above when entering $\Omega$. This is a reflection that you are choosing to think of $\Omega$ as closed and its complement as open, and not vise versa. $\endgroup$
    – Jeffrey Meier
    Commented Apr 15, 2016 at 0:06

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .