Here are some comments, including an answer to (3). Firstly, if you want an actual explicit computation of the mod-2 cohomology of symmetric groups $S_n$ for as large an $n$ as possible, you should look at M. Feshbach's `The mod-2 cohomology rings of the symmetric groups and invariants' Topology volume 41 (2002) 57-84. This contains explicit computations of the cohomology rings $H^*(S_n;\mathbb{F}_2)$ for $n\leq 16$, including correcting a minor error in a calculation in the book of A Adem and J Milgram.
The advantage of cohomology is that it is a ring, also that the cohomology of a finite group with coefficients in either $\mathbb{Z}$ or a field is a finitely presented algebra over the coefficients.
Here is a non-constructive solution to (3). By the universal coefficient theorem, it suffices to show the same thing for cohomology. Now let $n$ be sufficiently large that the symmetric group $S_n$ contains a cyclic subgroup of order $p^k$; of course the least such $n$ is $n=p^k$. For any such $n$, there will be elements of order $p^k$ in $H^j(S_n;\mathbb{Z})$ for infinitely many values of $j$. To see this one uses the Evens-Venkov theorem.
For any finite group $G$ and any subgroup $H$, the map from $H^*(G;\mathbb{Z})$ to $H^*(H;\mathbb{Z})$ makes the ring $H^*(H;\mathbb{Z})$ into a module for the ring $H^*(G;\mathbb{Z})$. The Evens-Venkov theorem tells us that $H^*(H;\mathbb{Z})$ is finitely generated as an $H^*(G;\mathbb{Z})$-module.
Now to apply this. The cohomology ring of the cyclic group of order $p^k$ is isomorphic to a polynomial ring $\mathbb{Z}[c]/(p^kc)$, where $c$ is a generator for $H^2$. If $R$ is a (graded) subring of this ring such that the whole ring is a finitely generated $R$-module, then $R$ contains $c^m$ for some $m$, and hence $H^{2mj}(S_n;\mathbb{Z})$ contains an element of order $p^k$ for every $j$. The universal coefficient theorem then tells you that $H_{2mj-1}(S_n;\mathbb{Z})$ contains an element of order $p^k$ for all $j$.
You asked about summands of order exactly $p^k$. In fact, $H^*(S_n;\mathbb{Z})$ will contain these whenever $n\geq p^k$, although I can't provide a quick argument. A quicker thing to see is that for $p^k \leq n < p^{k+1}$, the exponent of the $p$-local cohomology of $S_n$ is exactly $p^k$. I'll give the argument just for $n=p^k$. There is a subgroup of $S_{p^k}$ isomorphic to the direct product of $p$ copies of $S_{p^{k-1}}$. Furthermore, the index of this subgroup is divisible by $p$ but not divisible by $p^2$.
For any finite group $G$ and subgroup $H$, there is a transfer map in cohomology $H^*(H;\mathbb{Z})\rightarrow H^*(G;\mathbb{Z})$ with the property that the composite map from $H^*(G;\mathbb{Z})$ to itself given by first mapping to $H^*(H;\mathbb{Z})$ and then transferring back up is equal to multiplication by the index $|G:H|$.
Going back to the symmetric group, we know by induction on $k$ that the exponent of the $p$-part of $H^*(S_{p^{k-1}};\mathbb{Z})$ is $p^{k-1}$, and by the Kunneth formula the same holds true for the direct product of $p$ copies of this group. The restriction from $S_{p^k}$ down to this subgroup, followed by the transfer map back up is, up to units, multiplication by $p$ on the $p$-local cohomology $H^*(S_{p^k};\mathbb{Z}_{(p)})$. Hence the exponent of this group is at most $p^k$. Combined with the Evens-Venkov lower bound this gives the claim.
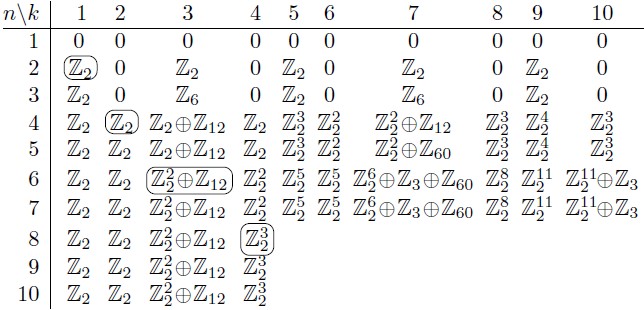 This seems to be an infinite amount of data with no apparent patterns, just the stabilization for $n\geq2k$.
This seems to be an infinite amount of data with no apparent patterns, just the stabilization for $n\geq2k$.