Impossible Range for Minkowski-Like Sum of Squares

Question

Given coprime positive integers M,N, and a corresponding integer z outside of the range (for all integers x,a,b,c) of $Mx^2-N(a^2+b^2+c^2)$, is there any such z which is "deceptive", meaning that it is modularly inside the range of the smaller components, i.e., inside the range of both $Mx^2$ mod N and $-N(a^2+b^2+c^2)$ mod M? Feel free to replace $a^2+b^2+c^2$ with "All integers except for $4^a(8b+7)$" per Lagrange.

Answer 1

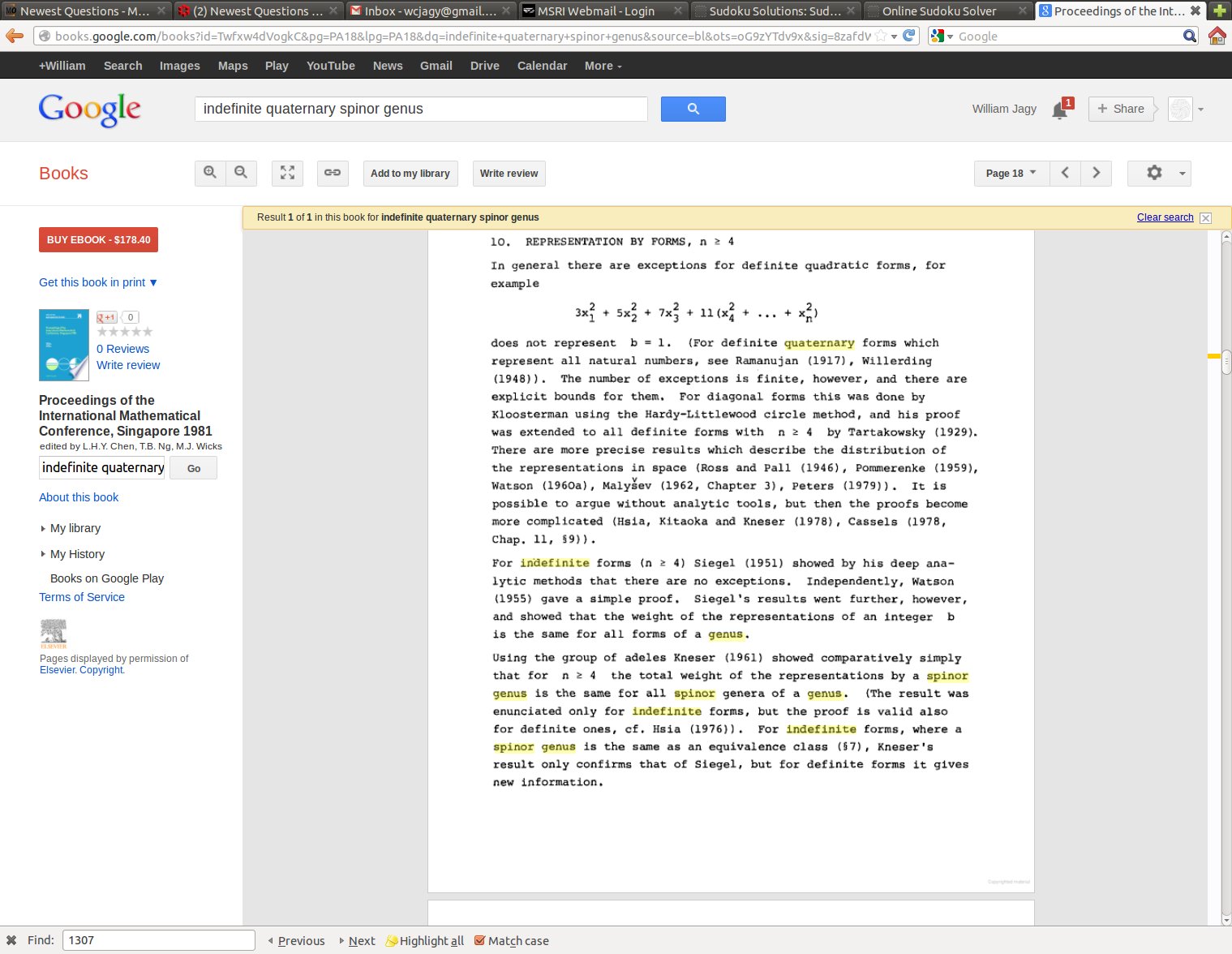
There are no deceptive integers for indefinite forms in at least 4 variables, this begins with Siegel (1951). I have the 1961 article by Martin Kneser, it is famous for a variety of reasons. This article is by J. W.S. Cassels, presented in the International Congress in Singapore in 1981, the article is pages 9-26 in the book. Below is page 18, then page 19.

Watson 1955 is Representations of integers by indefinite quadratic forms, Mathematika, volume 2, pages 32-38. I think it is covered on page 114 of his 1960 book, but he is maddeningly terse.

The first examples of deceptive integers were found by Jones and Pall in 1939, see at TERNARY. The shortest one to write would be $$ 3 x^2 + 4 y^2 + 9 z^2 \neq w^2, $$ whenever all (positive) prime factors of $w$ are congruent to $1 \pmod 3.$

Let me give a proof for Siegel's 1951 indefinite ternary example. The main lemma from binary forms is that, given a (positive) prime $q \equiv \pm 3 \pmod 8,$ if $q | (a^2 - 2 b^2),$ then $q|a,b,$ and in fact $q^2 | (a^2-2 b^2).$ By induction there is some $l$ such that $q^{2l} \parallel (a^2 - 2 b^2).$ The double vertical bar means that we are showing the highest exponent of the prime such that the indicated power still divides the expression on the other side.

Now, suppose we have some $t$ such that all (positive) prime factors $p$ of $t$ satisfy $p \equiv \pm 1 \pmod 8.$ We will prove that $(2x+z)^2 - 2 y^2 + 16 z^2 \neq t^2. $ For, if we had $(2x+z)^2 - 2 y^2 + 16 z^2 = t^2, $ we would need to have odd $z$ because $t$ is odd. Next $$(2x+z)^2 - 2 y^2 = t^2 - 16 z^2 = (t+4z)(t-4z). $$ Both $t+4z, \; t- 4 z \equiv \pm 3 \pmod 8$ because $z$ is odd. There is some (positive) prime $q \equiv \pm 3 \pmod 8$ with $q^{2j+1} \parallel t+4z.$ However, from the earlier lemma, as $q^{2l} \parallel ( (2x+z)^2 - 2 y^2),$ we find that $q^{2k+1} \parallel (t-4z).$ That is, $q | t+4z$ and $q | t - 4 z,$ so that $q | t,$ which contradicts the hypotheses on $t.$

To show explicitly that Siegel's second form does represent all $q^2,$ for prime $q \equiv \pm 3 \pmod 8.$ Start with $$ \left( \alpha^2 - 2 \beta^2 + 4 \gamma^2 \right)^2 = \left( \alpha^2 + 2 \beta^2 - 4 \gamma^2 \right)^2 - 2 \left( 2 \alpha \beta \right)^2 + 4 \left( 2 \alpha \gamma \right)^2$$

For $q \equiv 3 \pmod 8,$ we may take $\gamma = \beta, \; \; q = \alpha^2 + 2 \beta^2$ with both $\alpha,\beta$ odd, then $$ q^2 = \left( \alpha^2 - 2 \beta^2 \right)^2 - 2 \left( 2 \alpha \beta \right)^2 + 16 \left( \alpha \beta \right)^2 $$ with both $\alpha^2 - 2 \beta^2$ and $ \alpha \beta$ odd. So, we have written $$ q^2 = \zeta^2 - 2 \eta^2 + 16 \theta^2, \; \; \zeta \equiv \theta \pmod 2. $$

For $q \equiv 5 \pmod 8,$ we may take $ \beta = 0, \; \; q = \alpha^2 + 4 \gamma^2$ with both $\alpha,\gamma$ odd, then $$ q^2 = \left( \alpha^2 - 4 \gamma^2 \right)^2 - 2 \left( 0 \right)^2 + 16 \left( \alpha \gamma \right)^2 $$ with both $\alpha^2 - 4 \gamma^2$ and $ \alpha \gamma$ odd. So, we have written $$ q^2 = \zeta^2 - 2 \eta^2 + 16 \theta^2, \; \; \zeta \equiv \theta \pmod 2. $$

In either case, we may write $q^2 = (2x+z)^2 - 2 y^2 + 16 z^2$ with $$ x = (\zeta - \theta)/2, \; \; y = \eta, \; \; z = \theta. $$

On the other hand, Siegel's two forms agree on all other numbers except the specific squares mentioned. On page 19 below, Cassels is mostly talking about primitive representations, which has its own distinctions.

==================

=================

==================

Answer 2

As requested, a diagonal example.

I found this as Example 1.2 on page 324 of Representations by Spinor Genera of Ternary Quadratic Forms, by Rainer Schulze-Pillot and Fei Xu, pages 323-337 in a book. The book is Algebraic and Arithmetic Theory of Quadratic Forms, volume 344 in the Contemporary Mathematics series of the AMS, published 2004, edited by Ricardo Baeza, John S. Hsia, Bill Jacob, and Alexander Prestel.

The two forms $$ x^2 - 5 y^2 + 100 z^2 $$ and $$ 4 x^2 - 5 y^2 + 25 z^2 $$ are in the same genus but are distinct because $$ 4 x^2 - 5 y^2 + 25 z^2 \neq \varphi^2, $$ where all (positive) prime factors $p$ of $\varphi$ satisfy $$ p \equiv \pm 1 \pmod 5. $$ I picked $\varphi$ because it has an f sound that goes well with 5.

Assume $$ - 5 (y^2 - 5 z^2) = \varphi^2 - 4 x^2. $$ So $x$ is not divisible by 5. Furthermore, $\varphi^2 \equiv 1 \pmod 5.$ Therefore $x^2 \equiv 4 \pmod 5$ and $x \equiv \pm 2 \pmod 5. $ Next $4x \equiv \pm 2 \pmod 5. $ So, while one of $\varphi + 2 x, \varphi - 2 x $ is divisible by 5, the other one is congruent to $\pm 2 \pmod 5.$ Then, there is some odd prime $$ q \equiv \pm 2 \pmod 5 $$ such that $$ q^{2l+1} \parallel \varphi + 2 x, $$ say, having chosen $\pm x$ to arrange it this way. However, we know $$ q^{2k} \parallel y^2 - 5 z^2. $$ Therefore $$ q^{2j+1} \parallel \varphi - 2 x, $$ finally $$ q | 2 \varphi$$ and $q | \varphi,$ which is a contradiction.

NOTE: the positive binary forms $x^2 + 100 z^2$ and $4 x^2 + 25 z^2$ are in the same genus. In the sense of Estes and Pall, they are not in the same spinor genus, as $4 x^2 + 25 z^2$ is not a fourth power in the class group.