3
$\begingroup$

This question was posted in MSE but is still open hence posting in MO.

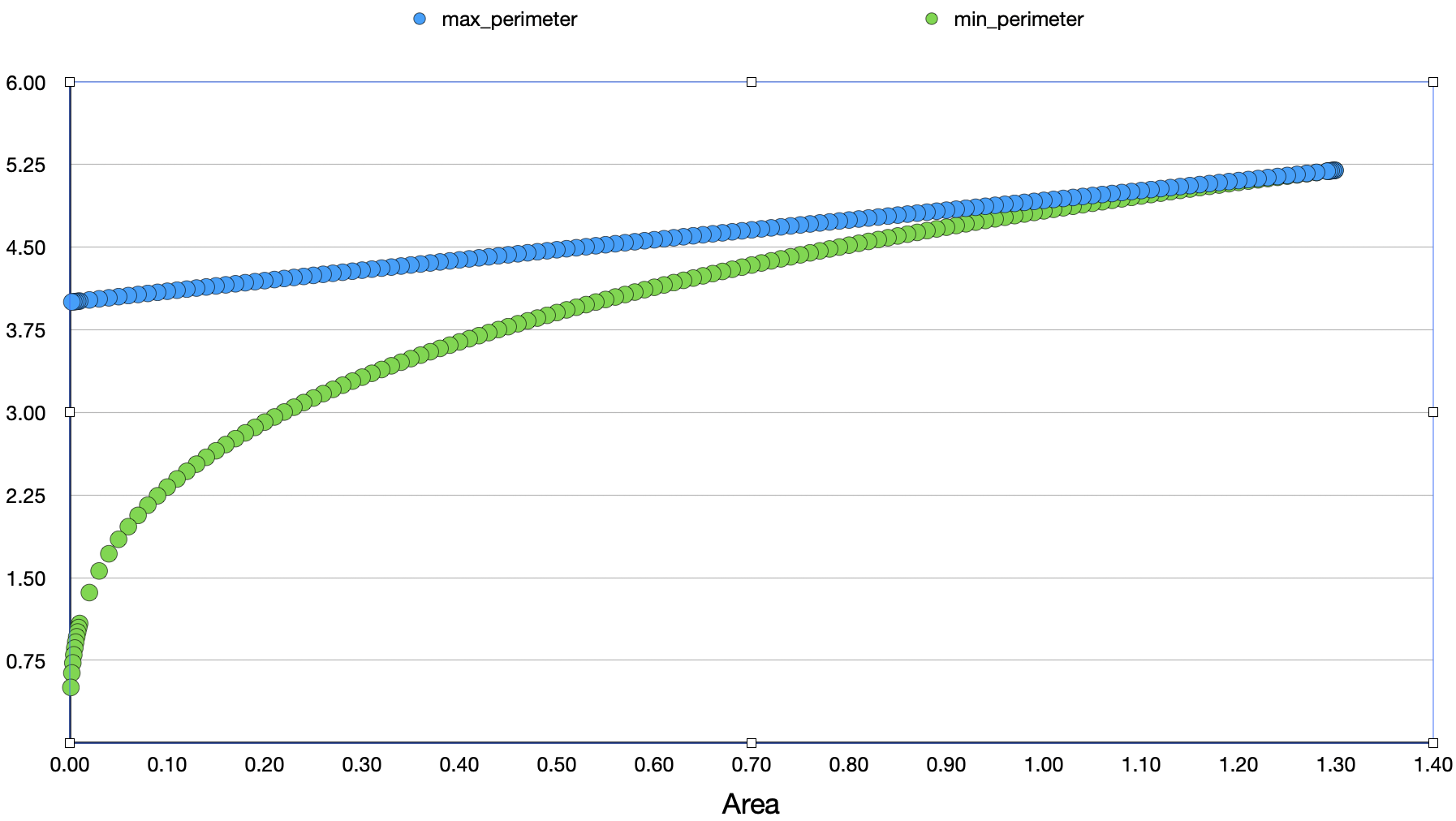
The area of the largest triangle that can be inscribed in a circle of raidus $1$ is $\displaystyle \frac{3 \sqrt{3}}{4}$ for a equilateral triangle and it also gives the perimeter is $3\sqrt{3}$. For any $\displaystyle 0 \le x \le \frac{3 \sqrt{3}}{4}$ we can ask the minimum perimeter $f(x) $and maximum perimeter $g(x)$ of triangles with area $x$ inscribed in a circle of radius $1$. I ran a simulation to calculate the values of $f(x)$ and $g(x)$ for all $x$ in this interval and obtained the plot below.

enter image description here

For the maximum perimeter, trivially $g(0) = 4$ and $\displaystyle g\left(\frac{3 \sqrt{3}}{4}\right) = 3 \sqrt{3}$. The graph of $g(x)$ appears to be a straight line as shown by the blue line. Fitting a straight line through these two points gives the model

$$ g(x) = 4 + \left(4 - \frac{16}{3\sqrt{3}}\right)x \tag 1 $$

Can this be proven? For the minimum perimeter we have trivially $f(0) = 0$ and $\displaystyle f\left(\frac{3 \sqrt{3}}{4}\right) = 3 \sqrt{3}$ and $f(1) = 2+2\sqrt{2}$ corresponding to an isosceles right triangle. Fitting a curve with the data for $g(x)$ suggests a model of the form $f(x) = (2+\sqrt{2})x^a$ with $a \approx 0.306$ however this model with inconsistent with the fact that $\displaystyle f\left(\frac{3 \sqrt{3}}{4}\right) = 3 \sqrt{3}$ hence this model was discarded.

Question: What is the minimum and the maximum perimeter of a triangle with area $x$ and circumradius $1$?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
4
$\begingroup$

The extrema are attained for symmetric (isosceles) triangles. Trigonometry gives for the pairs (area, perimeter) as a function of the half tip angle $\psi$ the relations $$\left( 4 \,\sin \psi\,\cos^3\psi, 4\, \cos\psi\,(1+\sin \psi) \right).$$

If this is plotted for $\psi \in [0,\pi/2)$ one obtains exactly your graph. The blue upper curve corresponds to $\psi \in [0,\pi/6)$ and is by no means a straight line. Here is a Mathematica code for the plot:

Table[4 {Sin[p] Cos[p]^3, Cos[p] (1 + Sin[p]) }, {p, 0, Pi/2, Pi/200}] // ListPlot

Improve this answer
$\endgroup$
6
  • 2
    $\begingroup$ "The extrema are attained for symmetric (isosceles) triangles." -- Why? $\endgroup$
    – Iosif Pinelis
    Commented Dec 1, 2023 at 15:42
  • 1
    $\begingroup$ @ Iosif Pinelis: I expected this question... A full argument is not easy, but at least area and perimeter are invariant under reflection $(a,b,c) \to (b,a,c)$ so the symmetric states are extremal. There also is an answer to the MSE post which claims to have shown this. $\endgroup$
    – Karl Fabian
    Commented Dec 1, 2023 at 15:59
  • 2
    $\begingroup$ I am not quite sure what reflection you are referring to here. Anyhow, how does the invariance imply the extremality? Also, I understand that a complete argument is not easy, but do you have it? At least, do you have an idea of a proof? $\endgroup$
    – Iosif Pinelis
    Commented Dec 1, 2023 at 16:26
  • 1
    $\begingroup$ @Iosif Pinelis: Here is a sketch for the first part: If $(a,b,c)$ are the sides of the triangle in positive order, then the triangle $(b,a,c)$ has the same circumcircle, area and perimeter. For arbitrarily small $\epsilon>0$ there is a constant $C$, such if you have a triangle $(a,a',c')$ near $(a,a,c)$ with the same area $x$, and $|a-a'|<\epsilon$, $|b-b'|<\delta< C\,\epsilon$, then $(a',a,c)$ has the same circumcircle, area and perimeter, and is equally close. For $\epsilon \to 0$ it follows that $(a,a,c)$ has extremal perimeter. The second part (see MSE) needs heavier trigonometry. $\endgroup$
    – Karl Fabian
    Commented Dec 1, 2023 at 20:34
  • 1
    $\begingroup$ I do not see how you get the extremality here. $\endgroup$
    – Iosif Pinelis
    Commented Dec 1, 2023 at 20:51

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .