Are there infinitely many "generalized triangle vertices"?

Question

Briefly, I'd like to know whether there are infinitely many "generalized triangle centers" which - like the orthocenter - are indistinguishable from a vertex of the original triangle. This is basically a refinement of this MSE question of mine; note that the type of "generalized triangle center" I'm interested in is not the standard one (see 1,2), although I would also be interested in the situation for that definition.

Separately, since MO bounties have time limits, I'll "informally bounty" this question: I'll reward the first complete answer to the question with a 1000 point bounty, whenever - if ever - that should happen.

---

Definitions

Let $\mathbb{T}$ be the set of noncollinear ordered triples of points in $\mathbb{R}^2$. Say that a topological triangle center representative (ttcr) is a function $t:G\rightarrow \mathbb{R}^2$ such that:

• $G$ is a connected dense open subset of $\mathbb{T}$ and $t$ is continuous;

• $G$ and $t$ are each symmetric: if $(a,b,c)\in G$, then $(a,c,b)$ and $(b,a,c)$ are in $G$ as well and we have $t(a,b,c)=t(a,c,b)=t(b,a,c)$;

• both $G$ and $t$ are homothety-etc.-invariant: if $\alpha:\mathbb{R}^2\rightarrow\mathbb{R}^2$ is a composition of rotations, reflections, translations, and homotheties, and $(a,b,c)\in G$, then $(\alpha(a),\alpha(b),\alpha(c))\in G$ and $t(\alpha(a),\alpha(b),\alpha(c))=\alpha(t(a,b,c))$.

• and $t$ is (usually) iterable: for a dense open $H\subseteq G$, if $(a,b,c)\in H$ then $(t(a,b,c),b,c)\in H$.

Each classical triangle center that I'm familiar with corresponds to a ttcr, possibly after tweaking the domain. For instance, in the case of the orthocenter we need to throw out right triangles to satisfy the iterability requirement.

A topological triangle center is then an equivalence class of ttcrs with respect to the relation $t\sim s\iff t_{\upharpoonright \operatorname{dom}(t) \cap \operatorname{dom}(s)}=s_{\upharpoonright \operatorname{dom}(t)\cap \operatorname{dom}(s)}$. Finally, a pseudovertex is a topological triangle center with a representative $t$ satisfying $$t(t(a,b,c),b,c)=a$$ for every $(a,b,c)\in \operatorname{dom}(t)$.

---

Question

My question is simply, how many pseudovertices are there? Specifically:

Are there infinitely many pseudovertices?

I strongly suspect that the answer is yes (indeed that there should be continuum-many due to the existence of at least one not-too-interesting continuously-parameterized family), and I suspect that in fact there is an easy proof of this fact, but I can't see it at the moment.

So far I know of three distinct pseudovertices (modulo appropriate definitional abuse):

• The orthocenter, $X(4)$.

• The isogonal conjugate of the Euler infinity point, $X(74)$.

• The isogonal conjugate of Parry's reflection point, $X(1138)$.

As a curiosity, note that these three centers are nontrivially related to each other: it turns out that $X(74)$ is the crosspoint of $X(4)$ and $X(1138)$. This fact, as well as the examples of $X(74)$ and $X(1138)$, was found by MSE user Blue at the above-linked question.

Answer 1

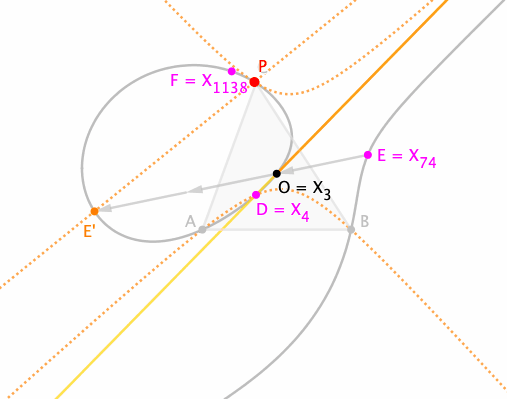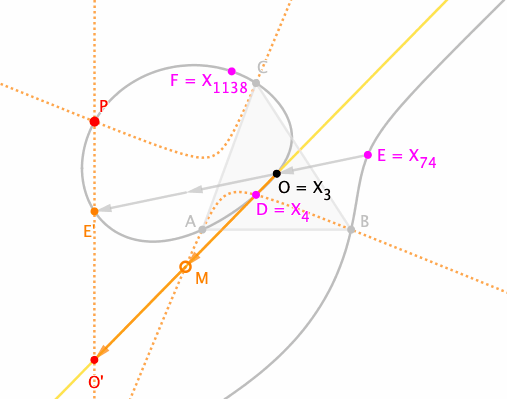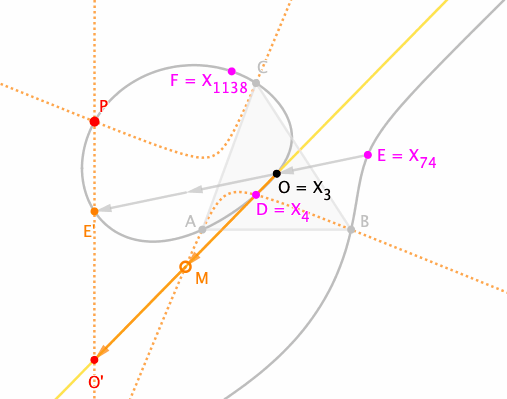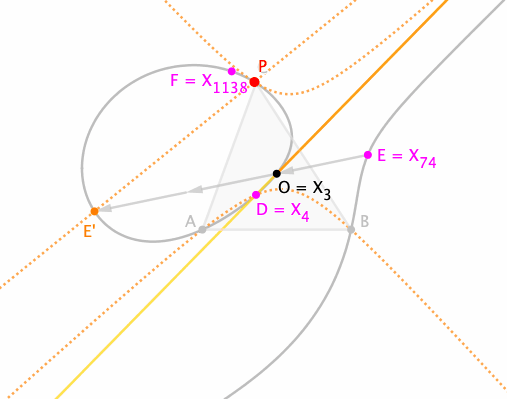
There are uncountably many pseudovertices. Specifically, I will show that starting with the pseudovertex $X(4)$, we can make a small perturbation to a certain subset of the domain, propogate this perturbation to the rest of the domain, and the result will still be a pseudovertex. The construction allows us to make these perturbations $C^\infty$ in the coordinates of the vertices, or piecewise rational in the coordinates, and still obtain uncountably many distinct pseudovertices. The pseudovertices $X(4)$, $X(74)$, and $X(1138)$ are particularly nice in that they can be expressed as rational functions in the coordinates; it's still open whether there are any other "algebraic" pseudovertices of this form.

Setup

Define $f,g,h:(\mathbb{C}\setminus\mathbb{R})\to (\mathbb{C}\setminus\mathbb{R})$ by $$f(z):=1-z,\qquad g(z):=z^{-1},\qquad h(z):=\overline{z}.$$ Let $\Gamma:=\langle f,g,h\rangle$; by checking the relations $$f^2=g^2=h^2=(fh)^2=(gh)^2=(fg)^3=1,$$ we conclude $\Gamma\simeq S_3\times C_2$ (with $(1\,2)\mapsto f$, $(2\,3)\mapsto g$, and $C_2\to\langle h\rangle$). Given a non-colinear triple $(a,b,c)$ of points in $\mathbb{C}$, there is a unique "homothety-etc" on $\mathbb{C}$ taking $b\mapsto 0$, $c\mapsto 1$, and $a$ into the upper half-plane. Note that if $(a,b,c)\mapsto z$ then $(c,b,a)\mapsto \overline{z}^{-1}$ and $(a,c,b)\mapsto 1-\overline{z}$. Thus there is a one-to-one correspondence between triangles (i.e. unordered triples) up to homothety-etc and $\Gamma$-orbits of $(\mathbb{C}\setminus\mathbb{R})$. The figure below illustrates how to partition $\mathbb{C}\setminus\mathbb{R}$ into twelve fundamental domains that are sent to each other by $\Gamma$.

Following Peter Taylor's answer, we can start with a pseudovertex and define a dense open $U\subseteq (\mathbb{C}\setminus\mathbb{R})$ that is invariant under $\Gamma$, and a continuous bijection $\mu:U\to U$ such that for all $z\in U$, $$\mu(1-z)=1-\mu(z),\qquad\mu(z^{-1})=z^{-1}\mu(z),\qquad\mu(\overline{z})=\overline{\mu(z)},\qquad \mu(\mu(z))=z.$$ We'll call these the "pseudovertex relations." Conversely, given any $\Gamma$-invariant subset $U\subseteq\mathbb{C}$ and continuous bijection $\mu:U\to U$ satisfying the pseudovertex relations for all $z\in U$, we can define a "partial pseudovertex" (a function satisfying all the axioms of a pseudovertex other than possibly the domain being connected dense open): bijectivity ensures iterability, $\mu(\overline{z})=\overline{\mu(z)}$ ensures reflection-invariance, the first two relations ensure symmetry, and the last relation ensures $t(t(a,b,c),b,c)=a$.

The action of a pseudovertex

Given a $\Gamma$-invariant subset $U\subseteq\mathbb{C}\setminus\mathbb{R}$ and a bijection $\mu:U\to U$, we can consider the group $\Gamma[\mu]:=\langle f,g,h,\mu\rangle$ of functions on $U$.

Lemma A: Suppose $\mu$ satisfies the pseudovertex relations. Then $\Gamma[\mu]\simeq S_4\times C_2$.

Proof: In addition to the relations between $f,g,h$ established above, the pseudovertex relations imply $(\mu f)^2=(\mu h)^2=\mu^2=1$. Now using the pseudovertex relation $(\mu\circ g)(z)=z^{-1}\mu(z)$, we obtain $$(\mu\circ g)^2(z)=(\mu\circ g)(z)^{-1}\mu((\mu\circ g)(z))=z\mu(z)^{-1}\mu(\mu(z^{-1}))=\mu(z)^{-1}.$$ Likewise, $$(\mu\circ g)^3(z)=(\mu\circ g)^2(z)^{-1}\mu((\mu\circ g)^2(z))=\mu(z)(\mu\circ g)(z)^{-1}=z.$$ Thus we have a well-defined homomorphism from $S_4\times C_2$ given by $(1\,2)\mapsto f$, $(2\,3)\mapsto g$, $(3\,4)\mapsto \mu$, and the generator of $C_2$ to $h$.

We can now ask whether this homomorphism has a kernel. Note that $S_3\times C_2$ maps bijectively onto $\Gamma$, so the intersection of the kernel with this subgroup must be trivial. By the classification of normal subgroups of $S_4$, the only possible nontrivial kernel is the normal $V_4\leq S_4$. But $(1\,2)(3\,4)\in V_4$ maps to $f\circ\mu$, and for this to be the identity we must have $\mu(z)=1-z$. This contradicts $\mu(z^{-1})=z^{-1}\mu(z)$. So the homomorphism is an isomorphism. $\square$

Using this association, we can find right-coset representatives of $\Gamma$ in $\Gamma[\mu]$: $$\Gamma[\mu]=\Gamma\cup \Gamma \mu\cup \Gamma \mu g\cup \Gamma \mu g f.$$ In particular, if we take a fundamental domain $R$ for the action of $\Gamma[\mu]$, then $R\cup \mu(R)\cup \mu g(R)\cup \mu gf(R)$ is a fundamental domain for the action of $\Gamma$.

Extending from a fundamental domain

In light of the above discussion, we can construct a partial pseudovertex by finding a fundamental domain $R$, choosing sets that will become $\mu(R)$, $\mu g(R)$, and $\mu gf(R)$, defining the action of $\mu$ on each of these, then extending everything by $\Gamma$. This is relatively straightforward except for the relation that $\mu$ and $g$ have to satisfy, making for some fairly tedious verifications.

Theorem B: Let $R\subseteq (\mathbb{C}\setminus\mathbb{R})$, and $\mu:R\to (\mathbb{C}\setminus\mathbb{R})$ a continuous function. Assume the following:

(a) $\mu$, $\mu_g:z\mapsto z^{-1}\mu(z)$, and $\mu_{gf}:z\mapsto \frac{1-\mu(z)}{1-z}$ are each injective on $R$;

(b) the sets $R$, $\text{im}(\mu)$, $\text{im}(\mu_g)$, $\text{im}(\mu_{gf})$ are disjoint;

(c) the set $S:=R\cup\text{im}(\mu)\cup\text{im}(\mu_g)\cup\text{im}(\mu_{gf})$ contains no two points in the same $\Gamma$-orbit.

Then $\mu$ can be extended to a function on $U:=\Gamma\cdot S$ satisfying the pseudovertex relations.

Proof: By the assumptions, we can write any $z\in U$ as exactly one of $\gamma(w)$, $\gamma(\mu(w))$, $\gamma(\mu_g(w)))$, or $\gamma(\mu_{gf}(w))$, for some unique choice of $\gamma\in\Gamma$ and $w\in R$. We will define $\mu(z)$ recursively, inducting on the number of generators $f,g,h$ needed to write $\gamma$.

For $w\in R$, define $\mu(\mu(w)):=w$, $\mu(\mu_g(w)):=w^{-1}$, and $\mu(\mu_{gf}(w)):=(1-w)^{-1}$; this defines $\mu$ on $S$ (the base case $\gamma=1$). Now suppose $\mu(z)$ is defined for some $z\in U$; we can then define $\mu(f(z)):=1-\mu(z)$, $\mu(g(z)):=z^{-1}\mu(z)$, and $\mu(h(z)):=\overline{\mu(z)}$. Repeating this as often as necessary, we obtain a definition of $\mu$ for all $z\in U$. One must check that this is well-defined: if two words in the symbols $f,g,h$ have the same product in $\Gamma$ then applying the recursive definition to each word yields the same output. It suffices to check that the relations $f^2=g^2=h^2=1$, $fh=hf$, $gh=hg$, and $fgf=gfg$ yield the same output when the recursive definition is applied to each side. The first five are straightforward so we just illustrate the last of these: $$\mu( fgf(z))=1-\mu(gf(z))=1-f(z)^{-1}\mu(f(z))=1-\frac{1-\mu(z)}{1-z}=\frac{(1-z)-(1-\mu(z))}{1-z}=\frac{z-\mu(z)}{z-1}=\frac{1-z^{-1}\mu(z)}{1-z^{-1}}=(1-g(z))^{-1}(1-\mu(g(z)))=fg(z)^{-1}\mu(fg(z))=\mu(gfg(z)).$$ Thus $\mu$ is well-defined, and the first three pseudovertex relations follow by definition. Note that we have $\mu_g=\mu\circ g|_R$ and $\mu_{gf}=\mu\circ g\circ f|_R$. We just need to check $\mu^2=1$.

Lemma C: Let $z\in U$ and $\gamma\in\langle h,f,\mu\rangle$. If $\mu^2 z=z$ then $\mu^2 \gamma z=\gamma z$.

Proof: $\gamma$ and $\mu$ commute, so $\mu^2 \gamma z=\gamma\mu^2 z=\gamma z$. $\square$

Lemma D: Let $z\in U$. If $\mu^2 z=z$ and $\mu^2 gz=gz$ then $\mu^2 g\mu z=g\mu z$.

Proof: Write the pseudovertex relation $\mu(g(z)):=z^{-1}\mu(z)$ as $\mu g z=g z\cdot \mu z$. Then

$$\mu\mu g\mu z=\mu (g\mu z\cdot \mu\mu z)=\mu(g\mu z\cdot z)=\mu g(gz\cdot \mu z)=\mu g\mu g z =g\mu g z\cdot \mu\mu gz=g(gz\cdot \mu z)\cdot gz=g\mu z.\square $$

Returning to the proof of Lemma B, let $w\in R$. We first check explicitly that $\mu^2\gamma w=\gamma w$ for $\gamma\in\{1,g,gf\}$: We have $\mu^2(w)=w$ by how we defined $\mu$ on $\text{im}(\mu)$, we have $\mu^2gw=\mu(\mu_g w)=gw$ by the definitions of $\mu_g$ on $R$ and of $\mu$ on $\text{im}(\mu_g)$, and likewise we have $\mu^2gfw=\mu(\mu_{gf} w)=gfw$.

Now by Lemma C, we can conclude that $\mu^2\gamma w=\gamma w$ for $$\gamma\in\{\mu,\mu g,\mu gf,f,fg,fgf,f\mu,f\mu g,f\mu gf\}.$$ By Lemma D, we can then conclude that $\mu^2\gamma w=\gamma w$ for $$\gamma\in\{g\mu,g\mu g,g\mu gf,gf\mu,gf\mu g,gf\mu gf\}.$$ (For the last three of these use $f\mu=\mu f$, together with $gfg=fgf$ and $gfgf=fg$ for the second last and last, respectively.) We can therefore apply Lemma C again to get $\mu^2\gamma w=\gamma w$ for $$\gamma\in\{fg\mu,fg\mu g,fg\mu gf,fgf\mu,fgf\mu g,fgf\mu gf\}.$$ (Note that the 24 elements listed above exhaust every combination of an element $1,f,g,fg,gf,fgf$ of $\langle f,g\rangle$ multiplied by one of $1$, $\mu$, $\mu g$, or $\mu gf$.) Finally, we apply Lemma C one more time to all the $\gamma$ above to get $\mu^2 h\gamma w=h\gamma w$. Thus the relation $\mu^2(z)=z$ holds for all $z$ of the form $\gamma w,\gamma\mu(w),\gamma\mu g(w),\gamma\mu gf(w)$, where $w\in R$ and $\gamma\in \Gamma$; that is, for all $z\in U$. Thus $\mu$ is in fact a pseudovertex. $\square$

Perturbing a pseudovertex

Let $$\mu(z):=\frac{(z+\overline{z})(z-1)}{z-\overline{z}}$$ be defined using the pseudovertex $X(4)$; we can rewrite this as $$\mu(a+bi)=a+\frac{a(1-a)}{b}i.$$ Let $R$ be the region $$R:=\{x+yi\in\mathbb{C}:(1-x)^2+y^2\leq 1,\; (x-\tfrac12)^2+y^2\geq\tfrac14, \; x\leq\tfrac12,\; y>0\}.$$ One can check that $R$ is a fundamental domain for $\Gamma[\mu]$, and that $R$, $\text{im}(\mu)$, $\text{im}(\mu\circ g)$, and $\text{im}(\mu\circ g\circ f)$ together make a (disconnected) fundamental domain for $\Gamma$. See the figure below; the green lines partition the plane into $48$ regions that are sent to each other by $\Gamma[\mu]$. The blue region is $R$, and the purple regions from top to bottom are $\mu(R)$, $\mu gf(R)$, and $\mu g(R)$.

Now let $\delta:R\to \mathbb{C}$ be a small continuous perturbation. Specifically, we choose $\delta$ to satisfy the following constraints:

• $\delta(z)=0$ for $z$ on the boundary of $R$.
• For $z$ in the interior $R^\circ$, $$|\delta(z)|<\min\{d(\mu(z),\text{im}(\mu)^c), |z|d(\mu_g(z),\text{im}(\mu_g)^c), |1-z|d(\mu_{gf}(z),\text{im}(\mu)^c)\},$$ where $d(z,X^c)$ denotes the minimum distance from $z$ to a point in the complement of $X$.
• For $z,z'\in R$, $$|\delta(z)-\delta(z')|<|\mu(z)-\mu(z')|,$$ $$|\frac{\delta(z)}{z}-\frac{\delta(z')}{z'}|<|\mu_g(z)-\mu_g(z')|,$$ $$|\frac{\delta(z)}{1-z}-\frac{\delta(z')}{1-z'}|<|\mu_{gf}(z)-\mu_{gf}(z')|.$$

The second condition can be obtained since $\mu,\mu_g,\mu_{gf}$ are each homeomorphisms from $R^\circ$ onto their respective images, so $z\in R^\circ$ implies that the distances are all positive. Just to show that there are lots of options that satisfy the third constraint as well, here's one construction: Let $y$ be a point where the derivative matrix for $\mu$ is invertible. This implies that for some small ball of radius $r$ around $y$, we have $|\mu(z)-\mu(z')|\geq c|z-z'|$ for some positive constant $c$ and all $z,z'$ in the ball. So we can pick any $0<t<1$ and set $\delta(z)=tc(r-|z-y|)$ for $|z-y|\leq r$ and $\delta(z)=0$ otherwise, in which case we obtain $$|\delta(z)-\delta(z')|=tc||z-y|-|z'-y||\leq tc|z-z'|<|\mu(z)-\mu(z')|.$$ If we choose $y$ such that the derivatives of $\mu_g$ and $\mu_{gf}$ are also invertible, we can use the same idea to pick $\delta$ to satisfy all constraints simultaneously.

The third constraint implies that $\mu+\delta$, $(\mu+\delta)_g$, and $(\mu+\delta)_{gf}$ are all injective onto their respective images, and the second constraint implies that $\mu+\delta$, $(\mu+\delta)_g$, and $(\mu+\delta)_{gf}$ have the same images as $\mu$, $\mu_g$, and $\mu_{gf}$, respectively. Thus $\mu+\delta$, restricted to the interior $R^\circ$ of $R$, satisfies the assumptions of Theorem B. Therefore it extends to a pseudovertex $\mu'$ on $\Gamma\cdot S$, which is the domain of $\mu$ minus the translates of $\partial R$. But since $\mu=\mu_0$ on $\partial R$, we can continuously extend $\mu'$ to the whole domain of $\mu$ by setting $\mu'(z):=\mu(z)$ on any translate of $\partial R$. Thus $\mu'$ doesn't just define a partial pseudovertex, it actually defines a complete pseudovertex (as it's defined wherever $X(4)$ is defined).

Answer 2

Not an answer. I'm just expanding a comment about @PeterTaylor's observation that the known pseudovertices $X(4)$, $X(74)$, $X(1138)$ lie on the Neuberg cubic ...

Bernard Gibert's "Pairs and Triads of points on the Neuberg Cubic connected with Euler Lines and Brocard Axes Isometric Parallel Chords" Proposition 1 characterizes the Neuberg cubic of $\triangle ABC$ as the locus of points $P$ such that the Euler lines of $\triangle ABC$, $\triangle PBC$, $\triangle APC$, $\triangle ABP$ concur (at $M$ in the figure). Consequently, it's also true that the Neuberg cubics of all four triangles contain all four points $A$, $B$, $C$, $P$ (and a companion point, $P'$, with the same properties).

This kind of interchangeability is at least reminiscent of the pseudovertex property, so it seems not-unreasonable to search the Neuberg cubic for other pseudovertex candidates. An explicit parameterization of the cubic might aid the search, so I'm providing one here.

Every point $M$ on the Euler line of $\triangle ABC$ corresponds to two points on the cubic (see animation and description below), so that the latter can be parameterized by the former.

Following a construction from Gibert, define circumcenter $O:=X(3)$ and orthocenter $D:=X(4)$, as well as $E:=X(74)$ and $F:=X(1138)$. Let $E'$ be the dilation of $E$ in $O$ by factor $-2$. For $M$ on the Euler line (through $O$ and $D$), let $O'$ be the reflection of $O$ in $M$. Then, we find $P$ and $P'$ on the cubic via the intersection of $\overleftrightarrow{O'E'}$ and the (necessarily rectangular) circumhyperbola through $A$, $B$, $C$, $D$, $M$. In particular, if $M = O + m(D-O)$, we can parameterize the barycentric $A$-coordinate of $P$ and $P'$ (with $B$- and $C$-coordinates following cyclically) as (deep breath) ... $$(\sin A)\left(\begin{array}{c} 2\left(m(m-1)^2\sigma^2+m\sigma_a\sigma_b\sigma_c+\sigma\right)\left( 2m(2\cos A-\cos(B-C)) - \cos A \right) \\ +\left(1-m^2\pm n\right)\left(m\sigma(2\cos A-\cos(B-C))+\sigma_b\sigma_c\cos A+2\sigma\cos B\cos C\right) \end{array}\right)$$ where $$\begin{align} \sigma_a &:= \frac{(-a^2+b^2+c^2)^2-b^2c^2}{b^2c^2}=1+2\cos 2A, \quad\sigma_b := \cdots, \quad\sigma_c := \cdots \\[0.5em] \sigma\phantom{_x} &:=\sigma_a+\sigma_b+\sigma_c \\[0.5em] n^2 &:= \left(1-m^2\sigma\right)^2+4m(2m-1)(m(m-1)\sigma^2+m\sigma_a\sigma_b\sigma_c+\sigma) \end{align}$$ (As a sanity check, the animation (created in GeoGebra) uses the parameterization to determine $P$ and $P'$, not GeoGebra's geometric construction features.)

The known pseudovertices $X(4)$, $X(74)$, $X(1138)$ correspond to $m = 0, \frac12, \infty$. (The "other" hyperbola intersection points for those $m$ are $X(3)$, $X(1263)$, $X(30)$.) Of course, it's not the case that every (Kimberling-esque) triangle center on the Neuberg cubic corresponds to a "scalar", triangle-independent $m$. (For instance $X(399)$ and $X(8487)$ arise from $m=6\sigma/(3\sigma^2-4\sigma_a\sigma_b\sigma_c)$.) However, it might be sensible to make such a restriction in a first pass at searching for (or ruling out) other pseudovertices on the cubic.

So, in that first pass, "all we have to do" is find an appropriate $m$ (and $\pm$ sign) to yield a $P$ from $\triangle ABC$ that in turn yields $A$, $B$, $C$ from $\triangle PBC$, $\triangle APC$, $\triangle ABP$.

Clearly, this is easier typed than done, as the second-level application of the parameterization explodes in complexity. Perhaps at least some of the thorny algebra can be avoided by mining the geometric lore of Neuberg cubics; Gibert's page about the curve, and associated papers, themselves provide a good deal of lore to consider. (Perhaps the pseudovertex question itself has already been addressed.)

---

Addendum. [Moved to this non-answer.]

Answer 3

This is a report on an unsuccessful computational approach which is rather too long for a comment.

I work with complex numbers to represent the points in the obvious way.

It suffices to consider $\mu(z) = t(z,0,1)$ because this can be extended under the invariants to the full $t(z,z',z'')$. Since multiplication by a complex number is just rotation and scaling, $z^{-1} t(z,0,1) = t(1,0,z^{-1}) = t(z^{-1},0,1)$ so $\mu(z^{-1}) = z^{-1} \mu(z)$. Similarly, $z \to 1 - z$ is a half-rotation around $\tfrac12 + 0i$, so $\mu(1-z) = 1 - \mu(z)$.

The three known pseudovertices have the following $\mu$: $$\mu_4 = \frac{(z + \overline{z})(z-1)}{z - \overline{z}} \\ \mu_{74} = \frac{-z(3z\overline{z}^2 + 3z^2\overline{z} - 2\overline{z}^2 - 8z\overline{z} - 2z^2 + 3\overline{z} + 3z)}{(z-\overline{z})(z^2+2z\overline{z}-2z-\overline{z})} \\ {\mu_{1138} = \frac{(9z^2\overline{z}^3+9z^3\overline{z}^2+\overline{z}^4-7z\overline{z}^3-24z^2\overline{z}^2-7z^3\overline{z}+z^4+9z\overline{z}^2+9z^2\overline{z})(3z\overline{z}^2-\overline{z}^2-4z\overline{z}-z^2+3z)}{3(\overline{z}-z)(z^2+2z\overline{z}-2z-\overline{z})(\overline{z}^2+2z\overline{z}-2\overline{z}-z)^2}}$$

I therefore considered candidates of the form $\mu(z) = \frac{P(z, \overline{z})}{Q(z, \overline{z})}$ where $P$ is a polynomial of total degree $k$ and $Q$ is a polynomial of total degree $k-1$, both having real coefficients.

The general approach was to use Sage to expand $(z\overline{z})^k z\mu(z^{-1}) - (z\overline{z})^k \mu(z)$ and $\mu(1-z) - 1 + \mu(z)$ for $z = a+bi$ with the coefficients of $P$ and $Q$ as variables; then since the results should be identically zero, I consider both values as polynomials in $a$, $b$; separately take the real and imaginary part of each coefficient; and form the ideal given by all of these subcoefficients. Finally I ask Sage for the minimal associated prime ideals.

The approaches I then took to filter down the prime ideals were rather more ad hoc: for the simpler ones I just expanded $\mu(\mu(z)) - z$ in the non-eliminated variables to get a new ideal and look for its primes; for more complicated ones I took a small number of non-real values of $z$, calculated $\mu(\mu(z)) - z$ for those values, and obtained an ideal that way; and for the most recent cases treated it occurred to me that $f(b) = \mathfrak{Im}(\mu(\tfrac12 + bi)) : \mathbb{R} \to \mathbb{R}$ should be an involution and I obtained ideals from a combination of $f(f(b)) - b$ and the obvious fact that the equilateral triangle gives $\mu(\tfrac12 + \tfrac{\sqrt 3}2i) = \tfrac12 + \tfrac{\sqrt 3}6i$ or a pole; there is a slim possibility that the cases treated by the second approach contained a solution which was missed due to having a pole at one of the sample points. But I didn't find any solutions other than $X(4)$ and $X(74)$ in the following cases:

• $P$ and $Q$ fully general polynomials of total degree respectively $3$ and $2$;
• $P$ a fully general polynomial of total degree $4$; $Q = (z - \overline{z})Q'$ where $Q'$ is a fully general polynomial of total degree $2$;
• $P$ a polynomial with monomials of total degree $3$ to $6$; $Q = (\overline{z}-z)(z^2+2z\overline{z}-2z-\overline{z})(\overline{z}^2+2z\overline{z}-2\overline{z}-z)$.

The almost-fully-general quartic case took several hours of calculations just to yield the initial prime ideals, before taking into account the involutive requirement, so I don't think I can push this approach any further.

Answer 4

Transferring the Addendum to my previous non-answer to a non-answer of its own, as it's beginning to sprawl (and because it actually seems more significant) ...

---

It happens that $X(4)$, $X(74)$, $X(1138)$ also lie on the circumcubic $K279$, for which there is this geometric description attributed to Angel Montesdeoca:

Let $\triangle A' B' C'$ be the cevian triangle of a point $P$, let $A^\star$, $B^\star$, $C^\star$ be the circumcenters of $\triangle AB'C'$, $\triangle A'BC'$, $\triangle A'B'C$, and let $Q$ be the circumcenter of $\triangle A^\star B^\star C^\star$. Then $K279$ is the locus of $P$ such that $Q$ lies on the Euler line.

Unlike with Neuberg, given a point $P$ on this curve, the corresponding cubic for $\triangle PBC$ does not necessarily pass through $A$. Indeed (barring triangle degeneracies), Mathematica-assisted symbol crunching (see below) shows that this occurs for (Kimberling-esque) triangle center $P$ when and only when $P$ is one of $X(4)$, $X(74)$, $X(1138)$. Effectively, then, the property

$P$ lies on the $K279$ of $\triangle ABC$, and $\triangle ABC$ is inscribed in the $K279$s of $\triangle PBC$, $\triangle APC$, $\triangle ABP$.

characterizes the known pseudovertices. This could be useful in the search for (or the ruling-out of?) other candidates.

---

For the symbol-crunching, we start with the barycentric $u:v:w$ equation for $K279$ relative to $\triangle ABC$:

$$\sum_{cyc} b^2 c^2 ((b^2 - c^2)^2 + a^2 (b^2 + c^2 - 2 a^2))\; u (v^2 - w^2) = 0 \tag{1}$$ Then, considering $P$ with barycentric coordinates $u:v:w$, the $K279$ with respect to $\triangle PBC$ has barycentric $u':v':w'$ equation that derives from $(1)$ by substituting $u\to u'$, $v\to v'$, $w\to w'$, and $$\begin{align} b^2 &\to |PC|^2 = \frac{a^2 v^2 + b^2 u^2 + (a^2+b^2-c^2) u v}{(u + v + w)^2} \\[0.5em] c^2 &\to |PB|^2 = \frac{a^2w^2 + c^2 u^2 + (a^2-b^2+c^2) u w }{(u+v+w)^2} \end{align}$$ Since $A$ has barycentric coordinates $u':v':w' = u+v+w:-v:-w$ relative to $\triangle PBC$, we substitute those, as well. This yields a degree-7 $uvw$ polynomial, whose $184$ terms I won't transcribe here. Mathematica confirms that the polynomial vanishes if $P$ is one of $X(4)$, $X(74)$, $X(1138)$. (It does not vanish when $P$ is $X(2)$, the centroid of $\triangle ABC$, unless $b=c$.)

Applying Mathematica's Resultant to eliminate $u$ from this and $(1)$ gives this equation (ignoring powers of factors):

$$vw(v + w)\cdot(v-w)\cdot f_4 \cdot f_{74} \cdot f_{1138} \cdot f = 0$$

Here, $$f_4 := v(-b^2+c^2+a^2) -w(-c^2+a^2+b^2)$$ so that $$f_4 = 0 \quad\implies\quad v = \frac{k}{-b^2+c^2+a^2} \quad w = \frac{k}{-c^2+a^2+b^2} \quad\left(u = \frac{k}{-a^2+b^2+c^2}\right)$$ (with the $u$-coordinate symmetrically completing the "center"). Thus, this factor can vanish only at center $X(4)$. Likewise, $f_{74}$ and $f_{1138}$ are linear factors in $v$ and $w$ that can vanish only at centers $X(74)$ and $X(1138)$.

Further, our interest in centers allows us to ignore factors $v$, $w$, $v+w$. Factor $v-w$ vanishes when $v=w(=u)$, which corresponds to centroid $X(2)$, a candidate we have already ruled-out.

Factor $f$ is a degree-$6$ polynomial in $v$ and $w$, with $711$ terms. I haven't done a proper analysis of it; however, when, say, $(a,b,c)=(6,9,13)$, the solutions are all non-real. I'm pretty sure we can consider this extraneous in general. (There's probably a neat way to avoid this factor altogether by first reducing the system or something, but I haven't found it.)

Of course, it would be best to devise a more-direct, less-computational argument, perhaps one based on Montesdeoca's geometric description of $K279$. (One may note that the cevian triangle of $P$ with respect to $\triangle ABC$, and that of $A$ with respect to $\triangle PBC$, are always the same triangle, so there's a start! :) That's what I'm investigating now.

Answer 5

[Yet another answer ...]

In a post to the Euclid discussion group at Groups.io, "Lky" asserted that X(4), X(74), and X(1138) are (in OP's terminology) generalized triangle vertices or (as I call them) "tetradic centers". (The post appeared in 2020, beating my observation of X(1138)'s tetradic nature by about a year. Such is life!)

Lky also stated matter-of-factly that the point $P$ such that the Brocard axes of $\triangle PBC$, $\triangle APC$, $\triangle ABP$, $\triangle ABC$ are parallel is tetradic (which, assuming existence and uniqueness, it would have to be). I believe I have identified this point as the intersection of three circumscribed toric sections (each the locus of points corresponding to Brocard parallelism for one of the associated triangles); full analysis is complicated by the fact that various expressions are entangled in enormous and thorny cubic equations. Nevertheless, this may well be the fourth tetradic center.

In a follow-up post, Vu Thanh Tung conjectured a complete family of such centers, one for each real $t$, corresponding to parallelism of lines through the circumcenter with points $X$ satisfying $$|XA|^2(b^t - c^t) + |XB|^2(c^t - a^t) + |XC|^2(a^t - b^t) = 0$$ (Cases $t=2$ and $t=-2$ are the Euler line (for which the target point is X(1138)) and Brocard axis (with Lky's point).) The idea is inspired by results in a 2001 Forum Geometricorum article from Hatzipolakis, et al, describing the locus of points for which the four associated lines concur. Tung is thus positing that exactly one point on the locus corresponds to concurrence at a point-at-infinity; could be true ... if not for arbitrary $t$, then perhaps for a reasonably-describable subset thereof. (Likewise, there may be a unique point yielding concurrence of the associated lines at some other distinguished point along them.)

---

By the way ... Lky also asserted that X(16), the Second Isodynamic Point, is tetradic. However, due to definitional entanglement with X(15), the First Isodynamic Point, neither is tetradic.

And yet ...

Associating $X(15)$ and $X(16)$ with respective signs "$+$" and "$-$", if $P$ is $\triangle ABC$'s isodynamic point for sign $\sigma$, then $A$ is $\triangle PBC$'s isodynamic point for sign $-\operatorname{sgn}\left(\cos\left(A-\sigma\frac\pi6\right)\right)$; likewise for $B$ and $C$. So, the points form a kind of "bi-tetradic" pair. Nifty!

It's worth noting that the isodynamic points are often defined in tandem; eg, as "the points" where three particular Apollonian circles concur. They also share properties, and many results involve circles/lines/whatever related to both of them together. So, geometrically, they are largely indistinguishable, which makes the standard First/Second distinction seem a little arbitrary: As mutual inverses in the circumcircle, one (declared the "First") always lies inside the circle, and the other (the "Second") lies outside. These declarations correspond nicely with a simple choice of sign in the barycentric definitions of these points (which, in turn, corresponds to the $\pm$ association I used above), but they raise the question:

Can we impose an alternative distinction that makes each isodynamic point alone properly tetradic?

I'll stop typing now.

Answer 6

I found this property of X(1138) no later than 2015. I called them X(4),X(74) and X(1138) system centers and tried to find another one but failed. I also found they all lie on the Neuberg's cubic and hence Neuberg quartic several years ago(a generalization of the Neuberg cubic). I recently computed the equation of the Neuberg quartic on the complex plane. I tried to find system centers of a quadrangle(= four vertices) but also failed. I just found another interesting property of these system centers these days. Given A,B,C together with their system center D, we can get the nine point conic of A,B,C,D(circumconic through the midpoints of the six sides connecting each pair of vertices). On the case of D=X(4), we get the famous nine point circle of ABC(with the eccentricity 0). On the case of D=X(74), we get a rectangular hyperbola(with the eccentricity the square root of 2). On the case of D=X(1138), we get a hyperbola with the eccentricity of 2. Maybe this property help us to find another one system center.