Let $ABC$ a triangle in the plane, but $D$ a point in (R3) space, such that the angles $\phi=ADB=BDC=CDA$ are equal. Let $E$ be the footpoint of $D$ in $ABC$. $E(\phi)$ describes a (irreducible) septic $S$ (that has 5 asymptotes, for the record).
Points that lie on $S$ (checked with ETC): Incenter $I$, Circumcenter $U$, Orthocenter $H$ (isolated), $A,B,C$ (double points), 1st (Fermat $F$) and 2nd isogonic center $D$.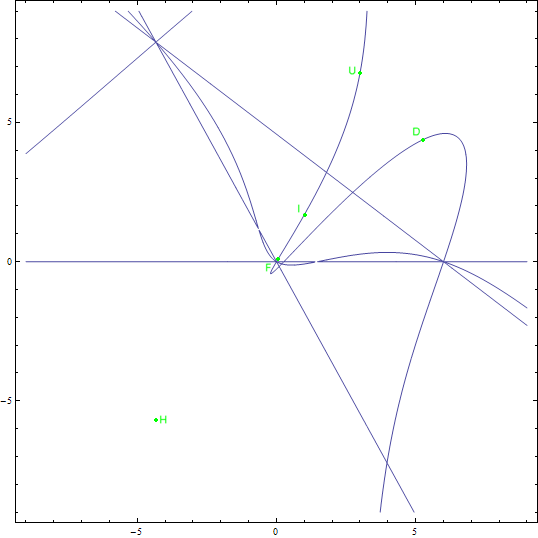 
It's near-lying to assume that $S$ is the Darboux septic (the only I heard of :-) of some triangle $XYZ$ where $XYZ$ is the foobar transform of $ABC$ but with that septic I'm still skeptic. (SCNR)
Can you help? Gibert's invaluable site went kaput, and I don't even find the original paper to the Darboux septic.
$\begingroup$
$\endgroup$
3
asked Oct 30, 2019 at 12:28
-
$\begingroup$ Bernard Gibert's site has been archived on the Wayback Machine: web.archive.org/web/20190321092028/http://… . Specifically for septics, see web.archive.org/web/20171212225952/http://… . Have you tried checking the isogonal, cyclocevian etc. conjugates of your points, to see whether they lie on a simpler curve? Also, is there an equivalent condition that "stays inside the plane"? $\endgroup$– darij grinbergCommented Oct 31, 2019 at 21:14
-
$\begingroup$ Ah, the Wayback Machine. Already played with the though of looking there but was too pessimistic. :-) The description already should rule it out - Darboux' septic has triple points. $\endgroup$– Hauke ReddmannCommented Nov 1, 2019 at 14:39
-
$\begingroup$ For what it's worth, Gilbert's site is alive and well. bernard-gibert.pagesperso-orange.fr $\endgroup$– brainjamCommented Jan 9, 2021 at 4:25
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
This is just a back of an envelope calculation so not really an answer, but too long for a comment. I hope it helps: we work in $(r,s,t)$ space ($p$ and $q$ are constants determined by the shape of the triangle). Then your curve is the projection onto the $(r,s)$ plane of the algebraic variety $$p_1(r,s,t)=p_2(r,s,t), p_3(r,s,t)=p_4(r,s,t),$$ where $$p_1(r,s,t)=(r^2-r+s^2+t^2)^2((r-p)^2+(s-q)^2+t^2),$$ $$ p_2(r,s,t)=((r-1)(r-p)+s(s-q)+t^2)^2(r^2+s^2+t^2), $$ $$ p_3(r,s,t)=(r^2-r+s^2+t)^2((r-p)^2+(s-q)^2+t^2) $$ and $$p_4(r,s,t)= ( r^2-rp+s^2-sq+t^2 )^2(( r-1 )^2 +s^2+t^2 ) .$$ Perhaps Gröbner bases can be used to tease out what you want.
