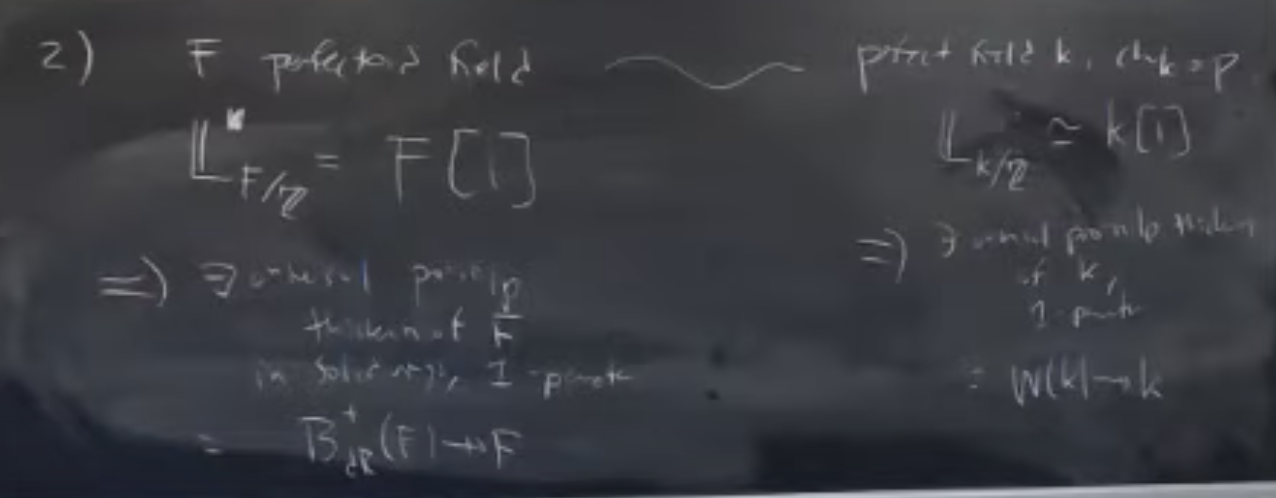Let me point out that, the original statement in the video of Dustin Clausen's
talk is essentially correct (although it might have been inadvertent,
according to his answer), thanks to Arpon Raksit's paper Hochschild homology and the derived de Rham cohomology revisited (arXiv link).
For sake of simplicity, we fix a base ring $R$. Roughly speaking, there is a
nonconnective version of animated $R$-algebras due to Bhatt–Mathew, which is
named after derived (commutative) $R$-algebras in Raksit's paper (§4). The
point is that, animated $R$-algebras are precisely modules over the monad
$\mathbb{L}{\operatorname{Sym}} : D (R)_{\geq 0} \rightarrow D
(R)_{\geq 0}$ on the $\infty$-category of connective $R$-module spectra (or
connective $R$-modules, by abuse of terminology). Using Goodwillie calculus,
one can extend to the whole $\infty$-category $D (R)$ of $R$-modules,
obtaining a monad $\mathbb{L}{\operatorname{Sym}} : D (R)
\rightarrow D (R)$, and derived $R$-algebras are modules over this monad,
whose $\infty$-category will be denoted by
${\operatorname{DAlg}}_R$.
This formalism applies more generally to derived algebraic contexts (4.2.1) in
place of $D (R)$, and in particular, to the $\infty$-category
$\widehat{{\operatorname{DF}}}^{\geq 0} (R)$ of completely
(nonnegatively decreasingly) filtered $R$-modules, obtaining a monoid, whose
modules are completely filtered derived $R$-algebras, or equivalently,
nonnegative $h_-$-differential graded derived $R$-algebras
${\operatorname{DG}}_-^{\geq 0} {\operatorname{DAlg}}_R$
due to 5.1.5.
Similar to 5.3.2 (and even easier), the functor
$\operatorname{gr}^0\colon{\operatorname{DG}}_-^{\geq 0} {\operatorname{DAlg}}_R
\rightarrow {\operatorname{DAlg}}_R$ admits a left adjoint
${\operatorname{Cpl}} : {\operatorname{DAlg}}_R
\rightarrow {\operatorname{DG}}_-^{\geq 0}
{\operatorname{DAlg}}_R$. There are two ways to understand this functor:
- By adjunction, $\operatorname{Cpl}(A)$ is the initial completely filtered derived $R$-algebra $B$ equipped with a map $A\to\operatorname{gr}^0(B)$ of derived $R$-algebras. In some sense, this is closely related to the final object in the "pro-infinitesimal site" of $A/R$ (reminder: the site is opposite to the category of rings);
- If we start with a quotient $R / I$ where
$I \subseteq R$ is an ideal generated by a Koszul-regular sequence, then the
image under this left adjoint is the $I$-completion $R_I^{\wedge}$ along with
the $I$-adic filtration (the readers could convince themselves that this
object is somehow initial), so this functor should be understood as a variant
of adic completion, which justifies the notation.
The proof of 5.3.6 essentially leads to the following:
Proposition. Given a derived
$R$-algebra $A$, the $n$-th associated graded piece
${\operatorname{gr}}^n ({\operatorname{Cpl}} (A))$ is
given by the symmetric product $\mathbb{L}{\operatorname{Sym}}_A^n
({\operatorname{gr}}^1 ({\operatorname{Cpl}} (A)))$ (and
in particular, ${\operatorname{gr}}^0
({\operatorname{Cpl}} (A)) = A$), and we have an equivalence
$\mathbb{L}_{A / R} \simeq
\Sigma {\operatorname{gr}}^1 ({\operatorname{Cpl}} (A))$ of $A$-modules where $\mathbb{L}_{A / R}$ is the
(algebraic) cotangent complex of $R$.
In particular, consider the case that $R =\mathbb{Z}$. If $A =\mathbb{F}_p$,
or more generally, $A$ is given by a perfect $\mathbb{F}_p$-algebra, then the
cotangent complex $\mathbb{L}_{A / R}$ has
${\operatorname{Tor}}$-amplitude in $[1, 1]$, therefore the
$A$-module ${\operatorname{gr}}^1 ({\operatorname{Cpl}}
(A))$, and consequently the $A$-modules ${\operatorname{gr}}^{\ast}
({\operatorname{Cpl}} (A))$, are flat. It follows that the
underlying derived ring ${\operatorname{Fil}}^0
({\operatorname{Cpl}} (A))$ of ${\operatorname{Cpl}}
(A)$ is concentrated in degree $0$, and there is a surjective map
${\operatorname{Fil}}^0 ({\operatorname{Cpl}} (A))
\rightarrow {\operatorname{gr}}^0 ({\operatorname{Cpl}}
(A)) = A$ of rings whose kernel $I$ is quasiregular, and by Quillen's result,
${\operatorname{Fil}}^n ({\operatorname{Cpl}} (A))$ are
simply given by $I^n$.
On the other hand, if we consider a reasonable ($\infty$-)category of
deformations of the $R$-algebra $A$, say the category of surjective maps $B
\twoheadrightarrow A$ of $R$-algebras whose kernel is finitely generated (the
completeness with respect to a non-finitely generated ideal is usually
pathologic), then this ($\infty$-)category should be a full subcategory
${\operatorname{Def}}_R$ of $\{ S \in
{\operatorname{DG}}_-^{\geq 0} {\operatorname{DAlg}}_R |
{\operatorname{gr}}^0 (S) \simeq A \}$. Having this in mind, when
the cotangent complex $\mathbb{L}_{A / R}$ is, as an $A$-module, equivalent to
a shift $P [1]$ where $P$ is a finite projective $A$-module, then
${\operatorname{Cpl}} (A)$ lies in this subcategory
${\operatorname{Def}}_R$ and the universal property follows.