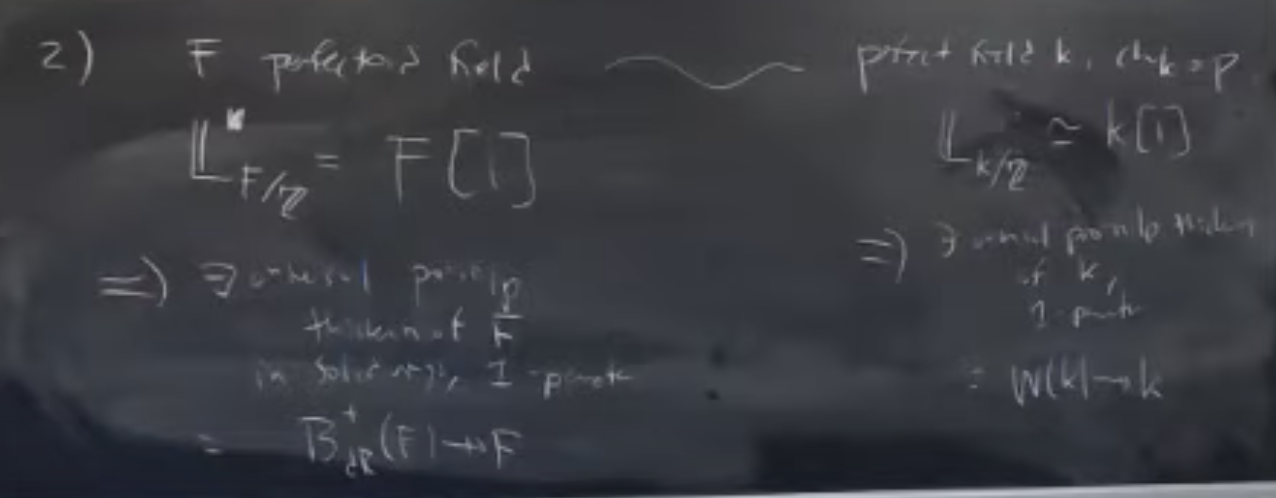In a remarkable lecture delivered on October 29th: New Foundations for functional analysis, Dustin Clausen suggests at the 40 minute mark a remarkable new construction interpretation of Fontaine's ring $B_{dR}^+$ making use of solid modules, the condensed analog of non-Archimedean completeness. This relies on a construction of the solid cotangent complex of a perfectoid field, which happens to be as simple as possible, a suspension of the field, and then proceeds to construct the desired period ring by analogy with the Witt vectors of a perfect ring. It's possible that I have misunderstood this story.
With the understanding that mathoverflow may not be the best place to push on unpublished results (feel free to close the question if it crosses the line), I wondered if anyone has a reference for or could offer a fully deformation theoretic construction of the Witt vectors, to at least have the other side of the analogy.
It seems to me that I can get a decent a priori construction of the Witt vectors by leveraging perfection (for cotangent vanishing and multiplicative lifting via p-adic contracting property of the Frobenius), but it might be difficult to get from here to the formulas, the $F$ and $V$ operators, the fact that when we begin with a field the result is a DVR. Excellent references abound, but I am curious whether there is any which begins and remains with the perspective of the cotangent complex.
The relevant blackboard is here: https://imgur.com/a/otKV4gG