I proveNote that they lie onthere exists a conic. Proving that it is ellipse requires checking some inequalities. I am not entirely sure that it is always so.
We starttriangle $\tilde{F}\tilde{D}\tilde{E}$ with
Lemma. Assume that on the picture side lengths 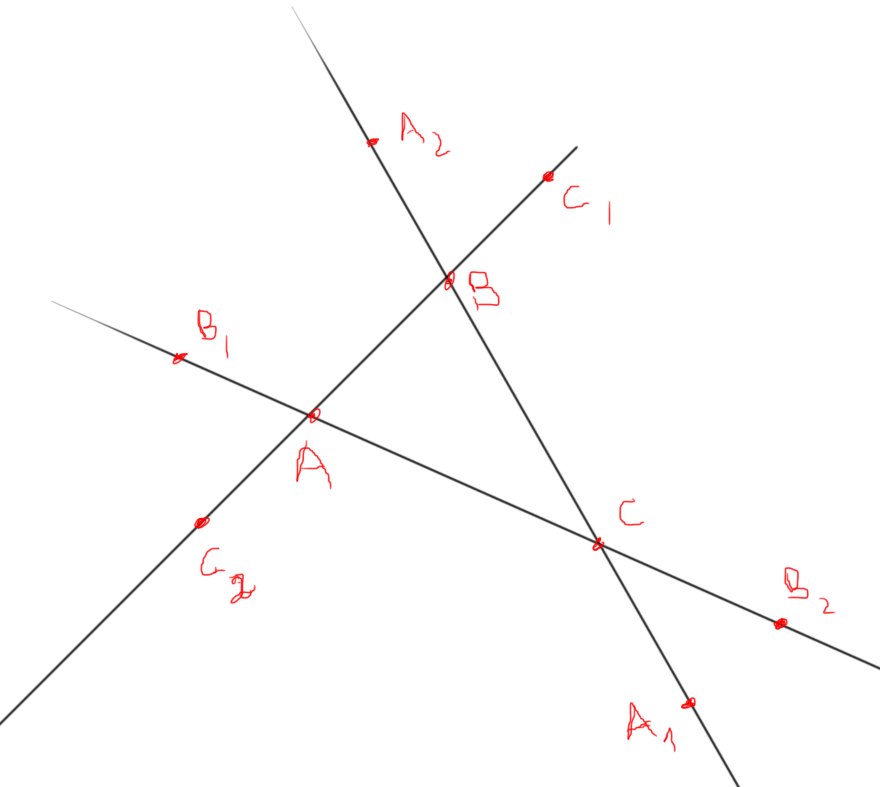 $\tilde{F}\tilde{D}=\sqrt{FD}$, $$\frac{AB_1\cdot AB_2}{AC_1\cdot AC_2}\cdot \frac{CA_1\cdot CA_2}{CB_1\cdot CB_2}\cdot
\frac{BC_1\cdot BC_2}{BA_1\cdot BA_2}=1.\quad\quad(\heartsuit)$$ Then$\tilde{E}\tilde{D}=\sqrt{ED}$, $A_1,A_2,B_1,B_2,C_1,C_2$ lie on a conic$\tilde{F}\tilde{E}=\sqrt{FE}$.
$\tilde{F}\tilde{D}=\sqrt{FD}$, $$\frac{AB_1\cdot AB_2}{AC_1\cdot AC_2}\cdot \frac{CA_1\cdot CA_2}{CB_1\cdot CB_2}\cdot
\frac{BC_1\cdot BC_2}{BA_1\cdot BA_2}=1.\quad\quad(\heartsuit)$$ Then$\tilde{E}\tilde{D}=\sqrt{ED}$, $A_1,A_2,B_1,B_2,C_1,C_2$ lie on a conic$\tilde{F}\tilde{E}=\sqrt{FE}$.
Proof. Apply inverse Pascal theorem Make an affine transform $\Phi$ which maps $F,E,D$ to the hexagon $C_2C_1A_2A_1B_2B_1$$\tilde{F}$, $\tilde{E}$, $\tilde{D}$ respectively. We shouldI claim that $\Phi$-images of $J,K,M,N,O,P$ are concylic. For proving this it suffices to check the pointsthat each quadrilateral $A_0=A_1A_2\cap B_1C_2$$KJMP$,$B_0=B_1B_2\cap C_1A_2$ $POJN$, $C_0=C_1C_2\cap A_1B_2$ are collinear. By Menelaus we have $A_0B:A_0C=(C_2B:C_2A)\cdot(B_1A:B_1C)$. Applying three such relations and$NMOK$ maps to a cyclic quadrilateral: their circumcircles either all coincide (inverse) Menelausthat we see that $A_0,B_0,C_0$need), or have concurrent radical axes, but these axes are collinear if and only ifthe sides of $(\heartsuit)$ holds$\triangle \tilde{F}\tilde{D}\tilde{E}$.
On your picture six segments cancel out asThe map $NE=NM$ etc$\Phi$ divides all lengths on line $ED$ by $\sqrt{ED}$ and all lengths on line $EF$ by $\sqrt{EF}$. For segments likeThus to prove that $ND$$NMOK$ is cyclic, we denote byshould check $S$ the double area of triangle$$EN\cdot EO/ED=EM\cdot EK/EF. \quad (\diamondsuit)$$ Since $AFD$$EN=EM$, (or$(\diamondsuit)$ reads as $CFE$$EO/ED=EK/EF$, or $BED$ --- three areas are equal due to your assumptions$OD/ED=KF/EF$, or $\frac{AB}{DA}=\frac{BC}{EB}=\frac{AC}{FC}$) write$OD\cdot EF=ED\cdot KF$, or $ND=AH+ED=AH+S/FD=(AH\cdot FD+S)/ED$${\rm Area}(CEF)={\rm Area}(DEB)$. And remaining terms in This follows from $(\heartsuit)$ also cancel out$\frac{AB}{DA}=\frac{BC}{EB}=\frac{AC}{FC}$.
