In 2014, I found a nice result in plane geometry, the result is a generalization of the Simson line theorem, and there are nine proofs for this result were published in [1]-[7]. Continuing, I find a new generalization of the old result as follows:
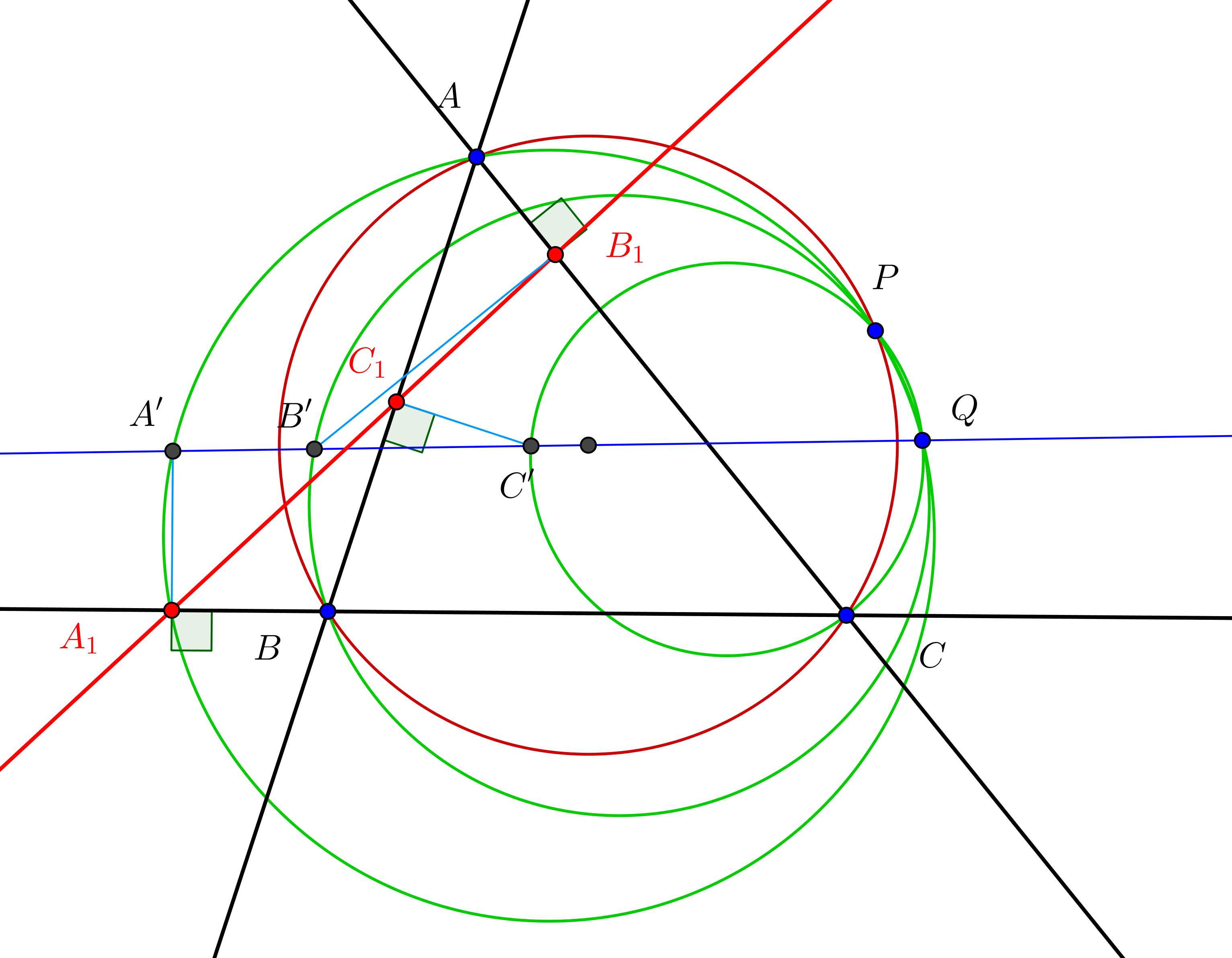
Problem: Let $ABC$ be a triangle, let $P$ be a point in the circumcircle, the circumcenter is $O$. Let $Q$ be the point in the plane. The circles $(APQ), (BPQ), (CPQ)$ meet $OQ$ again at $A', B', C'$ respectively. Let $A_1, B_1, C_1$ be the the projections of $A', B', C'$ onto $BC, CA, AB$ respectively. Then $A_1, B_1, C_1$ are collinear, and the new line through a fixed point on the Nine point circle when $Q$ be moved on the given line (or $P$ be moved in the circumcircle). When $Q$ in infinity we get the old result (the result I found in 2014).
My question, could you give a proof for this problem?
Note: Check the result is true with applet by click here
Note: You can see some problem around this configuration in
** References:**
[1]-Nguyen Van Linh, Another synthetic proof of Dao's generalization of the Simson line theorem, Forum Geometricorum, 16 (2016) 57--61.
[2]-Nguyen Le Phuoc and Nguyen Chuong Chi (2016). 100.24 A synthetic proof of Dao's generalisation of the Simson line theorem. The Mathematical Gazette, 100, pp 341-345. doi:10.1017/mag.2016.77.
[3]-Leo Giugiuc, A proof of Dao’s generalization of the Simson line theorem, tạp chí Global Journal of Advanced Research on Classical and Modern Geometries, ISSN: 2284-5569, Vol.5, (2016), Issue 1, page 30-32
[4]-Tran Thanh Lam, Another synthetic proof of Dao's generalization of the Simson line theorem and its converse, Global Journal of Advanced Research on Classical and Modern Geometries, ISSN: 2284-5569, Vol.5, (2016), Issue 2, page 89-92
[5]-Ngo Quang Duong, A generalization of the Simson line theorem, to appear in Forum Geometricorum.
[6]-Three other proofs by Telv Cohl, Luis Gonzalez, Tran Quang Huy A Generalization of Simson Line
[7]-Another proof https://www.artofproblemsolving.com/community/c6h1075523p5181203