My question: I am looking for a proof of problem as following:
Introduction: When I research a theorem as following:
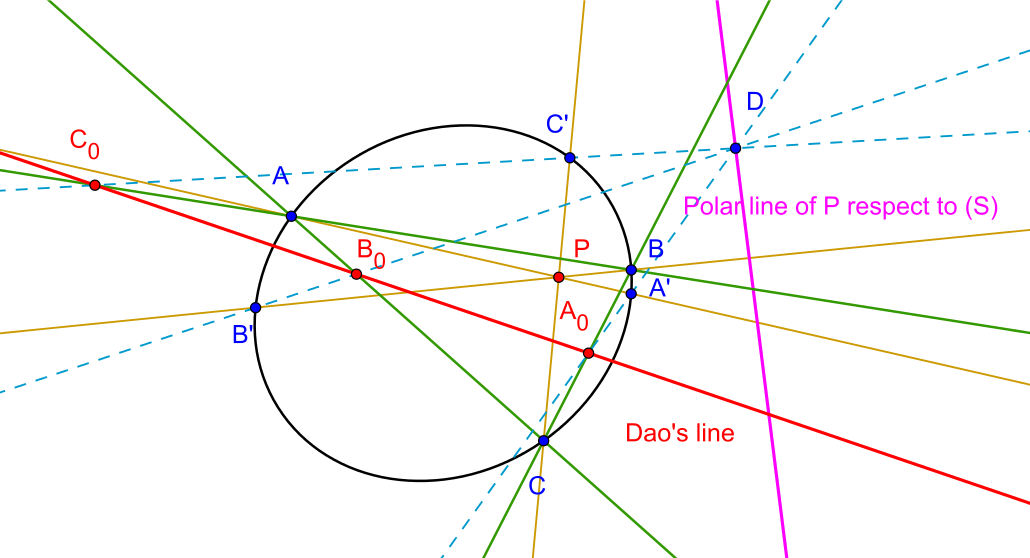
Theorem 1: Let $ABC$ be a triangle, let $(S)$ be a circumconic of $ABC$, let $P$ be a point on the plane. Let the lines $AP, BP, CP$ meet the conic again at $A', B', C'$. Let $D$ be a point on the polar of point $P$ with respect to $(S)$ or $D$ lies on the conic $(S)$. Let $A_0= DA' \cap BC$, $B_0= DB' \cap AC$; $C_0= DC' \cap AB$. Then $A_0, B_0, C_0$ are collinear. Further more four points $A_0, B_0, C_0, P$ are collinear if only if $D$ lie on the conic.
1-Nguyen Ngoc Giang, A proof of Dao theorem, Global Journal of Advanced Research on Classical and Modern Geometries, ISSN: 2284-5569, Vol.4, (2015), Issue 2, page 102-105
I found a nice result adding to configuration of theorem 1 as following:
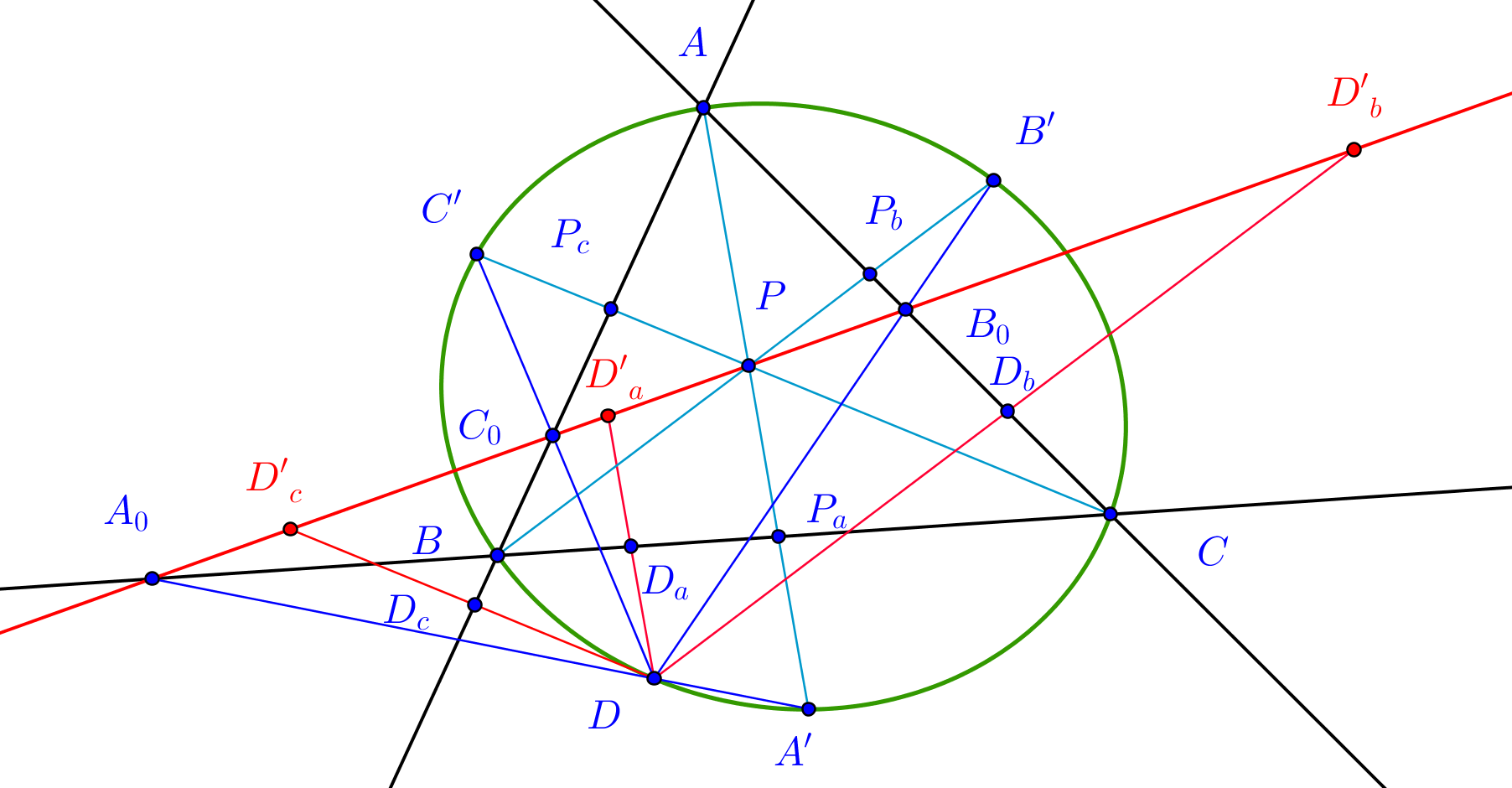
Problem: In the case D lies on the conic $(S)$. Let $AP$ meets $BC$ at $P_a$. Let the line through $D$ and parallel to $AP$, this line meets $BC$ at $D_a$. Let $D'_a$ on the ray $DD_a$ such that $\frac{\overline{DD_a}}{\overline{DD'_a}}=\frac{\overline{A'P_a}}{\overline{A'P}}$. Define $D'_b, D'_c$ cyclically. Then seven points $A_0, B_0, C_0, D'_a, D'_b, D'_c$ and $P$ are collinear.