I have asked this question already on mathstackexchange but got no answer (see https://math.stackexchange.com/questions/1795795/kazhdans-property-t-vs-residual-finiteness) and it was suggested that I may ask it here too.
There is a theorem that states that a discrete group $G$ with Kazhdan's Property $(T)$ and Property $F$ (so called factorisation property) is residually finite (see Kirchberg, Discrete groups with Kazhdan' s property T and factorization property are residually finite), i.e.
Kazhdan's Property $(T)$ + Property F $\Rightarrow$ Residual finiteness.
For the definitions of Kazhdan's Property $(T)$ and residual finiteness see e.g. the corresponding wiki-articles.
I am wondering if some kind of "converse" is true. More precisely, I am looking for some property, let us call it Property X, such that:
Residual finiteness + Property X $\Rightarrow$ Kazhdan's Property $(T)$.
Maybe there is something similar in the literature?
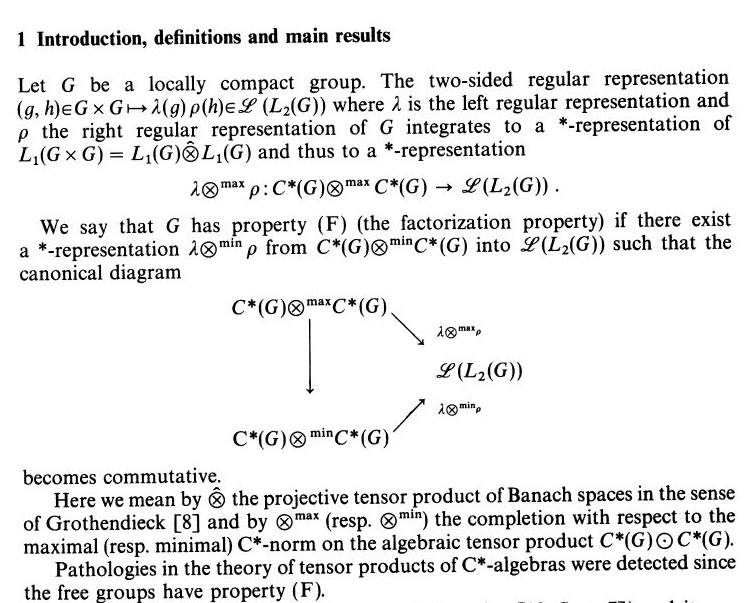
Definition for Property $F$ of the cited paper.