3
$\begingroup$

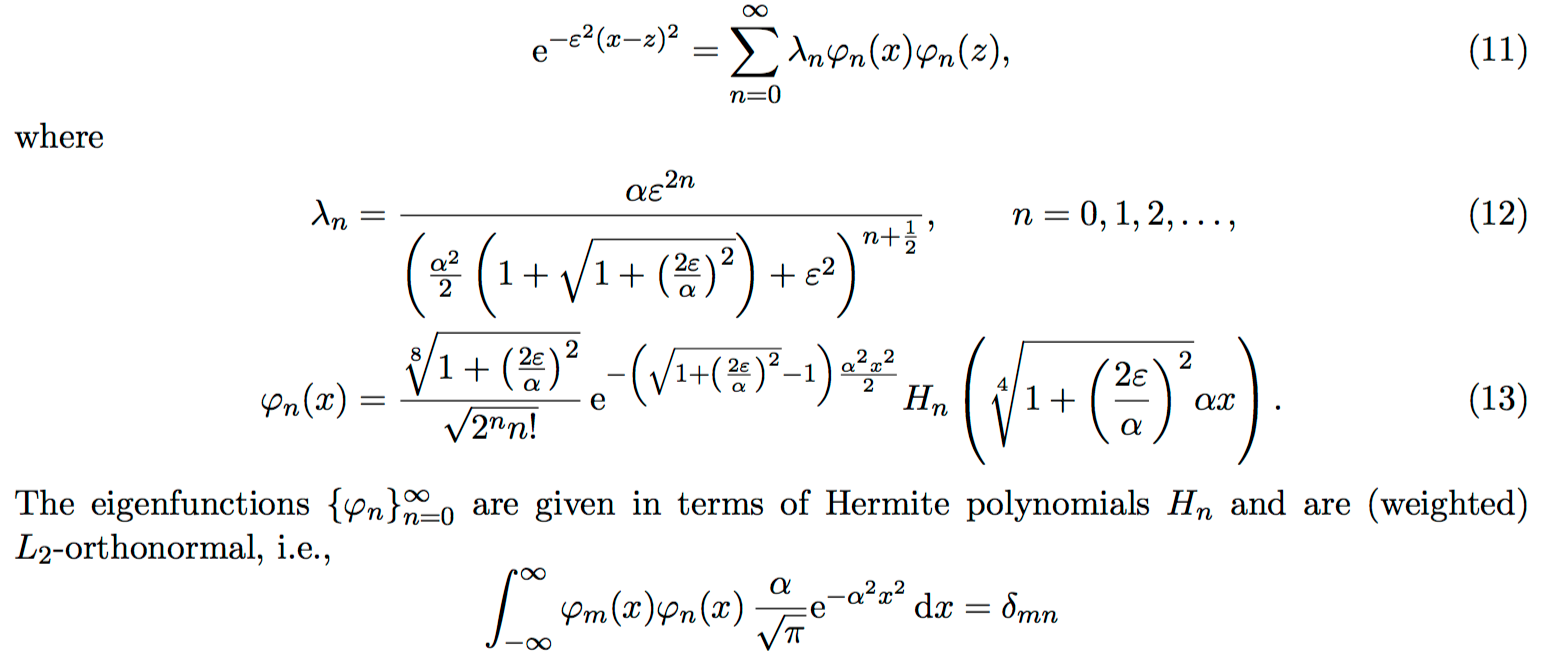
If I look at the Guassian kernel function $e^{- \frac {\vert x - y\vert_2^2 }{2 w^2 } }$ for $x, y \in \mathbb{R}$. Then w.r.t the Gaussian measure $N(\mu,\sigma)$ I believe it is true that this has a discrete spectrum such that the eigenfunction of the $i^{th}$ largest eigenvalue is proportional to $e^{-\frac{(x-\mu)^2 }{2 \sigma^2 } \frac{ -1+\sqrt{1+2\beta}}{2 } }H_{i-1} ( (\frac{1+2\beta }{4})^{\frac{1}{4}}\frac{x-\mu}{\sigma} )$ where $\beta = 2 \frac{\sigma^2}{w^2}$ and $H_{i-1}$ is the $(i-1)^{th}$-Hermite polynomial.

I can't remember where I saw this result and all I have is a note with me with this result written on it.

  • Can someone kindly reference to any derivation and or discussion of this?

  • Is there an immediate generalization of this to a case where $x,y \in \mathbb{R}^d$?

There is this discussion on MO here at, Gaussian kernel eigenfunctions and I would like to be able to relate that to this above result.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

for example, see Positive Definite Kernels by Gregory Fasshauer:

Improve this answer
$\endgroup$
3
  • $\begingroup$ stupid question, in (11), in 1D case, when x=z goes to infinity, left side of the equation remains one, but from (12) and (13), it looks like all eigenfunctions go to zero due to the exponential term? i.e., the 'diagonal' of the kernel should be the same value, regardless how large the x&z goes, but the eigenfunctions seem to diminish when x&z goes large, how come? $\endgroup$
    – QnA
    Commented Nov 27, 2023 at 4:50
  • $\begingroup$ you can check numerically that sum over $n$ gives 1 for $x=z$; each term is small, but there are infinitely many terms... $\endgroup$
    – Carlo Beenakker
    Commented Nov 27, 2023 at 11:34
  • $\begingroup$ Thanks! I just checked, and the eigenfunction expansion matches the Gaussian kernel well locally around x=z=0, but then they go to zero pretty fast before I can come up with enough higher order Hermite terms. Sort of like Taylor expansion, locally matches very well, but requires much more terms for it to work further out. $\endgroup$
    – QnA
    Commented Nov 28, 2023 at 0:01

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .