I'm reading this paper. In it there is the following argument (see page 240).
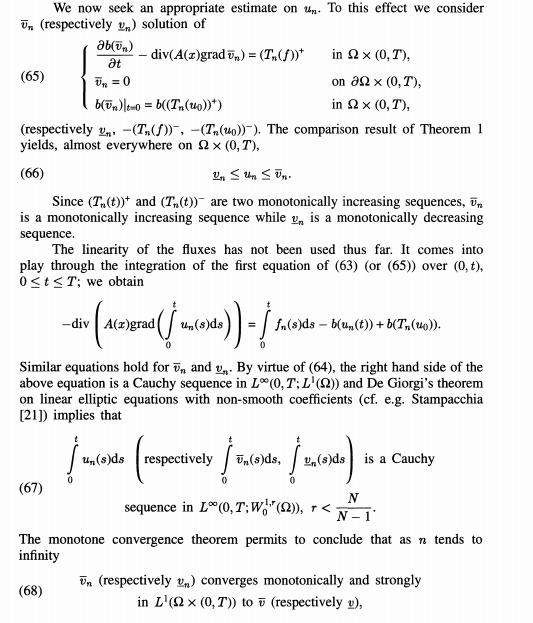
Firstly, what precisely does the author mean by the displayed equation after 66? The PDE in (65) only holds weakly.. $b(\overline{v}_n)$ is only in $H^1(0,T;H^{-1})\cap L^\infty(0,T;L^s)$ and $\overline{v}_n \in L^2(0,T;H^1)$. He just integrates it in time.. I don't follow.
Secondly, I am lost with the spaces when he says that $\int_0^T \overline{v}_n(s)\;ds$ is a Cauchy sequence in the space $L^\infty(0,T;W^{1,r}_0)$. And then he uses the monotone convergence theorem to say that $\overline{v}_n$ convreges strongly in $L^1(\Omega \times (0,T))$ to some $\overline{v}$. Any explanation appreciated.
I posed this here on MSE but did not receive an answer.
