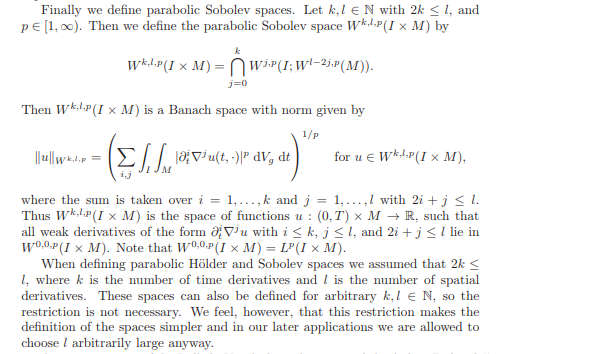
For parabolic sobolev spaces I follow the following definition:
According to this definition, we have that $W^{1,1,2}(I \times \Omega)=L^2(I; W^{1,2}(\Omega)) \cap W^{1,2}(I; W^{-1,2}(\Omega))$
Now my question is: If we have a function such that $f \in W^{1,2}(I; L^2(\Omega))$ with, in addition, $ \nabla f(x,\cdot) \in L^2(I;L^2(\Omega))$, can we claim that $f \in W^{1,1,2}(I \times \Omega)$?
Instinctively I would say yes, but I need a math confirmation.
Any hint or help is much appreciated.
Thanks in advance!