8
$\begingroup$

Let $$g(x) := \sqrt{1+x^2},$$ and $$h(x) := g^{-3/2}(x) \exp(-i2\pi g(x)).$$

I can observe that the Fourier transform $|H(f)|$ is almost flat if $|f|<1$, and $H(f)\approx 0, \; |f|>1$. This observation is important for my work, but I cannot understand why it happens. I tried for quite some time to figure out why, but failed. It basically means that $h(x)$ is a $\sin(x)/x$ function.

Improve this question
$\endgroup$
6
  • $\begingroup$ There may be a link with curvature, as the curvature of a $ C^{2} $ function $ f $ at $ x $ is expressed as $ f''(x).(1+f'(x)^2)^{-3/2} $, if I remember correctly. $\endgroup$
    – Sylvain JULIEN
    Commented Apr 28, 2017 at 17:24
  • 4
    $\begingroup$ What is $f$? What is $H(f)$? $\endgroup$
    – Nik Weaver
    Commented Apr 28, 2017 at 17:26
  • 5
    $\begingroup$ I think the OP is using $f$ for the variables many of us will normally call $\xi$, and $H$ the function many of us will write $\hat{h}$. $\endgroup$
    – Willie Wong
    Commented Apr 28, 2017 at 17:52
  • $\begingroup$ In the question, do you really mean $g^{-3/2}$ or $g^{-3}$? $\endgroup$
    – Willie Wong
    Commented Apr 28, 2017 at 17:54
  • 1
    $\begingroup$ This reminds me of something I read the other day (don't remember now where or who wrote it), where the author made the point that, contrary to what most mathematicians think, one cannot use arbitrary symbols for mathematical quantities. For example, while one may well call a function $f(x)$, denoting it by $x(f)$ instead immediately makes any further analysis impossible. $\endgroup$
    – Christian Remling
    Commented Apr 28, 2017 at 22:10

1 Answer 1

Reset to default
6
$\begingroup$

The Fourier transform $H_p(f)$ of $h_p(x)=g^{-p}(x)\exp[-2\pi ig(x)]$, with $g(x)=\sqrt{1+x^2}$ has a closed form expression for $p=1$: $$H_{1}(f)=\int_0^\infty h_{1}(x)\cos(2\pi f x)dx=K_0\left[2\pi\sqrt{f^2-1}\right],$$ see page 17 of Erdelyi's "Tables of Integral Transforms" (Volume I).

The Fourier transform of $1/\sqrt g$ is also a Bessel function, $$G(f)=\int_0^\infty g^{-1/2}(x)\cos(2\pi f x)dx=\frac{(\pi/f)^{1/4}}{\Gamma(\tfrac{1}{4})}K_{1/4}(2\pi f).$$

The key thing to note at this point is that $G(f)$ is basically a broadened delta function. The function $H_{1}$ if real for $f>1$ and decays rapidly to zero. This is unaffected by the convolution with $G$. For small $f$ there is a plateau at $|K_0(2\pi i)|=0.4992$, not exactly $1/2$ but close.

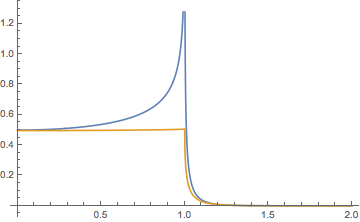
Plot of $|H_{1}(f)|$ (blue) and $|H_{3/2}(f)|$ (gold).

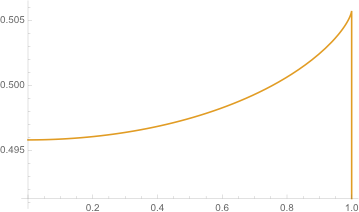
Plot of $|H_{3/2}(f)|$ for $f<1$, to show that it is almost but not quite flat, and almost but not quite $1/2$ for $f\rightarrow 0$. The sharp peak at $f=1$ that was present in $|H_{1}(f)|$ has been greatly suppressed by the convolution with $G$.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ From your graph it seems that $|H_{3/2}|$ is actually flat in the region $|f|\le1$. Can you confirm numerically? $\endgroup$
    – Piero D'Ancona
    Commented Apr 29, 2017 at 6:17
  • 2
    $\begingroup$ it is flat within 1%, I've added a detailed plot to show that. $\endgroup$
    – Carlo Beenakker
    Commented Apr 29, 2017 at 9:08
  • $\begingroup$ If we only know the behavior of $|H_a|, |H_b|$ (and not of the functions themselves), we can't really conclude much about $|H_a * H_b|$. $\endgroup$
    – Christian Remling
    Commented Apr 29, 2017 at 17:36
  • $\begingroup$ Thanks a lot! But doesn't it play a role here how the Fourier transform is defined? You seem to do the cosine-transform, but I intended $$H(f) = \int_{-\infty}^{\infty} h(x)\exp(-2\pi i f x)\mathrm{d}x.$$ $\endgroup$
    – Nicki
    Commented May 2, 2017 at 7:20
  • 1
    $\begingroup$ your $h(x)$ is even in $x$, so it's a cosine transform $\endgroup$
    – Carlo Beenakker
    Commented May 2, 2017 at 8:34

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .