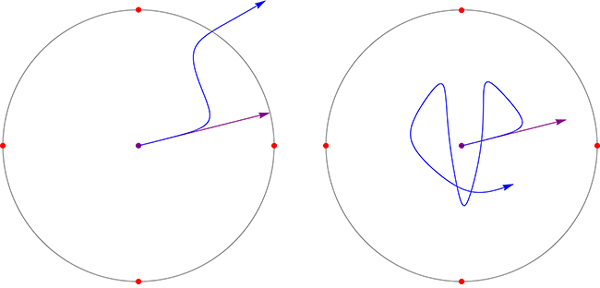
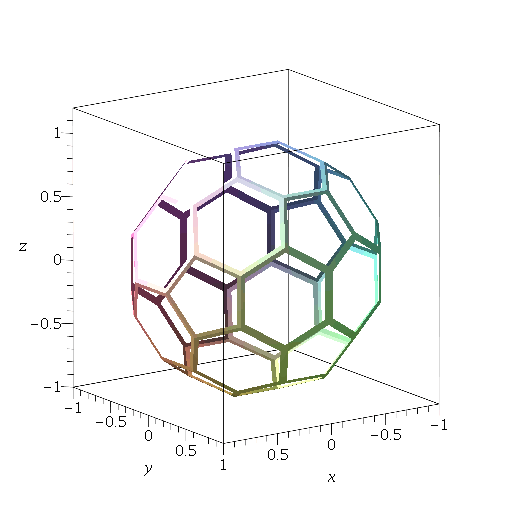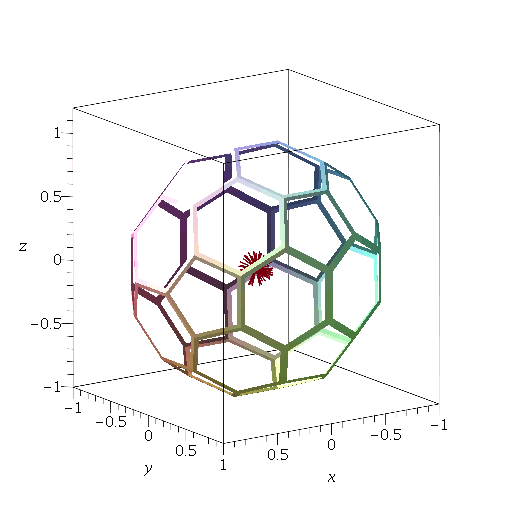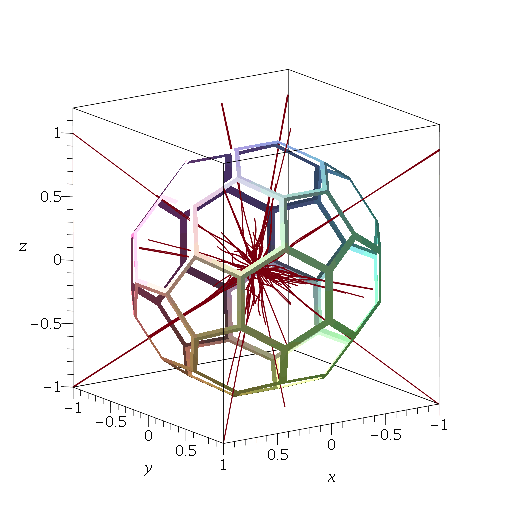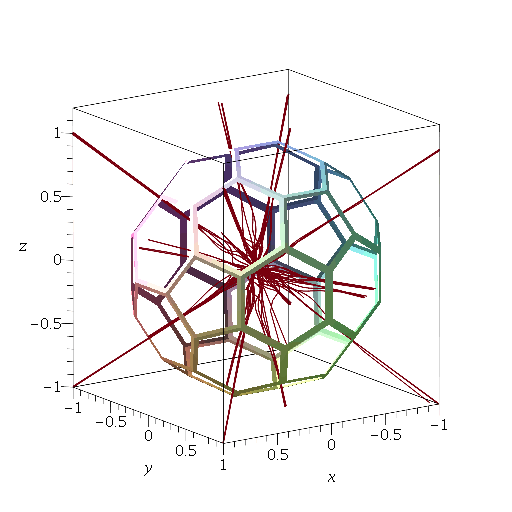I will describe the question first in 2D, but my interest is in $\mathbb{R}^3$. An electron $x$ will shoot from the origin along an initial vector $v$. You know the speed $|v|$ but not the direction. Your task is to arrange a minimum number of point-charge electrons at fixed locations on an origin-centered unit circle so that, no matter in which direction $v$ is pointing, $x$ cannot escape the disk. All the charges are equal, and repel each other via inverse-square Coulomb force.
Below there are four charges on the circle. The left electron escapes, the right, which is shot along the same direction but with a lesser speed, does not. (Of course aiming $v$ along a diagonal is the best escape strategy in this configuration.)
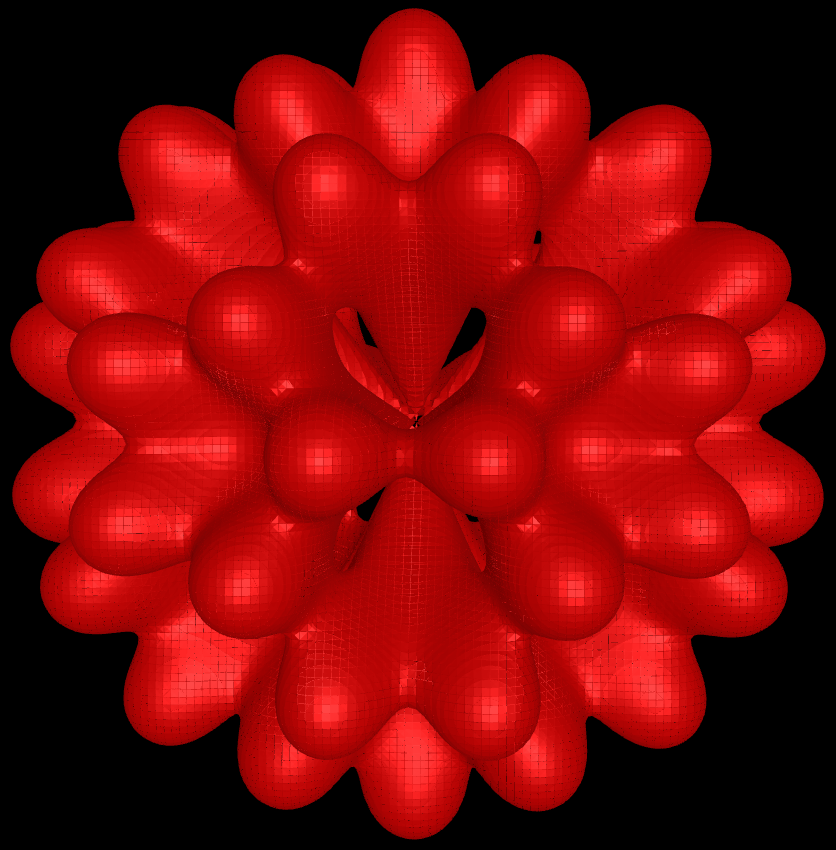
Now here is my question. Consider the same problem in $\mathbb{R}^3$. Given $|v|$, arrange fixed electrons on a sphere to cage-in the electron $x$ starting from the origin with velocity $v$ in any direction. It seems natural to think that an optimal arrangement is a type of disk-packing on a sphere, for example, the solutions to the Thompson problem or the Tammes problem. (Here I am mining an earlier MO question.)

(Image from Paul Sutcliffe.)
Q. Is an optimal electron cage for a given speed $|v|$ a configuration that minimizes electrostatic potential? Or maximizes the minimum distance between any pair of electrons, i.e., an optimal disk packing on the sphere?
Answered by Robert Israel: No such cage is possible in $\mathbb{R}^3$!
($\mathbb{R}^2$ is rather different and not a reliable guide to $\mathbb{R}^3$.)