Technically, there are best known configurations with pentagons and even with hexagons -- if we allow these polygons to loop around multiple faces. Such configurations may be read from the Wikipedia list via the presence of a proper ($C_n$) five- or sixfold rotational symmetry element in the listed point group. For example, the rigorously proved solution of a regular icosahedron for twelve points has a pentagon looping around the five faces touching each vertex. All configurations listed by Wikipedia having a proper $n$-fold symmetry axis with $n\in\{5,6\}$ have a number of points $\equiv2\bmod n$ (such as $7$ points with fivefold symmetry or $14$ with sixfold symmetry), indicating that the polygonal loops surround multiple faces with a central vertex at both poles of the rotational axis. Most commonly, the pentagons or hexagons are the bases of pyramids whose triangular lateral faces (instead of the base) are among the faces of the polyhedron.
Of some relevance for the absence of single faces having five or more sides is the instability that emerges from placing too many points on the edge of a hemisphere. On a hemisphere of unit radius, four identically signed unit charges equally spaced around the edge give a net repulsive energy of $1+2\sqrt2\approx3.83$ units; if we move one charge to the top of the hemisphere and redistribute the rest equally around the edge the repulsive energy becomes $(3/2)\sqrt2+\sqrt3\approx3.85$ units. Thus the square configuration is (barely) stable in this case (we must use a section larger than a hemisphere to realize the familiar tetrahedral pattern). With five points, however, the pentagonal planar configuration gives $\approx6.88$ units of repulsive energy while the square pyramid, with a point at the apex and thus only square and triangular faces, gives $\approx6.66$ units -- the square pyramid has become more stable. Wikipedia configurations that include five points on a great circle (or any small circle, for that matter) are possible only because the poles are plugged up by additional points.
We could make the pentagonal arrangement more stable by making the face smaller, so that the apex of the proposed square pyramid is not pushed as far out of the plane. But then there would be more points on the rest of the sphere which would tend to push the repulsion that forms the pyramid "from behind". We can see this destabilization effect by considering the case of ten points using two alternate configurations: a pentagonal antiprism (PA) in which each hemisphere has a pentagon of points, and a gyroelongated square dipyramid (GESD) in which each hemisphere has the five points in the form of a square pyramid. The figure below shows the resulting comparison.
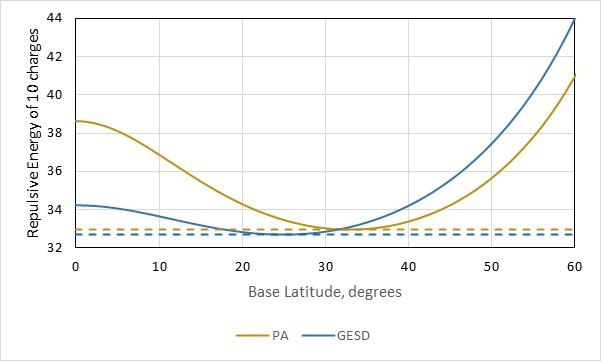
The GESD configuration is seen to win out over PA (the minimum shown for that configuration is actually the best known for ten charges), so the pentagonal faces are unstable. We can see why from the comparison of base latitudes: 34° for PA versus 25° for GESD. Thus in the PA configuration, the repulsions from "behind" each hemisphere by the other pushes the points to a higher and smaller latitude circle where as many as five points are pushed rather uncomfortably close together. Forming the pyramidal configuration lessens this unfavorable interaction and allows the points to spread out more optimally. This result favoring pyramidalization is also apparent from the known optima for six or seven points per hemisphere as well. This goes along with the earlier noting of solutions with pentagons and hexagons as multi-faced loops (bases of pyramids) instead of single faces.
It takes computer calculations to balance these effects in more complex situations, but the consistent result is similar to that above: the destabilizing effect of the outside points tends to win out over the face interior becoming more nearly planar. So a single pentagonal (or more-sided) face is never stabilized and the barely stable square configuration holds up only in a relatively few favorable cases.

