Suppose one has $k$ unit-radius disks, and the goal is to hide them inside a disk of radius $R \gg k$. The detection probes are rays along a line. (Think of the disks as tumor cells, and the rays as radiation.)
Q1. What is the optimal hiding configuration for $k$ disks?
That is, how can the $k$ disks be arranged to be difficult to detect by ray probes?
I believe the probability of detection from, say, random ray probes, would be proportional to the integral, over all directions $\theta \in [0,\pi)$, of the measure of the projection/shadow of the disks in direction $\theta$. So I seek a configuration with the smallest mean shadow.
For example, it seems that for $k=3$, the obvious is the optimal configuration:
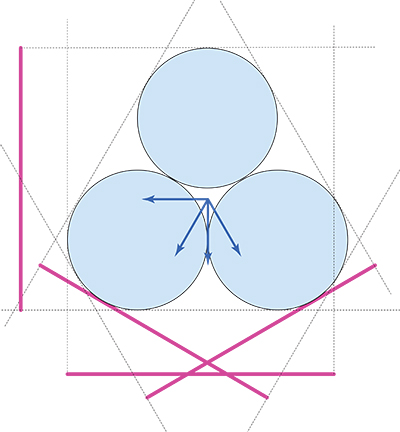
But it is unclear to me if the optimal configuration under this projection-based integral measure is identical to, say, (a) the optimal packing of $k$ disks in a surrounding disk of minimal radius, or (b) the packing of $k$ disks with the minimum area convex hull.
Q2. Is the projection integral measure identical to any of the well-known, previously studied disk packing constraints?
Or perhaps what I am suggesting has already been studied on its own, in which case a pointer would be appreciated—thanks!
