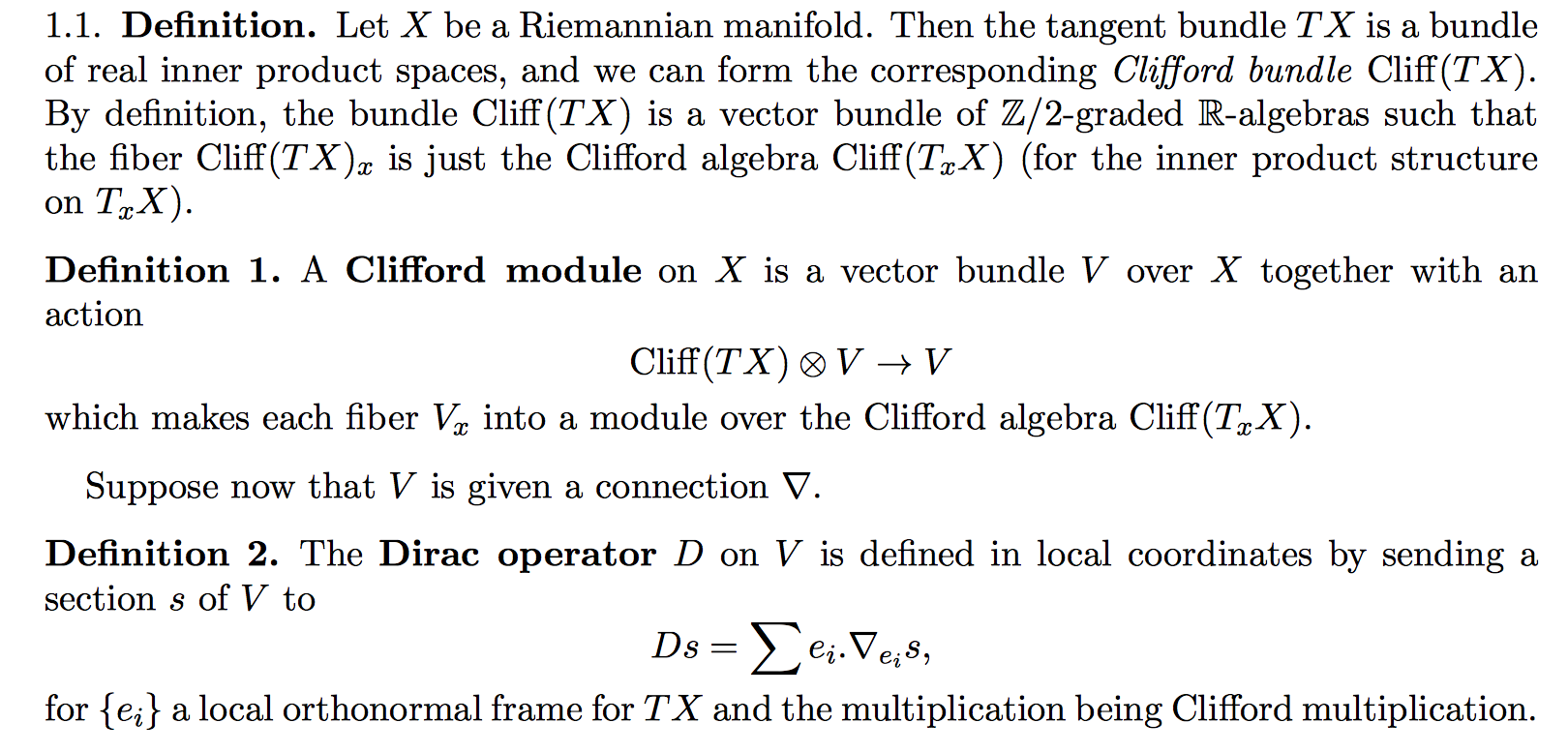
Let $(M,g)$ be an orientable pseudo-riemannian manifold. Each tangent space $T_xM$ is a pseudo-euclidean space and hence has an associated Clifford algebra $CL(T_xM)$, which is the fibre at $x\in M$ of the Clifford bundle $Cl(TM)$. If the manifold is spin (a topological condition which says that the oriented orthonormal frame bundle lifts to a spin bundle) then it admits a vector bundle $\Sigma$ whose fibre at $x$ is an irreducible Clifford module of $Cl(T_xM)$. This means that we have a bundle map
$$Cl(TM) \times \Sigma \to \Sigma$$
given fibrewise by the Clifford action of $Cl(T_xM)$ on $\Sigma_x$.
(Please allow me the notational abuse of confusing bundles with their sections, to save me some writing.)
Because we have a metric, we have musical isomorphisms
$$\flat: TM \to T^*M \qquad\text{and}\qquad \sharp: T^*M \to TM~,$$
the latter of which can be composed with the above Clifford action to arrive at
$$Cl(T^*M) \times \Sigma \xrightarrow{\operatorname{cl}} \Sigma~.$$
The Spin group $Spin(T_xM)$ is contained in $Cl(T_xM)$ and hence acts on $\Sigma_x$ by restriction. This representation need not be irreducible; although if $M$ is odd-dimensional it is. This allows us to view $\Sigma$ as a bundle of spinors, associated to a (not necessarily irreducible) spinor representation of the spin group. In fact, it is an associated vector bundle of the spin bundle.
The Levi-Civita connection on $TM$ defines an Ehresmann connection on the bundle of oriented orthonormal frames. That connection lifts to a connection on the spin bundle and hence induces a Koszul connection on $\Sigma$, called the spin connection. We thus get a bundle map
$$ \Sigma \xrightarrow{\nabla} T^*M \otimes \Sigma $$
The Dirac operator is now simply the composition
$$ \Sigma \xrightarrow{\nabla} T^*M \otimes \Sigma \xrightarrow{\operatorname{cl}} \Sigma~.$$
Thus the domain and range are the sections of $\Sigma$, the so-called spinor fields.
If $M$ is even-dimensional (and depending on signature perhaps after complexifying) then one can refine this. Under spin, $\Sigma$ splits into eigenbundles of the Clifford action by the volume form:
$$ \Sigma = \Sigma_+ \oplus \Sigma_-$$
Then $\nabla : \Sigma_\pm \to \Sigma_\pm$, but
$$ \operatorname{cl}: T^*M \otimes \Sigma_\pm \to \Sigma_\mp$$
whence now the Dirac operator, defined in the same way, breaks up into two maps $\Sigma_\pm \to \Sigma_\mp$.
Added
I'm adding more details to answer Igor's question. If we are in signature $(s,t)$, with $n=s+t$, being the dimension, then the answer depends on $s-t \mod 8$:
- $s-t = 0 \mod 8$: $\Sigma$ is real and $\Sigma = \Sigma_+ \oplus \Sigma_-$, where $\Sigma_\pm$ are real.
- $s-t = 1 \mod 8$: $\Sigma$ is complex but has a spin-invariant real structure: it does not split.
- $s-t = 2 \mod 8$: $\Sigma$ is quaternionic and it splits if we complexify: $\Sigma_{\mathbb{C}} = \Sigma_+ \otimes \Sigma_-$, with $\Sigma_\pm$ complex conjugates.
- $s-t = 3 \mod 8$: $\Sigma$ is quaternionic and remains so under spin: it does not split.
- $s-t = 4 \mod 8$: $\Sigma$ is quaternionic and it splits $\Sigma = \Sigma_+ \oplus \Sigma_-$, where $\Sigma_\pm$ are also quaternionic.
- $s-t = 5 \mod 8$: $\Sigma$ is complex but has a spin-invariant quaternionic structure: it does not split.
- $s-t = 6 \mod 8$: $\Sigma$ is real and if we complexify then it splits $\Sigma_{\mathbb{C}} = \Sigma_+ \otimes \Sigma_-$, with $\Sigma_\pm$ complex conjugates.
- $s-t = 7 \mod 8$: $\Sigma$ is real, does not split and remains real.