4
$\begingroup$

Let $p,q,r$ be integers with $r\ge2$; let $f$ be a polynomial of the form $f(X) = g((X+1)^r)$, which is not a $q$-th power. Let $\omega$ be a $p$-th root of unity.

Prove or disprove that the polynomial $f(X)f(\omega X)\cdots f(\omega^{p-1}X)$ is also not a $q$-th power.

NOTE: The special case $p=q=r$ is Lemma 5 in Samuel White, "The group generated by x->x+1 and x->x^p is free", J. Algebra 118 (1988) 408--422; he says that "the idea is attributed to George Bergman". I wondered how general the statement is.

Improve this question
$\endgroup$
4
  • 2
    $\begingroup$ Where does this question come from? $\endgroup$
    – Igor Rivin
    Commented Sep 1, 2014 at 14:51
  • 1
    $\begingroup$ The special case p=q=r is Lemma 5 in [White, "The group generated by x->x+1 and x->x^p is free", J. Algebra 118 (1988) 408--422]; he says that "the idea is attributed to George Bergman". I wondered how general the statement is. $\endgroup$
    – grok
    Commented Sep 1, 2014 at 15:11
  • 5
    $\begingroup$ Cool! Often, questions starting with "Show that..." are homework, so to avoid misunderstandings, might be better to give some back story (as in your comment). $\endgroup$
    – Igor Rivin
    Commented Sep 1, 2014 at 15:49
  • 4
    $\begingroup$ @grok, it is best to put information asuch as that you gice in the comment in the question body itself :-) $\endgroup$
    – Mariano Suárez-Álvarez
    Commented Sep 1, 2014 at 16:17

1 Answer 1

Reset to default
3
$\begingroup$

A family of counterexamples is defined as follows:

$r=2$,

$p_1\ge 2, \quad p_2\ge 3, \quad d_1 \perp p_1, \quad d_2 \perp p_2$,

$a=\dfrac{\tan(\pi d_1/p_1)-\tan(\pi d_2/p_2)}{\tan(\pi d_1/p_1)+\tan(\pi d_2/p_2)} \qquad b=\dfrac{2\tan(\pi d_1/p_1)\tan(\pi d_2/p_2)}{\tan(\pi d_1/p_1)+\tan(\pi d_2/p_2)}$,

(with $a=1, \;b=2\tan(\pi d_2/p_2)\;$ if $\;p_1=2$)

$g(X)=(X-(a+i b)^2)(X-(a-ib)^2)=X^2-2(a^2-b^2)X+(a^2+b^2)^2$,

$p=\text{lcm}(p_1, p_2), \quad q=2$,

$\prod_{j=0}^{p} f(\omega^j X)=(X^{p}-(a-1+ib)^{p})^2(X^{p}-(-a-1+ib)^{p})^2$.

Here is a picture of the four roots of $f$ in the complex plane:

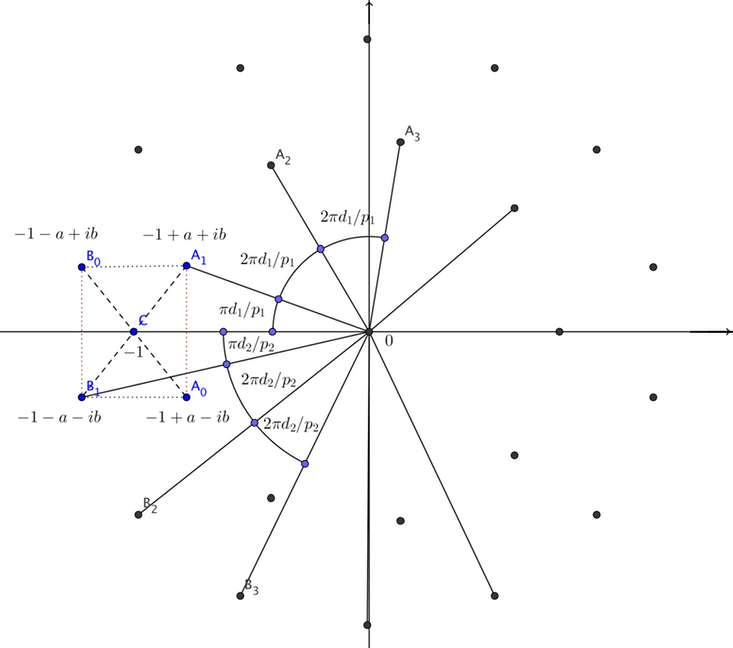
enter image description here

When $p_1\!=\!p_2$, $\;g$ is a square and the formulas simplify to the original answer:

$r\!=\!2, \; p\!\ge\!3, \; b\!=\!\tan(\frac{\pi}{p}), \; g(X)\!=\!X\!+b^2, \; q\!=\!2,\; \prod\limits_j f(\omega^j X)\!=\!\big(X^p\!-(-1\!+ib)^p\big)^2$.

When $\;a=\pm b\;$ the example simplifies to: $\; r=4, \; g=X+4a^4, \quad$ but the only such cases I know of are for $\;(d_1, p_1, d_2, p_2) \!=\! (3, 8, 1, 8)\;$ and $\;\prod f(\omega^j X)=(X^8-(\frac{1+i}{\sqrt{2}}-1)^8)^2(X^8-(\frac{-1+i}{\sqrt{2}}-1)^8)^2$,$\;\;$ or $(d_1, p_1, d_2, p_2) \!=\! (1, 6, 1, 12)\;$ and $\;\prod f(\omega^j X)=(X^{12}-(\frac{-1+i}{1+\sqrt{3}}-1)^{12})^2(X^{12}-(\frac{1+i}{1+\sqrt{3}}-1)^{12})^2\;$.

Ignoring trivial derivations (i.e. replacing $g$ with products of the "primitive" ones above), I'd be very surprised if any other examples esisted - but a proof of that looks to me extremely hard.

Improve this answer
$\endgroup$
2
  • $\begingroup$ There is more case for $r=4$, corresponding to $(d_1, p_1, d_2, p_2)=(7, 12, 1, 6)$, for which $a=b=(\sqrt{3}+1)/2$. $\endgroup$
    – Yaakov Baruch
    Commented Jan 8, 2015 at 10:11
  • $\begingroup$ I apologize for bumping up this answer one last time. I wanted to make the attached image clearer. $\endgroup$
    – Yaakov Baruch
    Commented Jan 8, 2015 at 19:31

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .