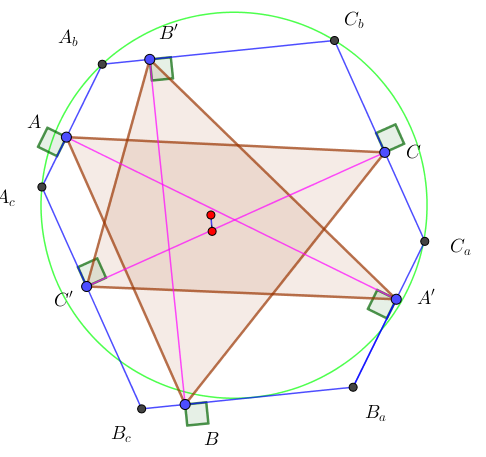
Let $\triangle ABC$ and $\triangle A'B'C'$ be two triangles. The line through $A$ and perpendicular to $AA'$ meets the line through $B'$ and perpendicular to $BB'$ at $A_b$; The line through $A$ and perpendicular to $AA'$ meets the line through $C'$ and perpendicular to $CC'$ at $A_c$. Define $B_c, B_a, C_a, C_b$ cyclically then six points $A_b$, $A_c$, $B_c$, $B_a$, $C_a$, $C_b$ lie on a circle if only if $ABC$ and $A'B'C'$ have the same centroid.

This circle have a property: The main dialog are equal: $A_bB_a=B_cC_b=C_aA_c$

Question: Is this result know?

This result is a generalization of the Floor van Lamoen circle: Six circumcenters lie on a circle, and also is a generalization of Elias M Hagos's six orthocenters lie on a circle

See also:

• Geogebra applet

• Geogebra another

• The paper