There are some new results above construction of the Golden ratio. Examples Odom's construction associated with an equilateral triangle, Dao's construction, Tran's construction associated with an equilateral triangle and right triangle, Dao-Ngo-Yiu's construction associated with Isosceles Triangle and Tran's constructionassociated with arbitrary triangle here and here
I proposed a new construction of Golden ratio in arbitrary triangle as follows:
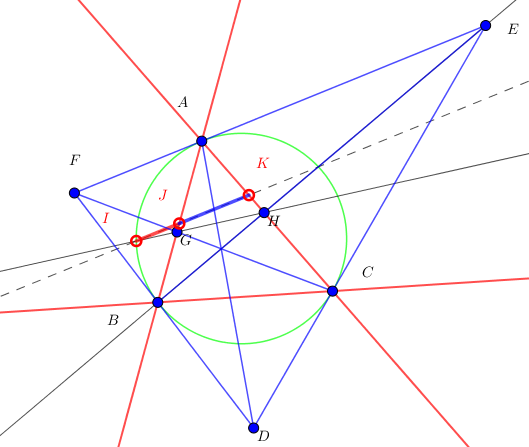
Let $ABC$ be arbitrary triangle and $DEF$ is the its tangential triangle. Let $CF$ meets $AB$ at $G$ and $BE$ meets $AC$ at $H$, let $GH$ meets the circumcircle at $I$. A line through $I$ and parallel to $EF$ meets $AB, AC$ at $J, K$ then $$\frac{JK}{JI}=\frac{\sqrt{5}+1}{2}$$
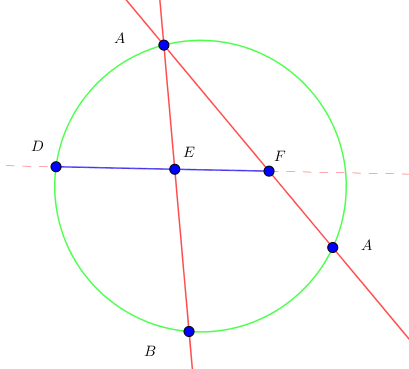
My question: Let $ABC$ be arbitrary triangle and $k$ is arbitrary positive real number (assume that we have a ruler with absolute accurate), how can construct points $D$ in the circumcircle $(ABC)$, $E$ in $AB$, $F$ in $AC$ such that $D, E, F$ are collinear and $$\frac{EF}{ED}=k$$
See also: