Special case of Golden ratio as a property of conic section (is it known?) as follows:
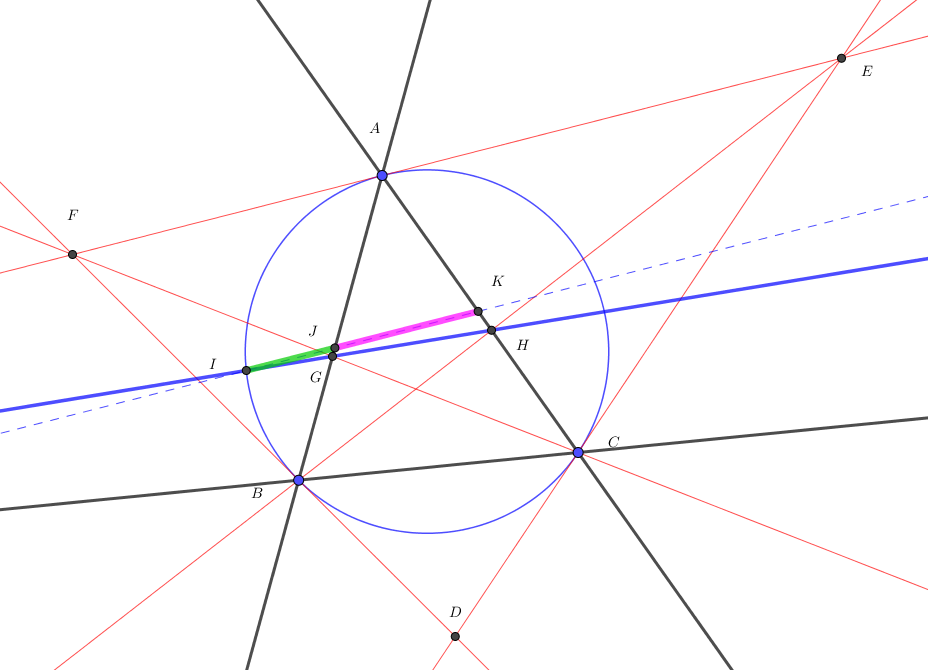
Let $ABC$ be arbitrary triangle and $DEF$ is the its tangential triangle. Let $CF$ meets $AB$ at $G$ and $BE$ meets $AC$ at $H$, let $GH$ meets the circumcircle at $I$. A line through $I$ and parallel to $EF$ meets $AB$, $AC$ at $J$, $K$ then $$\frac{JK}{JI}=\frac{\sqrt{5}+1}{2}$$
Open problem:
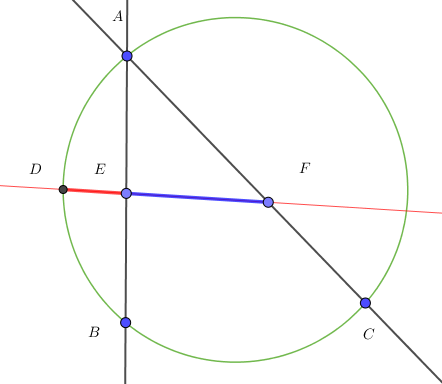
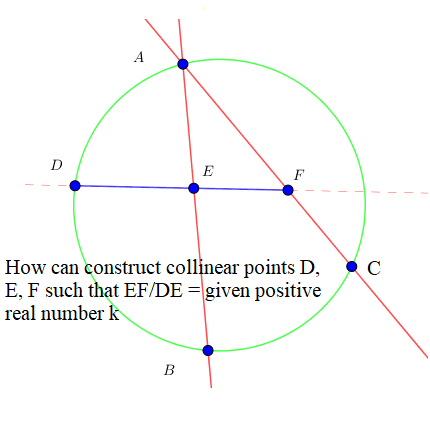
Cramer–Castillon problem being very difficult problem. Related to the Cramer–Castillon problem configuration, I posed a problem as follows:
Let $ABC$ be arbitrary triangle and let three collinear points $E'$, $F'$, $D'$ such that $\frac{E'F'}{E'D'}=k$ where $k$ is positive real number. How can construct points $D$ in the circumcircle of $ \triangle ABC$, $E$ in $AB$, $F$ in $AC$ such that $D, E, F$ are collinear and $\frac{EF}{ED}=k$ ?