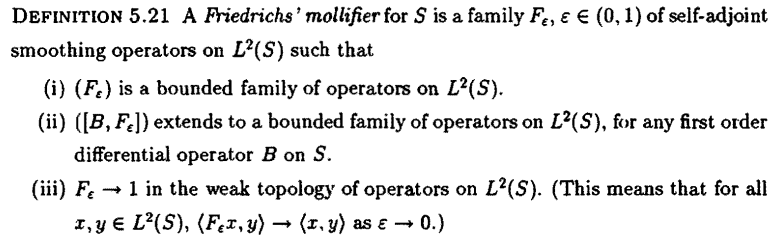
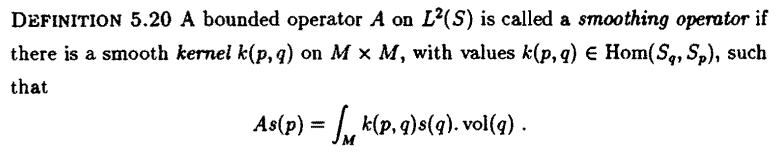$\renewcommand{\epsilon}{\varepsilon}$The following is from John Roe's book Elliptic operators, topology and asymptotic methods. $S$ is a vector bundle on a compact manifold $M$, but I think for my question it is sufficient to assume that $S = M \times \mathbb{C}$. I will probably be happy with an answer that only deals with the $L^2$ and Sobolev spaces of periodic functions on $\mathbb{R}^n$.

Later in the book the author claims that a Friedrichs' mollifier $(F_\epsilon)$, as well as $([B, F_\epsilon])$ for any first order differential operators $B$, are bounded families of operators on any Sobolev space $W^k$ (i.e. $W^{k, 2}$).

How could you prove this?

Everything that I could find online about this seems to talk about some "Friedrichs' Lemma", which I know as the statement that for a first-order smooth differential operator on an open subset of $\mathbb{R}^n$ and $v \in L^2(\mathbb{R}^n)$ $$ [P, S_\varepsilon] v \to 0 \text{ in } L^2 \quad\text{for } \varepsilon \to 0 $$ where $S_\varepsilon$ is a family of standard mollifiers. This certainly seems like it might be related to the question, but I don't really know how to use it.