Imagine a decreasing sequence of (positive) radii $r_1 > r_2 > r_3 > \cdots$ and a series of nested circles $C_1 \supset C_2 \supset C_3 \supset \cdots$ with these radii, initially each resting on the $x$-axis tangent at $(0,0)$. Each is assigned a rolling speed $s_1, s_2, s_3, \ldots$, the amount of arc length that $C_i$ rolls on the inside of $C_{i-1}$ per unit time. (Positive $s_i$ represents clockwise spinning of $C_i$; negative, counterclockwise.) $C_1$ rolls on the $x$-axis, which can be considered $C_0$.
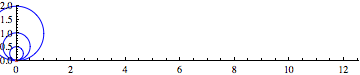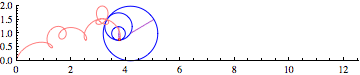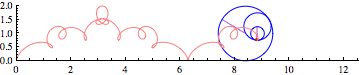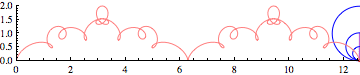
Here is an example, with $(r_1, r_2, r_3)=(1, \frac{1}{2}, \frac{1}{4})$
and $(s_1, s_2, s_3)=(1,2,3)$, with the track of a point on
the third circle highlighted:

Call the curve that is the track of the $n$-th circle
a rolling epicycle curve, or just a rolling curve.
My question is:
Q1. What is the class of functions on some interval $[0,X]$ that can be approximated by some rolling curve?
Say that a function $f(x)$ is approximated by a rolling curve if, for any $\epsilon > 0$, a curve may be found that remains within an $\epsilon$-tube around $f$. (One can easily substitute other reasonable definitions of approximation.) To be specific, we could insist that $f(0)=0$ and the rolling curve tracks the innermost circle's point that initially touches $(0,0)$.
Here is a more random example of four circles of
radii $(1, \frac{3}{4}, \frac{2}{3}, \frac{1}{2})$, with
green tracking the fourth circle:

There is considerable flexibility, but it seems difficult
to control. To pose a more specific version of Q1:
Q2. Can a straight line through $(0,0)$ be approximated on a given interval $[0,X]$?
Wondering about the power of Ptolemaic epicycles led me to this question (although I realize the rolling constraint renders my question different). Thanks for insights!
Addendum. As per J.M.'s request, here is an animated GIF for
the three-circle example (which may or may not animate, depending on your browser settings):