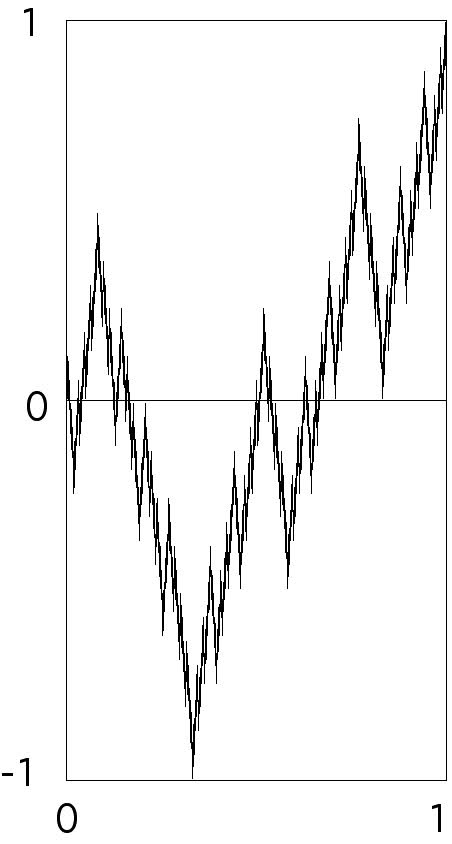
Coming from a specific field in algebraic geometry, I am a total noob in Fractal Theory and I'd like to learn it a bit. I hope I am tolerated for my maybe-trivial questions. I just read about the Weierstrass-Mandelbrot fractal (it's also simply called Weierstrass fractal using the Weierstrass function.. but there are dozens of Weierstrass functions so I'd rather call it "Weierstrass-Mandelbrot" function). The definition of this fractal is found in Wikipedia. I got easily impressed by it.
My question is whether there are nowhere differentiable continuous functions (between real numbers) whose graphs are not fractals? Is the WM function the easiest example of a nowhere differentiable continuous function?
The other question is quite basic (for experts probably). I have seen the definition of fractal in Wikipedia. This definition uses self-similarity. But in a reference of mine (from a lecture note) I get a definition that makes use of an inequality with Hausdorff-dimension and inductive dimension. Are these definitions equivalent or is the precise definition still under debate? (My reference suggests that the suggested definition was the former definition by Mandelbrot, and then this definition was changed as Mandelbrot fractals don't follow this definition.) A little enlightening would help :)

=)$\endgroup$