A delightful recent problem about disconnecting the plane by straight lines suggested me the following further question, that I can't resist to post.
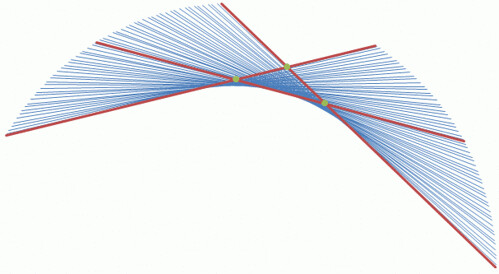
Let $\mathcal{F} $ be a countable family of straight lines, which is generic in the sense that no two of them are parallel, and no three are concurrent.
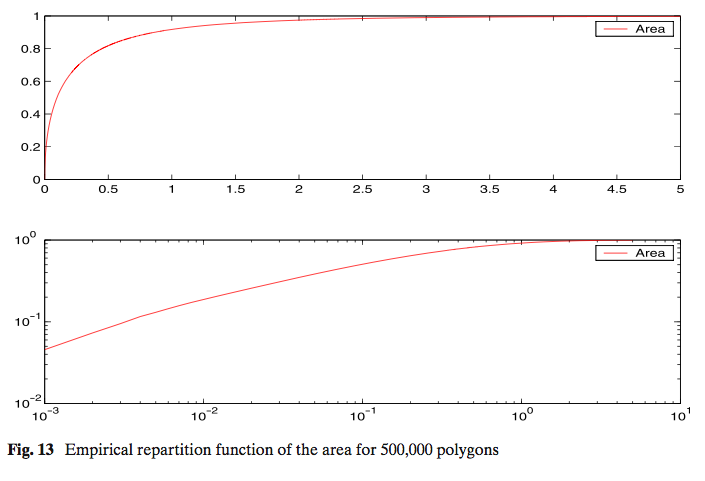
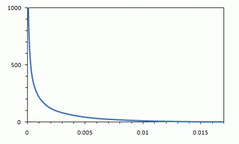
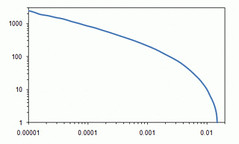
What can be said about the distribution of the areas of the polygons in which the plane is disconnected by $\mathcal{F} $?
After Yaakov Baruch's answer given in the linked question, we know that they can't be all equal. On the other hand, one can easily make examples where the areas are at least bounded below, and also examples where areas are bounded away from zero. But is there an example where all areas are between two positive constants? I tend to think there isn't any. More generally, what can we say about the distribution of these areas? (say, in an asymptotic sense, as limit of the distributions of the areas of the tiles within a ball of radius $R$).

springerlink.comin the comment above is broken. I'm also unable to find any snapshot saved on the Wayback Machine. $\endgroup$