Suppose that $f$ is a totally monotone function on $(0,\infty)$, so that $(-1)^n f^{(n)}\ge0$ for all $n=0,1,\dots$, $f(0+)\in(0,\infty)$, and $f(t)\sim\frac{1}{t^{\frac{3}{2}}}$ as $t\to\infty$. Can we prove that $g(t)=tf(t)$ has only one critical point over $(0,\infty)$?
1 Answer
Here is a counterexample:
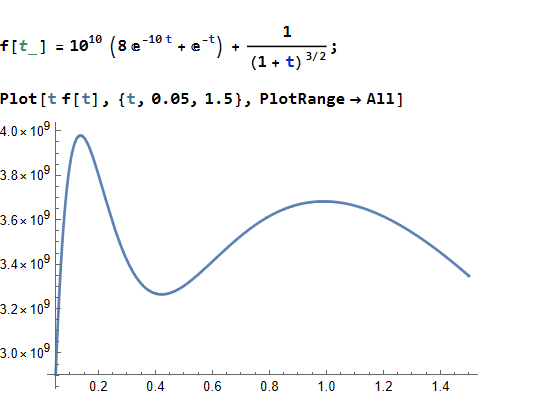
$$f(t)=10^{10} \left(8 e^{-10
t}+e^{-t}\right)+\frac{1}{(t+1)^{3/2}}$$
for real $t\ge0$.
Then $(-1)^n f^{(n)}\ge0$ on $[0,\infty)$ for all $n=0,1,\dots$. Also, $f(t)\sim \frac{1}{t^{3/2}}$ as $t\to\infty$.
Also, $g(0)=0$, $g(\frac1{10})\approx3.85\times10^9$, $g(\frac4{10})\approx3.27\times10^9$, $g(\frac{10}{10})\approx3.68\times10^9$, and $g(\infty-)=0$, so that $g(\frac1{10})>\max(g(0),g(\frac4{10}))$ and $g(\frac{10}{10})>\max(g(\frac4{10}),g(\infty-))$. So, there is a critical point of $g$ in each of the intervals $(0,\frac4{10})$ and $(\frac4{10},\infty)$. There also is a third critical point of $g$ in the interval $(\frac1{10},\frac{10}{10})$.
For an illustration, here is the graph $\{(t,g(t))\colon 0.05\le t\le1.5\}$:
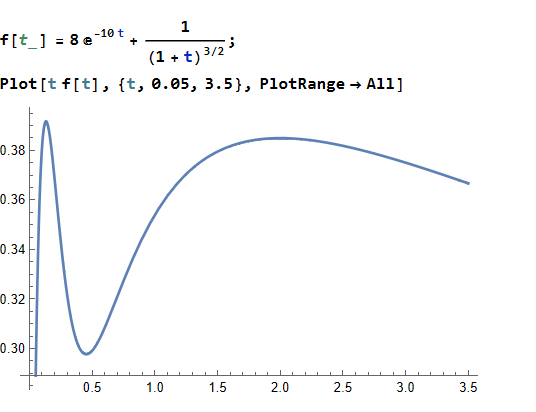
A simpler version of the previous example:
The idea here is the same as before: $8\approx10\approx\infty$.
-
$\begingroup$ Dear Pinelis, thank you so much. Do you have any idea that under what extra conditions $tf(t)$ have a single critical point? $\endgroup$– ErvandCommented Dec 9 at 8:45
-
1$\begingroup$ @Ervand : I think the totally monotonicity condition on $f$, involving the derivatives of $f$ of all orders, is hardly relevant here. What you need here is that $[(tf(t))'=]tf'(t)+f(t)$ change its sign only once on $(0,\infty)$. The latter condition involves only $f$ and $f'$, rather than the derivatives of $f$ of all orders. $\endgroup$ Commented Dec 9 at 15:02
-
$\begingroup$ Why the downvote? Is there anything wrong with this answer? $\endgroup$ Commented Dec 9 at 16:07
-
$\begingroup$ Yeah, I got your point, but what I have is just totally monotonicity of function f. I asked because there might be a subclass of completely monotone functions that satisfy this condition but it seems that there no clean criteria (in terms of total monotonicity) to isolate this subclass. By the way thanks for showing some counterexamples. $\endgroup$– ErvandCommented Dec 9 at 19:18