Finding an overtwisted disk in a contact surgery diagram

Question

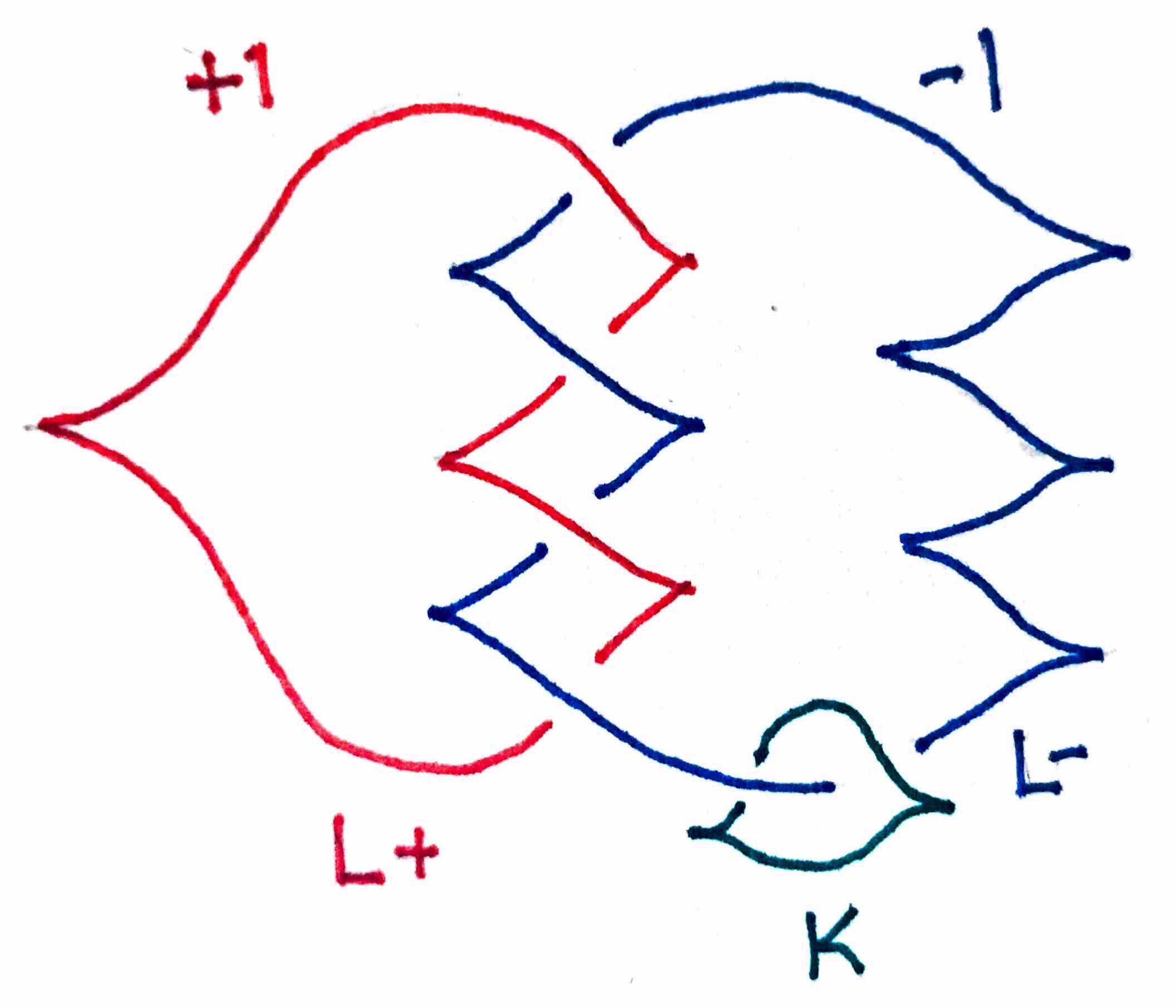
I've been reading through Surgery on Contact 3-Manifolds and Stein Surfaces by Ozbagci & Stipsicz and have been stuck on an exercise. Consider the following contact surgery diagram for $(S^3,\xi_{-1})$, where $\xi_{-1}$ is the [isotopy class of] overtwisted contact structure on $S^3$ with Hopf invariant $d_3(\xi_{-1}) = -3/2$.

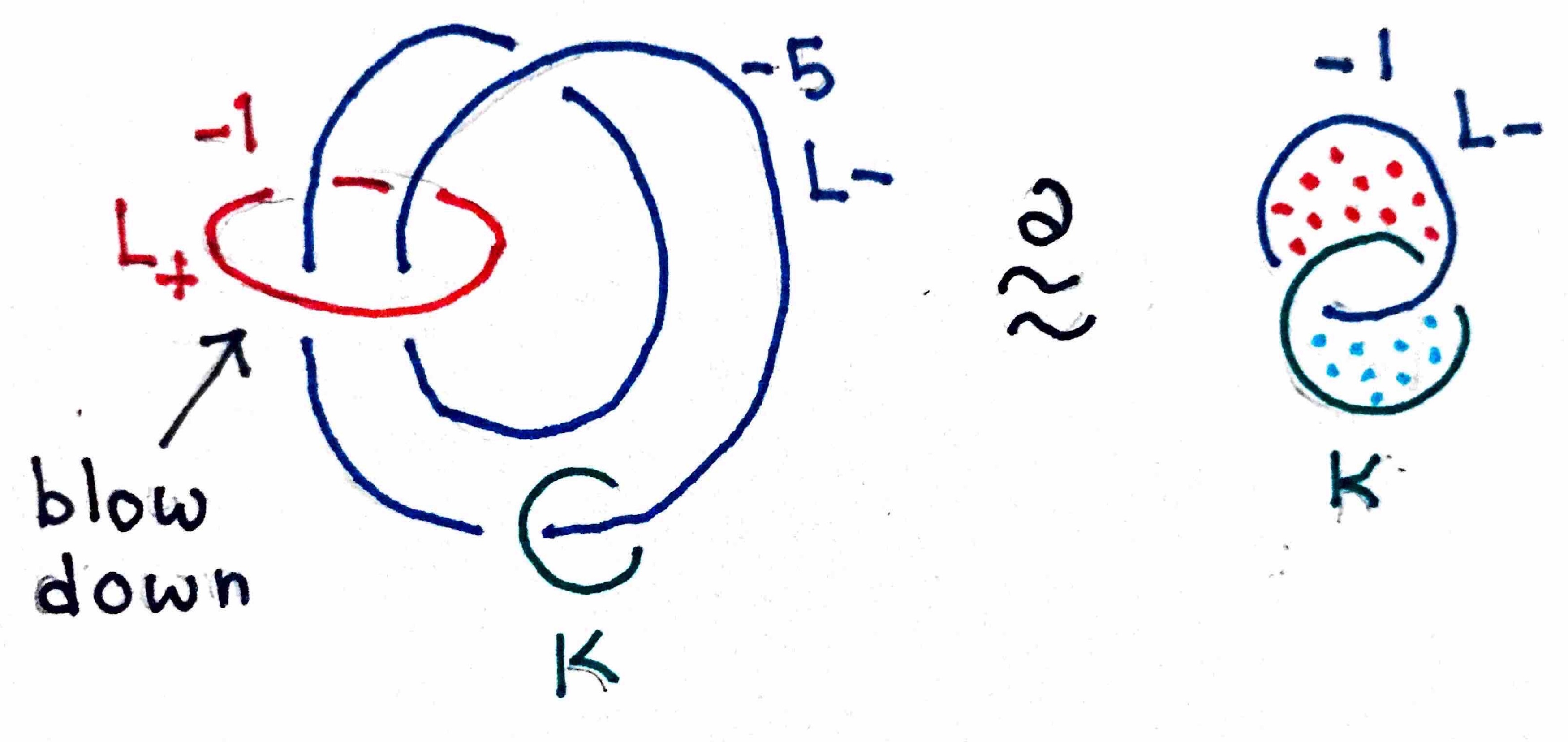
Above, surgery coefficeints are measured with respect to the contact framing. [With respect to the Seifert framing, the coefficients on $L_+$ and $L_-$ should be $-1$ and $-5$, respectively.] The problem is to find an explicit overtwisted disk in the above diagram. As another example, the surgery diagram below represents $(S^3,\xi_1)$ where $d_3(\xi_1) = 1/2$.

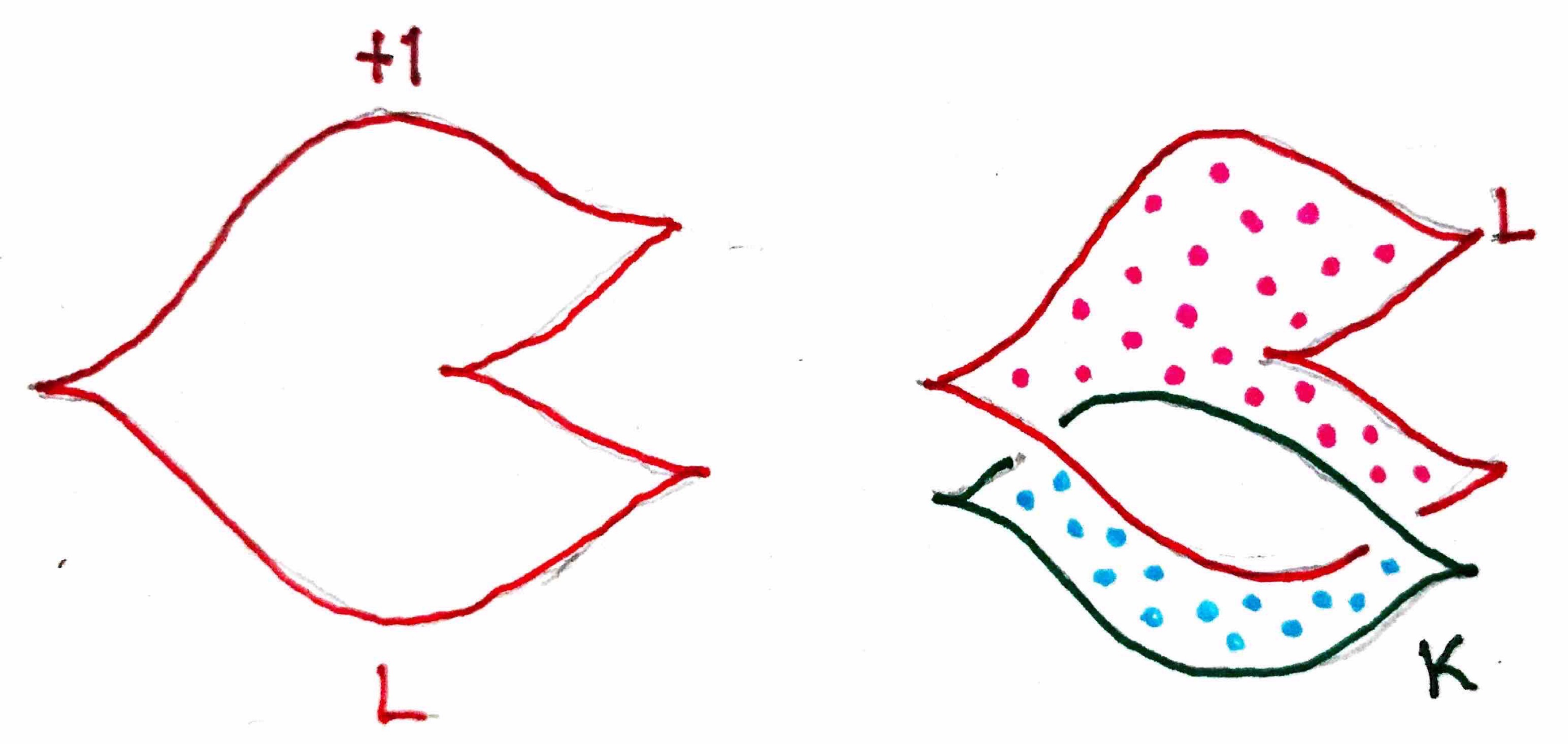
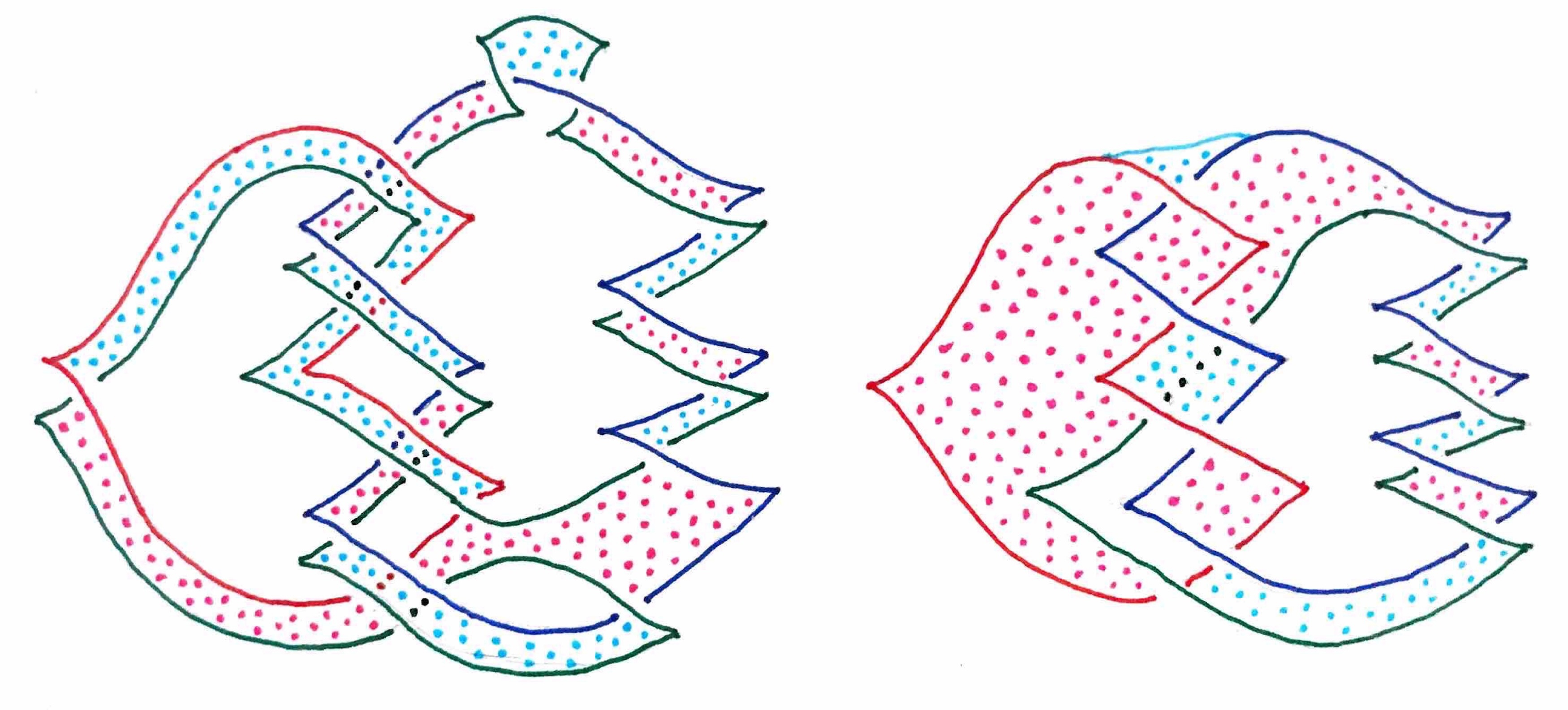
Above, $L$ and $K$ cobound an annulus $\Sigma$ [shown in polka-dots] so that the surface framing of $K$ by $\Sigma$ matches the contact framing, and the surface framing of $L$ by $\Sigma$ matches the surgery framing. Consequently, when surgery is perfomed on $L$, the annulus can be capped off by a disk at one end to obtain an overtwisted disk bounded by $K$.

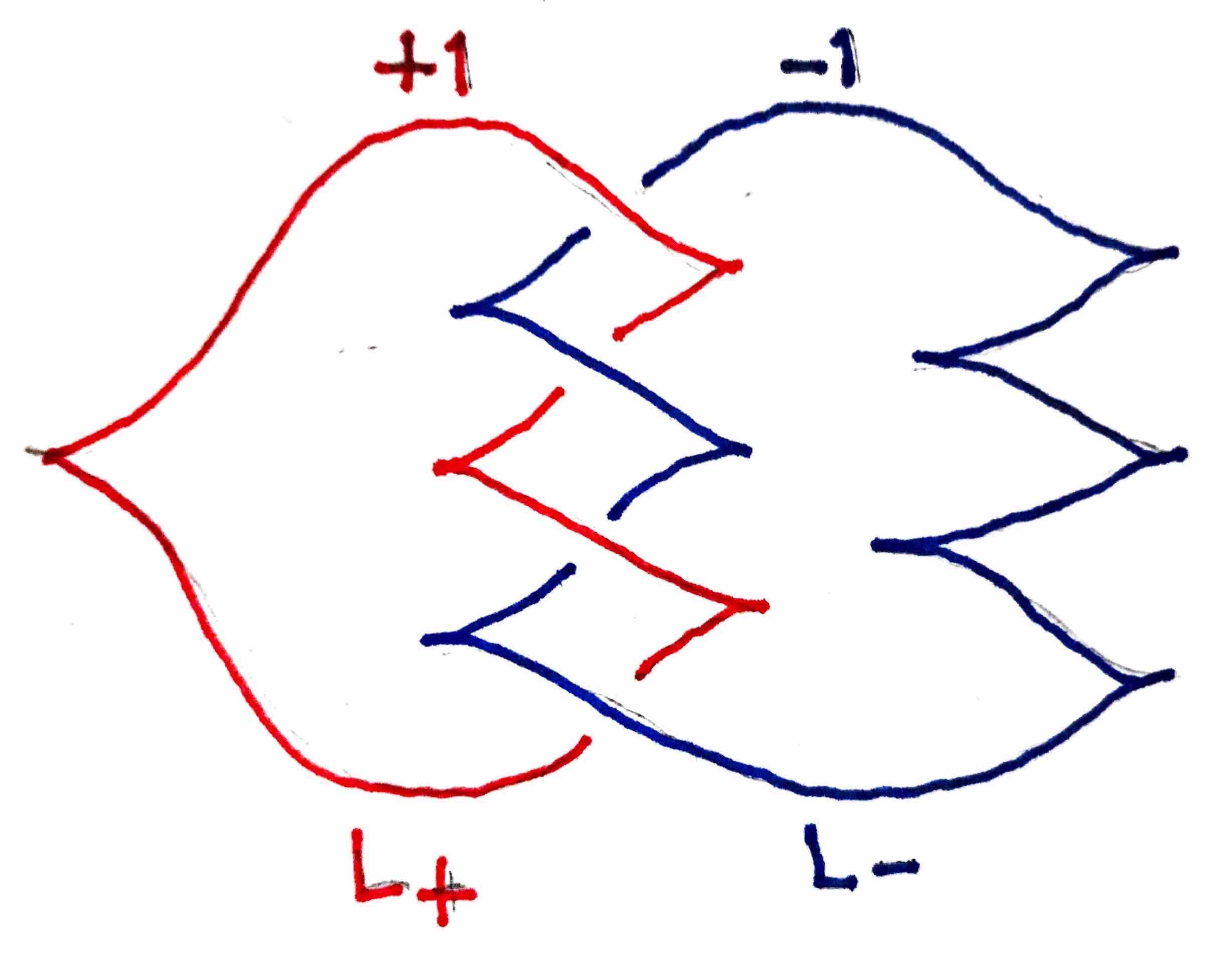
In the first diagram, I think the goal is to find an annulus or pair of pants $\Sigma$ bounded by $L_+$ and/or $L_-$ together with a third Legendrian knot $K$ with the same properties. We can't hope to find an annulus bounded by $L_{-}$ and $K$ with these properties, since the contact structure obtained by performing surgery on just $L_{-}$ is tight [in fact, Stein-fillable]. I've tried for quite some time but have been unsuccessful. Below are some failed attempts where the surface framings on $L_{\pm}$ are correct, but the surface framing on $K$ has one more $+1$ twist than the contact framing.

Answer 1

I apologize for posting an answer to my own question so soon. I was shown an answer recently by a post-doc at my university. My approach above could not have found this solution. The Legendrian knot $K$ in the diagram below bounds an overtwisted disk:

Working smoothly [with Seifert framings], we see that after blowing down $L_+$, the knots $K$ and $L_{-}$ bound an annulus which will become an overtwisted disk after surgery is performed on $L_{-}$:

I've convinced myself that in the initial diagram, this disk must be represented by a 4-times punctured sphere $\Sigma$ bounded by $L_{-}$, $K$, and two longitudes $L_+',L_+''$ of $L_+$ given by the surgery framing on $L_+$. The surface $\Sigma$ satisfies the following conditions: (i) the surface framing of $L_-$ by $\Sigma$ matches the surgery framing, (ii) the surface framing of $K$ by $\Sigma$ matches the contact framing, (iii) $\Sigma$ does not intersect $L_+$. However I can't quite picture what $\Sigma$ looks like.